A Temperature-Jump Optical Trap for Single-Molecule Manipulation - PubMed Skip to main page content
U.S. flag

An official website of the United States government

Dot gov

The .gov means it’s official.
Federal government websites often end in .gov or .mil. Before sharing sensitive information, make sure you’re on a federal government site.

Https

The site is secure.
The https:// ensures that you are connecting to the official website and that any information you provide is encrypted and transmitted securely.

Access keys NCBI Homepage MyNCBI Homepage Main Content Main Navigation
. 2015 Jun 16;108(12):2854-64.
doi: 10.1016/j.bpj.2015.05.017.

A Temperature-Jump Optical Trap for Single-Molecule Manipulation

Sara de Lorenzo  1 , Marco Ribezzi-Crivellari  2 , J Ricardo Arias-Gonzalez  3 , Steven B Smith  4 , Felix Ritort  5
Affiliations

A Temperature-Jump Optical Trap for Single-Molecule Manipulation

Sara de Lorenzo et al. Biophys J. .

Abstract

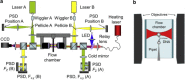
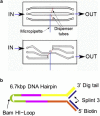
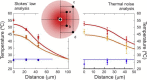
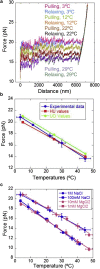
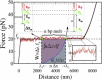
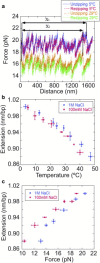
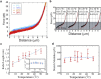
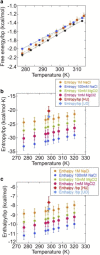
To our knowledge, we have developed a novel temperature-jump optical tweezers setup that changes the temperature locally and rapidly. It uses a heating laser with a wavelength that is highly absorbed by water so it can cover a broad range of temperatures. This instrument can record several force-distance curves for one individual molecule at various temperatures with good thermal and mechanical stability. Our design has features to reduce convection and baseline shifts, which have troubled previous heating-laser instruments. As proof of accuracy, we used the instrument to carry out DNA unzipping experiments in which we derived the average basepair free energy, entropy, and enthalpy of formation of the DNA duplex in a range of temperatures between 5°C and 50°C. We also used the instrument to characterize the temperature-dependent elasticity of single-stranded DNA (ssDNA), where we find a significant condensation plateau at low force and low temperature. Oddly, the persistence length of ssDNA measured at high force seems to increase with temperature, contrary to simple entropic models.

PubMed Disclaimer

Figures

Figure 1
Figure 1
Schematic of the setup. (a) Counterpropagating optical trap with a light-momentum force sensor modified to include a temperature controller. The heating beam passes through a 45° dichroic long-pass filter (Edmund Optics 69-878), which acts as a cold mirror, thus reflecting the blue LED light, which illuminates the experiment, until a CCD camera and allowing the heating wavelength to pass through. (b) Single-molecule experiment. The DNA hairpin under study is attached between two polystyrene beads: one captured in the optical trap and the other held by air suction on the tip of a micropipette (see text for details). To see this figure in color, go online.
Figure 2
Figure 2
Chamber channels and DNA hairpin designs. (a) Scheme of the old (upper panel) and new (lower panel) microfluidics chamber designed to prevent convection. The new design employs two coverglasses (24 × 60 mm, #2; VWR 48382-139) and one Nescolfilm layer (instead of two as in the old design). With this design, the microchamber thickness is reduced from 180 to 110 μm. The channel design was modified to avoid obstruction of the lower channel by the micropipette (∼80 μm in diameter). The central channel holds the micropipette in the dome (this cavity typically is 2 mm high and 1.8 mm wide). The direction of the flow, which is stopped during an experiment, is marked by IN and OUT labels. The top and bottom channels, connected to the main channel through dispenser tubes, are used to flow different types of beads and to control the concentration and flow speed at their entrance to the dome of the central channel. (b) Design of the 6.8 kb sequence. Details are provided in the Supporting Material. To see this figure in color, go online.
Figure 3
Figure 3
Temperature spatial profile of the heating laser beam. Measurements were taken at five different positions within the heating beam spot (see inset). (a–d) The distances from (a) and (b), and from (c) and (d) to the center of the screen are ∼23 μm and ∼42 μm, respectively. Red dots show the measured temperature at 5.7 mW inside the fluidics chamber (full heating laser power), and orange dots show the measured temperature at 4 mW. Blue dots show the measured temperature with the heating laser off. This measured temperature (∼27°C) is taken as ambient for the 1D heat-flow model (Supporting Material), whose predictions are the red and orange continuous curves for 5.7 and 4 mW, respectively. Left graph: Stokes’ law analysis; right graph: thermal noise analysis. To see this figure in color, go online.
Figure 4
Figure 4
Unzipping experiments at different temperatures. (a) Force-extension curves (FECs) measured at 1 M NaCl + TE, pH 7.5. Here the cold measurements have been aligned to the hot segments, where the position-sensitive photodetectors operate at room temperature, by matching the unzipping measurements at 25°C. See Supporting Material. Unzipping and rezipping traces are almost identical, showing that the experiments were carried out under quasistatic conditions. (b) Mean unzipping/rezipping force versus temperature. Experimental data points, each averaged over four molecules at 1 M NaCl TE buffer, pH 7.5, are shown in combination with two theoretical predictions based on experimental data from (10) (HU) or SantaLucia (4) (UO; see also Table S2). (c) Mean unzipping/rezipping force versus temperature in different ionic conditions. Experimental data represent the average over eight molecules. These results remarkably follow the rule regarding a 1:100 ratio of monovalent to divalent salt, similarly to what has been observed for RNA (33). To see this figure in color, go online.
Figure 5
Figure 5
Calculation of mean basepair free energies from unzipping measurements. The configuration of the experimental setup at two different distances (λ1, λ2) is shown above the FDC. The sawtooth pattern (inset) is approximated by a straight line corresponding to the mean unzipping force (fp). The force equals fp at λ1 and λ2, so the extension of trap and molecular handles (xT, xh) stays constant. Different distances λ correspond then to different ssDNA extensions. The reversible work necessary to drive the system reversibly from λ1 to λ2 corresponds to the area in the dashed rectangle. Along this transition, n basepairs are disrupted, and the corresponding free-energy change involves both the basepairing free energy and the free energy due to stretching 2n bases of ssDNA. The basepair free energy can be recovered once the elastic contribution arising from stretching the ssDNA is subtracted from W (as detailed in the main text). To see this figure in color, go online.
Figure 6
Figure 6
Dependence of the molecular extension of ssDNA on temperature and force. (a) Unzipping/rezipping traces for the same molecule at 5°C and 29°C. By measuring the change in distance between different peaks along an FDC (horizontal black arrows), we determine the molecular extension of the hairpin as a function of the temperature and force. (b) Extension/basepair versus temperature. Each dot represents the average over four molecules in either 1-M NaCl or 100-mM NaCl buffer solution. The force was not held constant; rather, the extensions were taken at the equilibrium zipping force, which varied with temperature, as shown in (a). Although the distance between basepairs does change with T, it does not appreciably change when the salt concentration is varied. (c) Extension/base versus unzipping force. Results were averaged over four molecules in either 1-M NaCl or 100-mM NaCl buffer solution. The distance between bases changes with force in both conditions. To see this figure in color, go online.
Figure 7
Figure 7
Temperature dependence of the single-stranded elastic response. (a) Cycles of pulling and relaxing curves of ssDNA at four different temperatures (6°C, 16°C, 22°C, and 26°C) and with 1 M NaCl, pH 7.5. The ssDNA was formed by using the oligo method (34). (b) The theoretical fit was obtained by using the ext-FJC model for forces in the range of 13–40 pN outside the dark gray region (labeled as no fitting in the legend). The experimental curves were taken at 1 M NaCl, pH 7.5. (c) Kuhn length (LK) values for ssDNA at different temperatures and 1 M NaCl as a function of the temperature. Inset: Kuhn length scaled to the thermal energy level, kBT. (d) Stretching modulus versus temperature for ssDNA measured at 1 M NaCl. In both panels, the dots represent the average at each temperature over six experiments, each with a different molecule. To see this figure in color, go online.
Figure 8
Figure 8
Average thermodynamic potentials versus temperature derived from DNA unzipping experiments. (a) Basepair free energy and comparison with UO (4) and HU (10) predictions at 1 M NaCl, pH 7.0. The orange squares show the experimental results calculated by fitting the elastic parameters as described in Supporting Material. The gray (blue) dots are average UO (HU) values for the free energy/basepair. (b) Basepair entropy as a function of temperature under different salt conditions. (c) Basepair enthalpy under different salt conditions. For comparison with the theoretical predictions, we also show the average of the entropies/enthalpies of the 16 NN motifs under standard conditions (1 M NaCl, 298 K) for the UO (blue diamond) and HU (red diamond) predictions. To see this figure in color, go online.
See this image and copyright information in PMC

Similar articles

See all similar articles

Cited by

See all "Cited by" articles

References

    1. Arias-Gonzalez J.R. Optical tweezers to study viruses. Subcell. Biochem. 2013;68:273–304. - PubMed
    1. Arias-Gonzalez J.R. Single-molecule portrait of DNA and RNA double helices. Integr Biol (Camb) 2014;6:904–925. - PubMed
    1. Hormeño S., Arias-Gonzalez J.R. Exploring mechanochemical processes in the cell with optical tweezers. Biol. Cell. 2006;98:679–695. - PubMed
    1. SantaLucia J., Jr. A unified view of polymer, dumbbell, and oligonucleotide DNA nearest-neighbor thermodynamics. Proc. Natl. Acad. Sci. USA. 1998;95:1460–1465. - PMC - PubMed
    1. Aboul-ela F., Koh D., Martin F.H. Base-base mismatches. Thermodynamics of double helix formation for dCA3XA3G + dCT3YT3G (X, Y = A,C,G,T) Nucleic Acids Res. 1985;13:4811–4824. - PMC - PubMed

Publication types

Substances