Mathematical modeling of lactate metabolism with applications to sports | Automation and Remote Control
Skip to main content
Springer Nature Link
Log in

Mathematical modeling of lactate metabolism with applications to sports

  • Control in Social Economic Systems, Medicine, and Biology
  • Published:
Automation and Remote Control Aims and scope Submit manuscript

Abstract

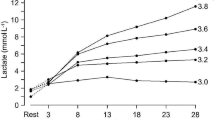
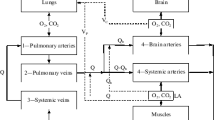
Based on a mathematical model of the blood circulatory system, we construct a mathematical model for lactate metabolism in a human body. We pose the identification problem for lactate metabolism parameters by measurements. We develop the method, algorithm, and software for solving this identification problem. We also consider practical applications in sports medicine and the training process, in particular in our studies of the anaerobic threshold phenomenon and propose new methods for estimating the individual anaerobic threshold and maximal oxygen consumption for athletes.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Shumakov, V.I., Novosel’tsev, V.N., Shtengol’d, E.Sh., and Sakharov, M.P., Modelirovanie fiziologicheskikh sistem organizma (Modeling Physiological Systems of the Human Body), Moscow: Meditsina, 1971.

    Google Scholar 

  2. Myakinchenko, E.B. and Seluyanov, V.N., Razvitie lokal’noi myshechnoi vynoslivosti v tsiklicheskikh vidakh sporta (Developing Local Muscular Endurance in Cyclical Sports), Moscow: TVT Divizion, 2009.

    MATH  Google Scholar 

  3. Janssen, P., Lactate Threshold Training, Champaign: Human Kinetics, 2001.

    Google Scholar 

  4. Proshin, A.P. and Solodyannikov, Yu.V., Identification of the Parameters of Blood Circulation System, Autom. Remote Control, 2010, vol. 71, no. 8, pp. 1629–1648.

    Article  MathSciNet  MATH  Google Scholar 

  5. Ostapenko, T.I., Proshin, A.P., and Solodyannikov, Yu.V., Research on Blood Circulation System Identifiability, Autom. Remote Control, 2007, vol. 68, no. 7, pp. 1239–1255.

    Article  MathSciNet  MATH  Google Scholar 

  6. Proshin, A.P. and Solodyannikov, Yu.V., Mathematical Modeling of Blood Circulation System and Its Practical Application, Autom. Remote Control, 2006, vol. 67, no. 2, pp. 329–341.

    Article  MathSciNet  MATH  Google Scholar 

  7. Solodyannikov, Yu.V., Elementy matematicheskogo modelirovaniya i identifikatsiya sistemy krovoobrashcheniya (Topics in Mathematical Modeling and Identification of the Blood Circulatory System), Samara: Samar. Univ., 1994.

    Google Scholar 

  8. Geoffrey, B.W., James, H.B., and Brian, J.E., A General Model for the Origin of Allometric Scaling Laws in Biology, Science, 1997, no. 276, pp. 122–126.

    Google Scholar 

  9. Kompleks apparatno-programmnyi neinvazivnogo issledovaniya tsentral’noi gemodinamiki metodom ob”emnoi kompressionnoi ostsillometrii “KAP TsG osm-Globus.” Instruktsiya po primeneniyu (A Hardware-Software Facility for Non-Invasive Studies of Central Hemodynamics with Volume Compression Oscillometry), Belgorod: OOO “Globus,” 2004.

  10. Severinghaus, J.W., Simple, Accurate Equations for Human Blood O2 Dissociation Computations, J. Appl. Physiol., 1979, no. 46, pp. 599–602.

    Google Scholar 

  11. Ellis, R.K., Determination of PO2 from Saturation, J. Appl. Physiol., 1989, no. 67, p. 902.

    Google Scholar 

  12. Rastrigin, L.A., Adaptatsiya slozhnykh sistem (Adaptation in Complex Systems), Riga: Zinatne, 1981.

    MATH  Google Scholar 

  13. Proshin, A.P. and Solodyannikov, Yu.V., Modelirovanie i identifikatsiya sistemy krovoobrashcheniya (Modeling and Identification of the Blood Circulatory System), Byull. Izobret., no. 2005611059, 2005.

    Google Scholar 

  14. Wasserman, K.Y. and McIlroy, M.B., Detecting the Threshold of Anaerobic Metabolism in Cardiac Patient during Exercise, Am. J. Cardiol., 1964, no. 14(3), pp. 844–852.

    Google Scholar 

  15. Holtmann, W.F., Zur Frange der Dauerleistungsfahigkeit, Fortschr. Med., 1961, no. 7(4), pp. 443–453.

    Google Scholar 

  16. Conconi, F., Ferrari, M., Ziglio, P.G., et al., Determination of the Anaerobic Threshold by a Non-Invasive Field Test in Runners, J. Appl. Physiol., 1982, no. 52(4), pp. 869–873.

    Google Scholar 

  17. Solov’ev, V.B., Gengin, M.T., Skudnov, V.M., and Petrushova, O.P., Acid-Base Blood Parameters for Sportsmen of Various Qualification Groups in the Norm and under Physical Exercise, Ros. Fiziol. Zhurn. im. I.M. Sechenova, 2010, vol. 96, no. 5, pp. 539–544.

    Google Scholar 

  18. Seluyanov, V.N., Sarsaniya, S.K., Stukalov, B.A., and Slutskii, L.V., Controlling Physical Preparedness in Sporting Adaptology, Teor. Praktika Fiz. Kul’tury, 2008, no. 5, pp. 36–38; 55–56.

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. SJC “Samara-Dialog,”, Samara, Russia

    A. P. Proshin & Yu. V. Solodyannikov

Authors
  1. A. P. Proshin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yu. V. Solodyannikov
    View author publications

    You can also search for this author in PubMed Google Scholar

Additional information

Original Russian Text © A.P. Proshin, Yu.V. Solodyannikov, 2013, published in Avtomatika i Telemekhanika, 2013, No. 6, pp. 133–152.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Proshin, A.P., Solodyannikov, Y.V. Mathematical modeling of lactate metabolism with applications to sports. Autom Remote Control 74, 1004–1019 (2013). https://doi.org/10.1134/S0005117913060106

Download citation

  • Received:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1134/S0005117913060106

Keywords

Search

Navigation