Abstract
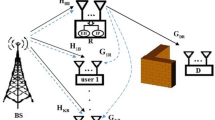
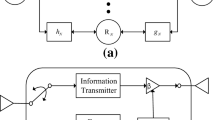
Self-energy recycling cooperative communication, in which the relay nodes simultaneously harvest energy from the source and their own transmitted signal, has been demonstrated to significantly increase the total harvested energy. In this paper, we present an optimization framework for the resource allocation and relay selection with the objective of maximizing the sum-throughput in afull-duplex multi-relay multi-user multiple-input-multiple-output network by incorporating self-energy recycling at the relays, for the first time. The formulated problem is a mixed integer non-linear programming problem, which is difficult to solve for the global optimal solution in polynomial-time. As a solution strategy, we decompose the optimization problem into two sub-problems: resource allocation problem and relay selection problem. First, we solve the resource allocation problem for a predetermined relay selection by using Taylor series approximation and convex optimization techniques. For the relay selection problem, we propose a polynomial-time sub-optimal algorithm based on the idea of iteratively selecting the relay offering the maximum throughput at each time. Through simulations, we demonstrate that the proposed dynamic time FD-MIMO system increases the throughput by 30% as compared to the conventional static time protocol system, and by a factor of 2.4 over the system without self-energy recycling.






Similar content being viewed by others
References
Lu, X., Wang, P., Niyato, D., Kim, D. I., & Han, Z. (2015). Wireless networks with RF energy harvesting: A contemporary survey. IEEE Communications Surveys Tutorials, 17(2), 757–789. https://doi.org/10.1109/COMST.2014.2368999
Ku, M.-L., Li, W., Chen, Y., & Ray Liu, K. J. (2016). Advances in energy harvesting communications: Past, present, and future challenges. IEEE Communications Surveys Tutorials, 18(2), 1384–1412. https://doi.org/10.1109/COMST.2015.2497324
Ponnimbaduge Perera, T. D., Jayakody, D. N. K., Sharma, S. K., Chatzinotas, S., & Li, J. (2018). Simultaneous wireless information and power transfer (SWIPT): Recent advances and future challenges. IEEE Communications Surveys Tutorials, 20(1), 264–302. https://doi.org/10.1109/COMST.2017.2783901
Kang, X., Ho, C. K., & Sun, S. (2015). Full-duplex wireless-powered communication network with energy causality. IEEE Transactions on Wireless Communications, 14(10), 5539–5551. https://doi.org/10.1109/TWC.2015.2439673
Hadzi-Velkov, Z., Nikoloska, I., Chingoska, H., & Zlatanov, N. (2017). Opportunistic scheduling in wireless powered communication networks. IEEE Transactions on Wireless Communications, 16(6), 4106–4119. https://doi.org/10.1109/TWC.2017.2691785
Iqbal, M. S., Sadi, Y., & Coleri, S. (2020). Minimum length scheduling for full duplex time-critical wireless powered communication networks. IEEE Transactions on Wireless Communications, 19(9), 5993–6006. https://doi.org/10.1109/TWC.2020.2999130
Iqbal, M. S., Sadi, Y., & Coleri, S. (2022). Minimum length scheduling for discrete-rate full-duplex wireless powered communication networks. IEEE Transactions on Wireless Communications, 21(1), 135–148. https://doi.org/10.1109/TWC.2021.3094138
Iqbal, M. S., Sadi, Y., & Coleri, S. (2021). Minimum length scheduling for multi-cell full duplex wireless powered communication networks. Sensors. https://doi.org/10.3390/s21196599
Kazmi, S. A. A., Iqbal, M. S., & Coleri, S. (2021). Relay selection and throughput maximization for full duplex wireless powered cooperative communication networks. In: 2021 IEEE 32nd annual international symposium on personal, indoor and mobile radio communications (PIMRC), pp. 360–365. https://doi.org/10.1109/PIMRC50174.2021.9569288
Iqbal, M. S., Sadi, Y., & Coleri, S. (2020). Throughput maximization for full duplex wireless powered communication networks. In: ICC 2020 - 2020 IEEE international conference on communications (ICC), pp. 1–6. https://doi.org/10.1109/ICC40277.2020.9148904
Laneman, J. N., Tse, D. N. C., & Wornell, G. W. (2004). Cooperative diversity in wireless networks: Efficient protocols and outage behavior. IEEE Transactions on Information Theory, 50(12), 3062–3080.
Ibrahim, A. S., Sadek, A. K., Su, W., & Liu, K. J. R. (2008). Cooperative communications with relay-selection: When to cooperate and whom to cooperate with? IEEE Transactions on Wireless Communications, 7(7), 2814–2827.
Nosratinia, A., Hunter, T. E., & Hedayat, A. (2004). Cooperative communication in wireless networks. IEEE Communications Magazine, 42(10), 74–80.
Pabst, R., Walke, B. H., Schultz, D. C., Herhold, P., Yanikomeroglu, H., Mukherjee, S., Viswanathan, H., Lott, M., Zirwas, W., Dohler, M., Aghvami, H., Falconer, D. D., & Fettweis, G. P. Relay-based deployment concepts for wireless and mobile broadband radio. IEEE Communications Magazine, 42(9), 80–89. https://doi.org/10.1109/MCOM.2004.1336724
Sendonaris, A., Erkip, E., & Aazhang, B. (2003). User cooperation diversity. Part I. System description. IEEE Transactions on Communications, 51(11), 1927–1938. https://doi.org/10.1109/TCOMM.2003.818096
Mishra, D., De, S., & Krishnaswamy, D. (2017). Dilemma at RF energy harvesting relay: Downlink energy relaying or uplink information transfer? IEEE Transactions on Wireless Communications, 16(8), 4939–4955. https://doi.org/10.1109/TWC.2017.2704084
Nasir, A. A., Zhou, X., Durrani, S., & Kennedy, R. A. (2013). Relaying protocols for wireless energy harvesting and information processing. IEEE Transactions on Wireless Communications, 12(7), 3622–3636. https://doi.org/10.1109/TWC.2013.062413.122042
Nasir, A. A., Zhou, X., Durrani, S., & Kennedy, R. A. (2014). Throughput and ergodic capacity of wireless energy harvesting based DF relaying network. In: 2014 IEEE international conference on communications (ICC), pp. 4066–4071. https://doi.org/10.1109/ICC.2014.6883957
Chen, Y. (2016). Energy-harvesting AF relaying in the presence of interference and Nakagami-\(m\) fading. IEEE Transactions on Wireless Communications, 15(2), 1008–1017. https://doi.org/10.1109/TWC.2015.2481393
Ding, Z., Krikidis, I., Sharif, B., & Poor, H. V. (2014). Wireless information and power transfer in cooperative networks with spatially random relays. IEEE Transactions on Wireless Communications, 13(8), 4440–4453. https://doi.org/10.1109/TWC.2014.2314114
Atapattu, S., Jiang, H., Evans, J., & Tellambura, C. (2015). Time-switching energy harvesting in relay networks. In: 2015 IEEE International conference on communications (ICC), pp. 5416–5421. https://doi.org/10.1109/ICC.2015.7249185
Amarasuriya, G., Larsson, E. G., & Poor, H. V. (2016). Wireless Information and power transfer in multiway massive MIMO relay networks. IEEE Transactions on Wireless Communications, 15(6), 3837–3855. https://doi.org/10.1109/TWC.2016.2530067
Lee, H., Song, C., Choi, S.-H., & Lee, I. (2017). Outage probability analysis and power splitter designs for SWIPT relaying systems with direct link. IEEE Communications Letters, 21(3), 648–651. https://doi.org/10.1109/LCOMM.2016.2627055
Tao, R., Salem, A., & Hamdi, K. A. (2016). Adaptive relaying protocol for wireless power transfer and information processing. IEEE Communications Letters, 20(10), 2027–2030. https://doi.org/10.1109/LCOMM.2016.2593877
Gupta, A., Singh, K., & Sellathurai, M. (2019). Time-switching EH-based joint relay selection and resource allocation algorithms for multi-user multi-carrier AF relay networks. IEEE Transactions on Green Communications and Networking, 3(2), 505–522. https://doi.org/10.1109/TGCN.2019.2906616
Boshkovska, E., Ng, D. W. K., Zlatanov, N., & Schober, R. (2015). Practical non-linear energy harvesting model and resource allocation for SWIPT systems. IEEE Communications Letters, 19(12), 2082–2085. https://doi.org/10.1109/LCOMM.2015.2478460
Gautam, S., Lagunas, E., Chatzinotas, S., & Ottersten, B. (2019). Relay selection and resource allocation for SWIPT in multi-user OFDMA systems. IEEE Transactions on Wireless Communications, 18(5), 2493–2508. https://doi.org/10.1109/TWC.2019.2904273
Dong, Y., Hossain, M. J., & Cheng, J. (2016). Performance of wireless powered amplify and forward relaying over Nakagami-\(m\) fading channels with nonlinear energy harvester. IEEE Communications Letters, 20(4), 672–675. https://doi.org/10.1109/LCOMM.2016.2528260
Zhang, J., & Pan, G. (2016). Outage analysis of wireless-powered relaying MIMO systems with non-linear energy harvesters and imperfect CSI. IEEE Access, 4, 7046–7053. https://doi.org/10.1109/ACCESS.2016.2617893
Wang, S., Xia, M., Huang, K., & Wu, Y.-C. (2017). Wirelessly powered two-way communication with nonlinear energy harvesting model: Rate regions under fixed and mobile relay. IEEE Transactions on Wireless Communications, 16(12), 8190–8204. https://doi.org/10.1109/TWC.2017.2758767
Zhong, C., Suraweera, H. A., Zheng, G., Krikidis, I., & Zhang, Z. (2014). Wireless information and power transfer with full duplex relaying. IEEE Transactions on Communications, 62(10), 3447–3461. https://doi.org/10.1109/TCOMM.2014.2357423
Mohammadi, M., Chalise, B. K., Suraweera, H. A., Zhong, C., Zheng, G., & Krikidis, I. (2016). Throughput analysis and optimization of wireless-powered multiple antenna full-duplex relay systems. IEEE Transactions on Communications, 64(4), 1769–1785. https://doi.org/10.1109/TCOMM.2016.2527785
Liu, H., Kim, K. J., Kwak, K. S., & Vincent Poor, H. (2016). Power splitting-based SWIPT with decode-and-forward full-duplex relaying. IEEE Transactions on Wireless Communications, 15(11), 7561–7577. https://doi.org/10.1109/TWC.2016.2604801
Liu, H., Kim, K. J., Kwak, K. S., & Poor, H. V. (2017). QoS-constrained relay control for full-duplex relaying with SWIPT. IEEE Transactions on Wireless Communications, 16(5), 2936–2949. https://doi.org/10.1109/TWC.2017.2672551
Wang, D., Zhang, R., Cheng, X., Yang, L., & Chen, C. (2017). Relay selection in full-duplex energy-harvesting two-way relay networks. IEEE Transactions on Green Communications and Networking, 1(2), 182–191. https://doi.org/10.1109/TGCN.2017.2686325
Chen, G., Xiao, P., Kelly, J. R., Li, B., & Tafazolli, R. (2017). Full-duplex wireless-powered relay in two way cooperative networks. IEEE Access, 5, 1548–1558. https://doi.org/10.1109/ACCESS.2017.2661378
Guo, J., Zhang, S., Zhao, N., & Wang, X. (2020). Performance of SWIPT for full-duplex relay system with co-channel interference. IEEE Transactions on Vehicular Technology, 69(2), 2311–2315. https://doi.org/10.1109/TVT.2019.2958626
Nauryzbayev, G., Abdallah, M., & Rabie, K. M. (2018). Outage probability of the EH-Based Full-Duplex AF and DF Relaying Systems in \(\alpha -\mu\) Environment. In: 2018 IEEE 88th vehicular technology conference (VTC-Fall), pp. 1–6. https://doi.org/10.1109/VTCFall.2018.8690985
Liu, Y., Wen, Z., Beaulieu, N. C., Liu, D., & Liu, X. (2020). Power allocation for SWIPT in Full-duplex AF relay interference channels using game theory. IEEE Communications Letters, 24(3), 608–611. https://doi.org/10.1109/LCOMM.2019.2963640
Liu, K.-H., & Kung, T.-L. (2017). Performance improvement for RF energy-harvesting relays via relay selection. IEEE Transactions on Vehicular Technology, 66(9), 8482–8494. https://doi.org/10.1109/TVT.2017.2692804
Xie, X., Chen, J., & Fu, Y. (2018). Outage performance and QoS optimization in full-duplex system with non-linear energy harvesting model. IEEE Access, 6, 44281–44290. https://doi.org/10.1109/ACCESS.2018.2861383
Kazmi, S. A. A., & Coleri, S. (2020). Optimization of full-duplex relaying system with non-linear energy harvester. IEEE Access, 8, 201566–201576. https://doi.org/10.1109/ACCESS.2020.3035005
Nguyen, B. C., Hoang, T. M., Dung, L. T., & Kim, T. (2021). Impacts of nonlinear energy harvesting and residual self-interference on the performance of full-duplex decode-and-forward relay system. IEEE Access, 9, 42333–42344. https://doi.org/10.1109/ACCESS.2021.3065500
Hwang, D., Hwang, K. C., Kim, D. I., & Lee, T. (2017). Self-energy recycling for RF powered multi-antenna relay channels. IEEE Transactions on Wireless Communications, 16(2), 812–824.
Zeng, Y., & Zhang, R. (2015). Full-duplex wireless-powered relay with self-energy recycling. IEEE Wireless Communications Letters, 4(2), 201–204.
Nasir, A. A., Tuan, H. D., Duong, T. Q., & Poor, H. V. (2019) Full-duplex MIMO-OFDM communication with self-energy recycling. arXiv:1903.09931. [Online]. Available: arXiv:1903.09931
Nasir, A. A., Tuan, H. D., Duong, T. Q., & Poor, H. V. (2020). MIMO-OFDM-based wireless-powered relaying communication with an energy recycling interface. IEEE Transactions on Communications, 68(2), 811–824.
Wei, Z., Sun, S., Zhu, X., In Kim, D., & Ng, D. W. K. (2019). Resource allocation for wireless-powered full-duplex relaying systems with nonlinear energy harvesting efficiency. IEEE Transactions on Vehicular Technology, 68(12), 12079–12093.
Ng, D. W. K., Lo, E. S., & Schober, R. (2012). Dynamic resource allocation in mimo-ofdma systems with full-duplex and hybrid relaying. IEEE Transactions on Communications, 60(5), 1291–1304. https://doi.org/10.1109/TCOMM.2012.031712.110233
Zeng, Y., & Zhang, R. (2015). Optimized training design for wireless energy transfer. IEEE Transactions on Communications, 63(2), 536–550. https://doi.org/10.1109/TCOMM.2014.2385077
Ng, D. W. K., Breiling, M., Rohde, C., Burkhardt, F., & Schober, R. (2016). Energy-efficient 5G outdoor-to-indoor communication: SUDAS over licensed and unlicensed spectrum. IEEE Transactions on Wireless Communications, 15(5), 3170–3186. https://doi.org/10.1109/TWC.2016.2517626
Rong, Y., & Hua, Y. (2009). Optimality of diagonalization of multi-hop MIMO relays. IEEE Transactions on Wireless Communications, 8(12), 6068–6077. https://doi.org/10.1109/TWC.2009.12.090379
Floudas, C. A. (1995). Nonlinear and mixed-integer optimization: Fundamentals and applications. London, U.K.: Oxford Univ. Press.
Soysa, M., Suraweera, H. A., Tellambura, C., & Garg, H. K. (2012). Partial and opportunistic relay selection with outdated channel estimates. IEEE Transactions on Communications, 60(3), 840–850. https://doi.org/10.1109/TCOMM.2012.12.100671
Chalise, B. K., Vandendorpe, L., Zhang, Y. D., & Amin, M. G. (2012). Local CSI based selection beamforming for amplify-and-forward MIMO relay networks. IEEE Transactions on Signal Processing, 60(5), 2433–2446. https://doi.org/10.1109/TSP.2012.2184535
Funding
This work is supported by the Scientific and Technological Research Council of Turkey Grant \(\#\)117E241.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
Conflict of interest
All authors declare that they have no conflict of interest.
Ethical approval
This article does not contain any studies with human participants or animals performed by any of the authors.
Additional information
Publisher's Note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Appendix
Appendix
Proof
Taking the derivative of Lagrangian function L w.r.t \(\tau _1^{m_k,k}\) and \(\tau _2^{m_k,k}\) yields
We know that the optimization problem is maximized when
Therefore, the following equality holds
Substituting constraint (17d) into Eq. (30) gives the optimal solution of \(\tau _1^{m_k,k}\) and \(\tau _2^{m_k,k}\), as presented in Theorem 1.
Similarly, taking the derivative of L w.r.t \(p_{s,n}^{m_k,k}\) and \(p_{r,n}^{m_k,k}\) is given as
Putting the values of \(\tau _1^{m_k,k}\) and \(\tau _2^{m_k,k}\) from Eqs. (19) and (20) give
After some simple algebraic manipulations which are skipped for brevity, Eqs. (33) and (34) can be written as in Theorem 1. \(\square\)
Rights and permissions
Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.
About this article
Cite this article
Kazmi, S.A.A., Iqbal, M.S. & Coleri, S. Resource allocation for full-duplex MIMO relaying system with self-energy recycling. Wireless Netw 30, 781–797 (2024). https://doi.org/10.1007/s11276-023-03527-x
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11276-023-03527-x