Quantum video encryption based on bitplanes and improved Arnold scrambling | Quantum Information Processing Skip to main content

Advertisement

Springer Nature Link
Log in

Quantum video encryption based on bitplanes and improved Arnold scrambling

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

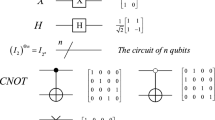
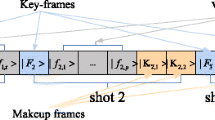
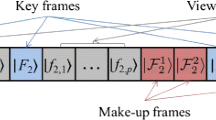
Abstract

Quantum video encryption is an essential method for ensuring video content security. This paper proposes a quantum video encryption method based on bitplanes and improved Arnold scrambling. Firstly, we design a quantum video representation based on bitplanes (BRQV) and an improved Arnold scrambling to entangle video frames. Then, we use 2D quantum wavelet transforms and an improved logic mapping for frequency-domain encryption. The encryption security analysis reveals that the post-encryption SSIM value is close to 0.015, with an information entropy reaching 7.9541. The circuit complexity analysis shows that the proposed method can realize the video encryption using \(16{n^3} + 272{n^2} - 88n\) quantum basic gates with the circuit width \(2n + \log _2 n + 5\). The best existing quantum video encryption method needs \(\mathrm{{O(}}{2^n}{n^3})\) quantum basic gates with the circuit width \(3n + 3\). Simulation results demonstrate that the proposed video encryption method exhibits low correlation coefficients and high efficiency.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24
Fig. 25
Fig. 26
Fig. 27

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Data Availability

The datasets generated during and/or analyzed during the current study are available from the corresponding author on reasonable request.

References

  1. Adesso, G., Bromley, T.R., Cianciaruso, M.: Measures and applications of quantum correlations. J. Phys. A Math. Theor. 49 (2016)

  2. Blais, A., Girvin, S.M., Oliver, W.D.: Quantum information processing and quantum optics with circuit quantum electrodynamics. Nat. Phys. 16, 247–256 (2020)

    Article  Google Scholar 

  3. Yan, F., Iliyasu, A.M., Venegas-Andraca, S.E.: A survey of quantum image representations. Quantum Inf. Process. 15, 1–35 (2016)

    Article  ADS  MathSciNet  Google Scholar 

  4. Song, S.Y., Wang, C.: Recent development in quantum communication. Chin. Sci. Bull. 57, 4694–4700 (2012)

    Article  Google Scholar 

  5. Wang, Z.B., Xu, M.Z., Zhang, Y.N.: Review of quantum image processing. Arch. Comput. Meth. Eng. 29, 737–761 (2022)

    Article  MathSciNet  Google Scholar 

  6. Venegas-Andraca, S.E., Ball, J.L.: Processing images in entangled quantum systems. Quantum Inf. Process. 9, 1–11 (2010)

    Article  MathSciNet  Google Scholar 

  7. Iliyasu, A.M., Le, P.Q., Dong, F.Y., Hirota, K.: Watermarking and authentication of quantum images based on restricted geometric transformations. Inf. Sci. 186, 126–149 (2012)

    Article  MathSciNet  Google Scholar 

  8. Liang, H.R., Tao, X.Y., Zhou, N.R.: Quantum image encryption based on generalized affine transform and logistic map. Quantum Inf. Process. 15, 2701–2724 (2016)

    Article  ADS  MathSciNet  Google Scholar 

  9. Li, H.S., Zhu, Q.X., Lan, S., Shen, C.Y., Zhou, R.G., Mo, J.: Image storage, retrieval, compression and segmentation in a quantum system. Quantum Inf. Process. 12, 2269–2290 (2013)

    Article  ADS  MathSciNet  Google Scholar 

  10. Jiang, N., Wu, W.Y., Wang, L.: The quantum realization of Arnold and Fibonacci image scrambling. Quantum Inf. Process. 13, 1223–1236 (2014)

    Article  ADS  MathSciNet  Google Scholar 

  11. Zhou, N.R., Chen, W.W., Yan, X.Y., Wang, Y.Q.: Bit-level quantum color image encryption scheme with quantum cross-exchange operation and hyper-chaotic system. Quantum Inf. Process. 17 (2018)

  12. Gong, L.H., He, X.T., Cheng, S., Hua, T.X., Zhou, N.R.: Quantum image encryption algorithm based on quantum image XOR operations. Int. J. Theor. Phys. 55, 3234–3250 (2016)

    Article  MathSciNet  Google Scholar 

  13. Song, X.H., Wang, H.Q., Venegas-Andraca, S.E., Abd El-Latif, A.A.: Quantum video encryption based on qubit-planes controlled-XOR operations and improved logistic map. Physica A. 537 (2020)

  14. Wu, W., Wang, Q.: Quantum image encryption based on baker map and 2D logistic map. Int. J. Theor. Phys. 61, 64 (2022)

    Article  MathSciNet  Google Scholar 

  15. Ma, H., Ma, Y., Zhang, W., et al.: Development of video encryption scheme based on quantum controlled dense coding using GHZ state for smart home scenario. Wirel. Pers. Commun. 123, 295–309 (2022)

    Article  Google Scholar 

  16. Ran, Q.W., Wang, L., Ma, J., Tan, L.Y., Yu, S.Y.: A quantum color image encryption scheme based on coupled hyper-chaotic Lorenz system with three impulse injections. Quantum Inf. Process. 17 (2018)

  17. Li, P.C., Zhao, Y.: A simple encryption algorithm for quantum color image. Int. J. Theor. Phys. 56, 1961–1982 (2017)

    Article  MathSciNet  Google Scholar 

  18. Le, P.Q., Dong, F., Hirota, K.: A flexible representation of quantum images for polynomial preparation, image compression, and processing operations. Quantum Inf. Process. 10, 63–84 (2011)

    Article  MathSciNet  Google Scholar 

  19. Zhang, Y., Lu, K., Gao, Y.H., Wang, M.: NEQR: a novel enhanced quantum representation of digital images. Quantum Inf. Process. 12, 2833–2860 (2013)

    Article  ADS  MathSciNet  Google Scholar 

  20. Sang, J.Z., Wang, S., Li, Q.: A novel quantum representation of color digital images. Quantum Inf. Process. 16 (2017)

  21. Li, H.S., Fan, P., Xia, H.Y., Peng, H., Song, S.: Quantum implementation circuits of quantum signal representation and type conversion. IEEE Trans. Circuits Syst. I Reg. Papers 66, 341–354 (2019)

    Article  Google Scholar 

  22. Li, H.S., Chen, X., Xia, H.Y., Liang, Y., Zhou, Z.S.: A quantum image representation based on bitplanes. IEEE Access 6, 62396–62404 (2018)

    Article  Google Scholar 

  23. Hu, W.B., Dong, Y.M.: Quantum color image encryption based on a novel 3D chaotic system. J. Appl. Phys. 131 (2022)

  24. Zhou, N.R., Hu, Y.Q., Gong, L.H., Li, G.Y.: Quantum image encryption scheme with iterative generalized Arnold transforms and quantum image cycle shift operations. Quantum Inf. Process. 16 (2017)

  25. Iliyasu, A.M., Le, P.Q., Dong, F.Y., Hirota, K.: A framework for representing and producing movies on quantum computers. Int. J. Quantum Inf. 9, 1459–1497 (2011)

    Article  Google Scholar 

  26. Yan, F., Iliyasu, A.M., Venegas-Andraca, S.E., et al.: Video encryption and decryption on quantum computers. Int. J. Theor. Phys. 54, 2893–2904 (2015)

    Article  MathSciNet  Google Scholar 

  27. Zhu, D., Zheng, J., Zhou, H., et al.: A hybrid encryption scheme for quantum secure video conferencing combined with blockchain. Mathematics 10(17), 3037 (2022)

    Article  Google Scholar 

  28. Li, H.S., Fan, P., Xia, H.Y., Peng, H.L., Long, G.L.: Efficient quantum arithmetic operation circuits for quantum image processing. Sci. China Phys. Mech. Astron. 63 (2020)

  29. Li, H.S., Fan, P., Xia, H.Y., Long, G.L.: The circuit design and optimization of quantum multiplier and divider. Sci. China Phys. Mech. Astron. 65 (2022)

  30. Jiang, N., Wang, L.: Analysis and improvement of the quantum Arnold image scrambling. Quantum Inf. Process. 13, 1545–1551 (2014)

    Article  ADS  MathSciNet  Google Scholar 

  31. Li, H.S., Fan, P., Peng, H.L., Song, S.X., Long, G.L.: Multilevel 2-D quantum wavelet transforms. IEEE Trans. Cybern. 52, 8467–8480 (2022)

    Article  Google Scholar 

  32. Abd El-Latif, A.A., Li, L., Wang, N., Han, Q., Niu, X.M.: A new approach to chaotic image encryption based on quantum chaotic system, exploiting color spaces. Signal Process. 93, 2986–3000 (2013)

    Article  Google Scholar 

  33. Pak, C., An, K., Jang, P., Kim, J., Kim, S.: A novel bit-level color image encryption using improved 1D chaotic map. Multimedia Tools Appl. 78, 12027–12042 (2019)

    Article  Google Scholar 

  34. Zhang, Y.: The unified image encryption algorithm based on chaos and cubic S-Box. Inf. Sci. 450, 361–377 (2018)

    Article  ADS  MathSciNet  Google Scholar 

  35. Abd-El-Atty, B.: Quaternion with quantum walks for designing a novel color image cryptosystem. J. Inf. Secur. Appl. 71, 103367 (2022)

    Google Scholar 

Download references

Funding

This work is supported by the Natural Science Foundation of Guangxi under Grant Nos. 2020GXNSFDA238023 and 2023JJA170043.

Author information

Authors and Affiliations

  1. College of Electronic and Information Engineering, Guangxi Normal University, Guilin, 541004, Guangxi, China

    Yuxing Wei, Hai-sheng Li, Kai Liu & Shantao Zhao

Authors
  1. Yuxing Wei
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Hai-sheng Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Kai Liu
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Shantao Zhao
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Hai-sheng Li.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Consent to participate

All authors consent to participate.

Consent for publication

All authors consent for publication.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wei, Y., Li, Hs., Liu, K. et al. Quantum video encryption based on bitplanes and improved Arnold scrambling. Quantum Inf Process 23, 67 (2024). https://doi.org/10.1007/s11128-024-04281-5

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11128-024-04281-5

Keywords

Search

Navigation