Abstract
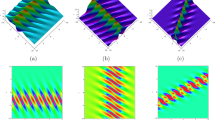
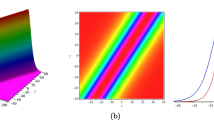
In this paper, based on the direct method proposed by Akira Nakamura, we present an efficient numerical scheme to calculate the N-periodic wave solutions to the Tzitzeica equation and the (2 + 1)-dimensional modified Bogoyavlenskii-Schiff (mBS) equation which can be transformed into a coupled bilinear system with some dependent variable transformation. By using this numerical scheme, we calculate their 2-periodic wave solutions and 3-periodic wave solutions as examples. We also show the asymptotic behaviors under a “small amplitude” limit of these quasi-periodic wave solutions numerically.








Similar content being viewed by others
References
Newell, A.C.: Solitons in Mathematics and Physics. SIAM, Philadelphia (1981)
Ablowitz, M.J., Clarkson, P.A.: Solitons, Nonlinear Evolution Equations and Inverse Scattering. Cambridge university press (1991)
Matveev, V.B., Salle, M.A.: Darboux Transformation and Solitons. Springer (1991)
Hirota, R.: The Direct Method in Soliton Theory, Cambridge University Press (2004)
Belokolos, E.D., Bobenko, A.I., Enol’skij, V.Z., Its, A.R., Matveev, V.B.: Algebro-Geometrical Approach to Nonlinear Integrable Equations. Springer (1994)
Yang, J.: Nonlinear Waves in Integrable and Nonintegrable Systems. SIAM, Philadelphia (2010)
Novikov, S.P.: A periodic problem for the Korteweg-de Vries equation. I. Funktsional Anal. i Prilozhen. 8, 54–66 (1974)
Dubrovin, B.A.: Periodic problems for the Korteweg-de Vries equation in the class of finite band potentials. Funct. Anal. Appl. 9, 215–223 (1975)
Dubrovin, B.A., Novikov, S.P.: Periodic and conditionally periodic analogues of the many-soliton solutions of the Kortweg-de Vries equation. Sov. Phys. JETP. 40, 1058 (1975)
Lax, P.D.: Periodic solutions of the KdV equation. Commun. Pure Appl. Math. 28, 141–188 (1975)
Its, A.R., Matveev, V.B.: The periodic Korteweg-de Vries equation. Funct. Anal. Appl. 9, 67 (1975)
McKean, H.P., van Moerbeke, P.: The spectrum of Hill’s equation. Invent. Math. 30, 217–274 (1975)
Ma, Y.C., Ablowitz, M.J.: The periodic cubic Schrödinger equation. Stud. Appl. Math. 65, 113–158 (1981)
Forest, M.G., McLaughlin, D.W.: Spectral theory for the periodic sine-Gordon equation: A concrete viewpoint. J. Math. Phys. 23, 1248 (1982)
Date, E., Tanaka, S.: Periodic multi-soliton solutions of Korteweg-de Vries equation and Toda lattice. Prog. Theor. Phys. Suppl. 59, 107–126 (1976)
Krichever, I.M.: Algebraic-geometric construction of the Zaharov-Sabat equations and their periodic solutions. Dokl. Akad. Nauk SSSR 227, 394–397 (1976)
Dubrovin, B.A.: Theta functions and nonlinear equations. Russ. Math. Surv. 36, 11–92 (1981)
Belokolos, E.D., Bobenko, A.I., Enol’skii, V.Z., Its, A.R., Matveev, V.B.: Algebro-Geometric Approach to Nonlinear Integrable Equations. Springer, Berlin (1994)
Cao, C.W.: Parametric representation of the finite-band solution of the Heisenberg equation. Phys. Lett. A. 184, 333–338 (1994)
Cao, C.W., Wu, Y.T., Geng, X.G.: On quasi-periodic solutions of the 2 + 1 dimensional Caudrey-Dodd-Gibbon-Kotera-Sawada equation. Phys. Lett. A. 256, 59–65 (1999)
Geng, X.G., Wu, L.H., He, G.L.: Quasi-periodic solutions of the Kaup-Kupershmidt hierarchy. J. Nonlinear Sci. 23, 527–555 (2013)
Geng, X.G., Wu, L.H., He, G.L.: Algebro-geometric constructions of the modified Boussinesq flows and quasi-periodic solutions. Physica D 240, 1262–1288 (2011)
Geng, X.G., Wu, L.H., He, G.L.: Quasi-Periodic Solutions of Nonlinear Evolution Equations Associated with a 3 × 3 Matrix Spectral Problem. Stud. Appl. Math. 127, 107–140 (2011)
Trogdon, T., Deconinck, B.: Numerical computation of the finite-genus solutions of the Korteweg-de Vries equation via Riemann-Hilbert problems. Appl. Math. Lett. 26, 5–9 (2013)
Trogdon, T., Deconinck, B.: A numerical dressing method for the nonlinear superposition of solutions of the KdV equation. Nonlinearity 27, 67–86 (2014)
Frauendiener, J., Klein, C.: Hyperelliptic theta-functions and spectral methods. J. Comput. Appl. Math 167, 193–218 (2004)
Frauendiener, J., Klein, C.: Hyperelliptic theta-Functions and spectral methods: KdV and KP Solutions, Lett. Math. Phys. 76, 249–267 (2006)
Nakamura, A.: A direct method of calculating periodic wave solutions to nonlinear evolution equations. I. Exact two-periodic wave solution. J. Phys. Soc. Jpn. 47, 1701–1705 (1979)
Nakamura, A.: A direct method of calculating periodic wave solutions to nonlinear evolution equations. II. Exact one- and two-periodic wave solution of the coupled bilinear equations. J. Phys. Soc. Jpn. 48, 1365–1370 (1980)
Hirota, R., Ito, M.: A direct approach to multi-periodic wave solutions to nonlinear evolution Equations. J. Phys. Soc. Jpn. 50, 338–342 (1981)
Fan, E.G., Hon, Y.C.: Quasi-periodic waves and asymptotic behavior for Bogoyavlenskiis breaking soliton equation in (2 + 1) dimensions. Phys. Rev. E. 78, 036607 (2008)
Fan, E.G., Chow, K.W.: On the periodic solutions for both nonlinear differential and difference equations: a unified approach. Phys. Lett. A. 374, 3629–3634 (2010)
Ma, W.X., Zhou, R.G., Gao, L.: Exact one-periodic and two-periodic wave solutions to Hirota bilinear equations in (2 + 1) dimensions. Mod. Phys. Lett. A. 24, 1677–1688 (2009)
Tian, S.F., Zhang, H.Q.: Riemann theta functions periodic wave solutions and rational characteristics for the nonlinear equations. J. Math. Anal. Appl. 371, 58–608 (2010)
Zhang, Y.N., Hu, X.B., Sun, J.Q.: A numerical study of the 3-periodic wave solutions to KdV-type equations. J. Comput. Phys. 355, 566–581 (2018)
Zhang, Y.N., Hu, X.B., He, Y., Sun, J.Q.: A numerical study of the 3-periodic wave solutions to Toda-type equations. Commun. Comput. Phys. 26, 579–598 (2019)
Tzitzeica, G., Acad, C.R.: Sur une nouvelle classe de surfaces. C. R. Acad. Sci. 150, 955–956 (1910)
Tzitzeica, G., Acad, C.R.: Sur une nouvelle classe de surfaces. C. R. Acad. Sci. 150, 1227–1229 (1910)
Yu, S.J., Toda, K., Sasa, N., Fukuyama, T.: N soliton solutions to the Bogoyavlenskii-Schiff equation and a quest for the soliton solution in (3 + 1) dimensions. J. Phys. A: Math. Gen. 31, 3337–3347 (1998)
Toda, K., Yu, S.J., Fukuyama, T.: The Bogoyavlenskii-Schiff hierarchy and integrable equations in (2 + 1) dimensions. Rep. Math. Phys. 44, 247 (1999)
Huang, L.L., Chen, Y.: Nonlocal symmetry and exact solutions of the (2 + 1)-dimensional modified Bogoyavlenskii-Schiff equation. J. Chin. Phys. B. 25, 67–74 (2016)
Kaptsov, O.V., Shanko, Yu.V.: Trilinear representation and the Moutard transformation for the Tzitzeica equation, arXiv:solv-int/9704014 (1997)
Lambert, F., Loris, I., Springael, J., Willox, R.: On the Hirota representation of soliton equations with one tau-function. J. Phys. Soc. Jpn. 70, 605–608 (2001)
Wu, L.H., Geng, X.G., Zhang, J.S.: Algebro-geometric solution to the BulloughCDoddCZhiberCShabat equation. Int. Math. Res. Notices 8, 2141–2167 (2015)
Cherdantzev, I.Y., Sharipov, R.A.: Solitons on a finite-gap background in BulloughC DoddCJiberCShabat model. Int. J. Modern Phys. A 5(15), 3021–3027 (1990)
Kaptsov, O.V., Shan’ko, V.Y. : Multiparameter solutions of the Tzitzeica equation. Differ. Equ. 35(12), 1683–1692 (1999)
Udriste, C., Arsinte, V., Cipu, C.: Tzitzeica and sine-Gordon solitons. Balkan J. Geom. Appl. 16(1), 150–154 (2011)
Wazwaz, A.M.: The tanh method: solitons and periodic solutions for the Dodd-Bullough- Mikhailov and the Tzitzeica-Dodd-Bullough equations. Chaos, Solitons Fractals 25(1), 55–63 (2005)
Madsen, K., Nielsen, H.B., Tingleff, O.: Methods for Non-linear Least Squares Problems, 2nd edn, pp. 60 (2004)
Osborne, A.: Nonlinear Ocean Waves & the Inverse Scattering Transform. Academic Press (2010)
Funding
This work was partially supported by the National Natural Science Foundation of China (Grant nos. 12071447, 11971473, 11871444, 11731014) and Fundamental Research Funds for the Central Universities (Grant no. 201964008).
Author information
Authors and Affiliations
Corresponding author
Additional information
Publisher’s note
Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.
Rights and permissions
About this article
Cite this article
Wang, XX., Sun, JQ. & Zhang, YN. A numerical study on the N-periodic wave solutions of two coupled bilinear equations. Numer Algor 88, 711–728 (2021). https://doi.org/10.1007/s11075-020-01054-w
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11075-020-01054-w
Keywords
- Tzitzeica equation
- (2 + 1)-Dimensional modified Bogoyavlenskii-Schiff equation
- N-Periodic wave solution
- Riemann’s 𝜃-function