A Generalization of Ritz-Variational Method for Solving a Class of Fractional Optimization Problems

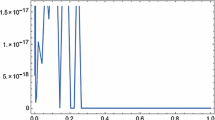
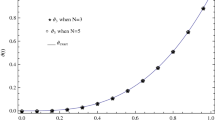
Abstract

This paper presents an approximate method for solving a class of fractional optimization problems with multiple dependent variables with multi-order fractional derivatives and a group of boundary conditions. The fractional derivatives are in the Caputo sense. In the presented method, first, the given optimization problem is transformed into an equivalent variational equality; then, by applying a special form of polynomial basis functions and approximations, the variational equality is reduced to a simple linear system of algebraic equations. It is demonstrated that the derived linear system has a unique solution. We get an approximate solution for the initial optimization problem by solving the final linear system of equations. The choice of polynomial basis functions provides a method with such flexibility that all initial and boundary conditions of the problem can be easily imposed. We extensively discuss the convergence of the method and, finally, present illustrative test examples to demonstrate the validity and applicability of the new technique.