A Family of Two-Grid Partially Penalized Immersed Finite Element Methods for Semi-linear Parabolic Interface Problems | Journal of Scientific Computing Skip to main content
Springer Nature Link
Log in

A Family of Two-Grid Partially Penalized Immersed Finite Element Methods for Semi-linear Parabolic Interface Problems

  • Published:
Journal of Scientific Computing Aims and scope Submit manuscript

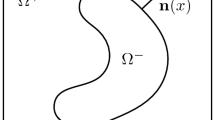
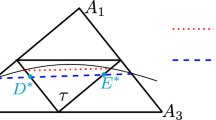
Abstract

In this paper, we present a family of two-grid algorithms for semi-linear parabolic interface problems based on Partially penalized immersed finite element discretizations. Optimal a priori error estimates are derived both in the energy norm and \(L^2\) norm, under the standard piecewise \(H^2\) regularity assumption for the exact solution. For the nonlinear right hand side, we investigate two-grid methods base on Newton method. The efficiency of the two-grid methods is confirmed theoretically and numerically.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16
Fig. 17
Fig. 18
Fig. 19
Fig. 20
Fig. 21
Fig. 22
Fig. 23
Fig. 24
Fig. 25

Similar content being viewed by others

Data Availability

The data supporting the findings of this study is available within the article and its supplementary materials.

References

  1. Adjerid, S., Chaabane, N., Lin, T.: An immersed discontinuous finite element method for stokes interface problems. Comput. Methods Appl. Mech. Eng. 293, 170–190 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  2. Ahn, H.T., Shashkov, M.: Multi-material interface reconstruction on generalized polyhedral meshes. J. Comput. Phys. 226(2), 2096–2132 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  3. Attanayake, C., Senaratne, D.: Convergence of an immersed finite element method for semilinear parabolic interface problems. Appl. Math. Sci. 5(3), 135–147 (2011)

    MathSciNet  MATH  Google Scholar 

  4. Babuška, I.: The finite element method for elliptic equations with discontinuous coefficients. Computing 5(3), 207–213 (1970)

    Article  MathSciNet  MATH  Google Scholar 

  5. Babuska, I.: The finite element method for elliptic equations with discontinuous coefficients. Computing 5(3), 207–213 (1970)

    Article  MathSciNet  MATH  Google Scholar 

  6. Camp, B., Lin, T., Lin, Y., Sun, W.: Quadratic immersed finite element spaces and their approximation capabilities. Adv. Comput. Math. 24(1–4), 81–112 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  7. Chan, K.H., Zhang, K., Zou, J.: Spherical interface dynamos: mathematical theory, finite element approximation, and application. SIAM J. Numer. Anal. 44(5), 1877–1902 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  8. Chen, L., Wei, H., Wen, M.: An interface-fitted mesh generator and virtual element methods for elliptic interface problems. J. Comput. Phys. 334, 327–348 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  9. Chen, Y., Huang, Y.: A multilevel method for finite element solutions for singular two-point boundary value problems. Nat. Sci. J. Xiangtan Univ. 16, 23–26 (1994)

    MathSciNet  Google Scholar 

  10. Chen, Y., Li, Q., Wang, Y., Huang, Y.: Two-grid methods of finite-element solutions for semi-linear elliptic interface problems. Numer. Algorithm 84, 307–330 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  11. Chen, Z., Zou, J.: Finite element methods and their convergence for elliptic and parabolic interface problems. Numer. Math. 79(2), 175–202 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  12. Dawson, C.N., Wheeler, M.F., Woodward, C.S.: A two-grid finite difference scheme for nonlinear parabolic equations. SIAM J. Numer. Anal. 35(2), 435–452 (1998)

    Article  MathSciNet  MATH  Google Scholar 

  13. Gong, Y., Li, B., Li, Z.: Immersed-interface finite-element methods for elliptic interface problems with nonhomogeneous jump conditions. SIAM J. Numer. Anal. 46(1), 472–495 (2008)

    Article  MathSciNet  MATH  Google Scholar 

  14. Guo, R., Lin, T., Zhuang, Q.: Improved error estimation for the partially penalized immersed finite element methods for elliptic interface problems. Int. J. Numer. Anal. Model 16(4), 575–589 (2019)

    MathSciNet  MATH  Google Scholar 

  15. Hansbo, A., Hansbo, P.: An unfitted finite element method, based on Nitsche’s method, for elliptic interface problems. Comput. Meth. Appl. Mech. Eng. 191(47–48), 5537–5552 (2002)

  16. He, Y.: Two-level method based on finite element and Crank-Nicolson extrapolation for the time-dependent Navier–Stokes equations. SIAM J. Numer. Anal. 41(4), 1263–1285 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  17. Huang, J., Zou, J.: A mortar element method for elliptic problems with discontinuous coefficients. IMA J. Numer. Anal. 22(4), 549–576 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  18. Huang, P., Wu, H., Xiao, Y.: An unfitted interface penalty finite element method for elliptic interface problems. Comput. Meth. Appl. Mech. Eng. 323, 439–460 (2017)

    Article  MathSciNet  MATH  Google Scholar 

  19. Kwak, D.Y., Wee, K.T., Chang, K.S.: An analysis of broken \(p_1\)-nonconforming finite element method for interface problems. SIAM J. Numer. Anal. 48(6), 2117–2134 (2009)

    Article  MATH  Google Scholar 

  20. Layton, W.J., Schieweck, F., Yotov, I.: Coupling fluid flow with porous media flow. SIAM J. Numer. Anal. 40(6), 2195–2218 (2002)

    Article  MathSciNet  MATH  Google Scholar 

  21. Li, J., Melenk, J.M., Wohlmuth, B., Zou, J.: Optimal a priori estimates for higher order finite elements for elliptic interface problems. Appl. Numer. Math. 60(1–2), 19–37 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  22. LI, J., Zou, J.: Advances of finite element analysis for elliptic, parabolic and maxwell interface equations. Sci. China Math. 45(7), 1025–1040 (2015)

    Google Scholar 

  23. Li, Z., Ito, K.: The immersed interface method: numerical solutions of PDEs involving interfaces and irregular domains, vol. 33. SIAM (2006)

  24. Li, Z., Lin, T., Lin, Y., Rogers, R.C.: An immersed finite element space and its approximation capability. Numer. Meth. Part. D. E. 20(3), 338–367 (2004)

    Article  MathSciNet  MATH  Google Scholar 

  25. Li, Z., Lin, T., Wu, X.: New cartesian grid methods for interface problems using the finite element formulation. Numer. Math. 96(1), 61–98 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  26. Lin, M., Lin, T., Zhang, H.: Error analysis of an immersed finite element method for Euler-Bernoulli beam interface problems. Int. J. Numer. Anal. Model. 14(6), 822–841 (2017)

    MathSciNet  MATH  Google Scholar 

  27. Lin, T., Lin, Y., Sun, W.W., Wang, Z.: Immersed finite element methods for 4th order differential equations. J. Comput. Appl. Math. 235(13), 3953–3964 (2011)

    Article  MathSciNet  MATH  Google Scholar 

  28. Lin, T., Lin, Y., Zhang, X.: Partially penalized immersed finite element methods for elliptic interface problems. SIAM J. Numer. Anal. 53(2), 1121–1144 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  29. Lin, T., Yang, Q., Zhang, X.: Partially penalized immersed finite element methods for parabolic interface problems. Numer. Meth. Part. D. E. 31(6), 1925–1947 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  30. Lin, T., Zhang, X.: Linear and bilinear immersed finite elements for planar elasticity interface problems. J. Comput. Appl. Math. 236(18), 4681–4699 (2012)

    Article  MathSciNet  MATH  Google Scholar 

  31. Lin, T., Zhuang, Q.: Optimal error bounds for partially penalized immersed finite element methods for parabolic interface problems. J. Comput. Appl. Math. 366, 112401 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  32. Mu, M., Xu, J.: A two-grid method of a mixed Stokes-Darcy model for coupling fluid flow with porous media flow. SIAM J. Numer. Anal. 45(5), 1801–1813 (2007)

    Article  MathSciNet  MATH  Google Scholar 

  33. Nicaise, S., Sändig, A.M.: General interface problems–i. Math. Meth. Appl. Sci. 17(6), 395–429 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  34. Olshanskii, M.A., Reusken, A.: Analysis of a stokes interface problem. Numer. Math. 103(1), 129–149 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  35. Pennes, H.H.: Analysis of tissue and arterial blood temperatures in the resting human forearm. J. Appl. Physiol. 85(1), 5–34 (1998)

    Article  Google Scholar 

  36. Wang, H., Liang, D., Ewing, R.E., Lyons, S.L., Qin, G.: An approximation to miscible fluid flows in porous media with point sources and sinks by an Eulerian–Lagrangian localized adjoint method and mixed finite element methods. SIAM J. Sci. Comput. 22(2), 561–581 (2000)

    Article  MathSciNet  MATH  Google Scholar 

  37. Wang, Y., Chen, Y.: A two-grid method for incompressible miscible displacement problems by mixed finite element and Eulerian–Lagrangian localized adjoint methods. J. Math. Anal. Appl. 468(1), 406–422 (2018)

    Article  MathSciNet  MATH  Google Scholar 

  38. Wang, Y., Chen, Y., Huang, Y.: A two-grid Eulerian–Lagrangian localized adjoint method to miscible displacement problems with dispersion term. Comput. Math. Appl. 80, 54–68 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  39. Wang, Y., Chen, Y., Huang, Y.: A two-grid method for semi-linear elliptic interface problems by partially penalized immersed finite element methods. Math. Comput. Simul. 169, 1–15 (2020)

    Article  MathSciNet  MATH  Google Scholar 

  40. Wang, Y., Chen, Y., Huang, Y., Liu, Y.: Two-grid methods for semi-linear elliptic interface problems by immersed finite element methods. Appl. Math. Mech. (Engl. Ed.) 40(11), 1657–1676 (2019)

    Article  MathSciNet  MATH  Google Scholar 

  41. Wei, H., Chen, L., Huang, Y., Zheng, B.: Adaptive mesh refinement and superconvergence for two-dimensional interface problems. SIAM J. Sci. Comput. 36(4), A1478–A1499 (2014)

    Article  MathSciNet  MATH  Google Scholar 

  42. Wu, H., Xiao, Y.: An unfitted \(hp\)-interface penalty finite element method for elliptic interface problems. Eprint Arxiv 323,(2010)

  43. Xu, J.: A novel two-grid method for semilinear elliptic equations. SIAM J. Sci. Comput. 15(1), 231–237 (1994)

    Article  MathSciNet  MATH  Google Scholar 

  44. Xu, J.: Two-grid discretization techniques for linear and nonlinear PDEs. SIAM J. Numer. Anal. 33(5), 1759–1777 (1996)

    Article  MathSciNet  MATH  Google Scholar 

  45. Xu, J., Zhou, A.: Local and parallel finite element algorithms based on two-grid discretizations. Math. Comput. Am. Math. Soc. 69(231), 881–909 (2000)

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. School of Mathematics and Computational Science, Hunan Key Laboratory for Computation and Simulation in Science and Engineering, Xiangtan University, Xiangtan, 411105, China

    Yang Wang, Yunqing Huang & Huaming Yi

  2. School of Mathematical Sciences, South China Normal University, Guangzhou, 510631, China

    Yanping Chen

Authors
  1. Yang Wang
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Yanping Chen
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Yunqing Huang
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Huaming Yi
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Yanping Chen.

Ethics declarations

Conflict of interest

The authors declare that they have no conflict of interest.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

This work is supported by National Natural Science Foundation of China (41974133, 11971410) and the State Key Program of National Natural Science Foundation of China (11931003) and Project for Hunan National Applied Mathematics Center of Hunan Provincial Science and Technology Department (2020ZYT003) and Postgraduate Scientific Research Innovation Project of Xiangtan University (XDCX2021B109)

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, Y., Chen, Y., Huang, Y. et al. A Family of Two-Grid Partially Penalized Immersed Finite Element Methods for Semi-linear Parabolic Interface Problems. J Sci Comput 88, 80 (2021). https://doi.org/10.1007/s10915-021-01575-z

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s10915-021-01575-z

Keywords

Mathematics Subject Classification