An impossibility result on graph secret sharing | Designs, Codes and Cryptography Skip to main content
Springer Nature Link
Log in

An impossibility result on graph secret sharing

  • Published:
Designs, Codes and Cryptography Aims and scope Submit manuscript

Abstract

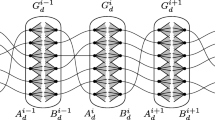
A perfect secret sharing scheme based on a graph G is a randomized distribution of a secret among the vertices of the graph so that the secret can be recovered from the information assigned to vertices at the endpoints of any edge, while the total information assigned to an independent set of vertices is independent (in statistical sense) of the secret itself. The efficiency of a scheme is measured by the amount of information the most heavily loaded vertex receives divided by the amount of information in the secret itself. The (worst case) information ratio of G is the infimum of this number. We calculate the best lower bound on the information ratio for an infinite family of graphs the entropy method can give. We argue that almost all existing constructions for secret sharing schemes are special cases of the generalized vector space construction. We give direct constructions of this type for the first two members of the family, and show that for the other members no such construction exists which would match the bound yielded by the entropy method.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Beimel A., Livne N., Padró C.: Matroids can be far from ideal secret sharing. In: Proceedings of TCC’08, LNCS, vol. 4948, pp. 194–212 (2008).

  2. Beimel A., Ishai Y.: On the power of nonlinear secret sharing schemes. SIAM J. Discrete Math. 19, 258–280 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  3. Beimel A., Livne N.: On matroids and non-ideal secret sharing. In: Halevi S., Rabin T. (eds.) Proceedings of the Third Theory of Cryptography Conference—TCC 2006, LNCS, vol. 3876, pp. 482–501 (2006).

  4. Beimel A., Weinreb E.: Separating the power of monotone span programs over different fields. SIAM J. Comput. 34, 1196–1215 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  5. Blakley G.R.: Safeguarding cryptographic keys. In: Proceedings of the 1979 AFIPS National Computer Conference, pp. 313–317 (1979).

  6. Blundo C., De Santis A., Simone R.D., Vaccaro U.: Tight bounds on the information rate of secret sharing schemes. Des. Codes Cryptogr. 11, 107–110 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  7. Brickell E.F.: Some ideal secret sharing schemes. J. Combin. Math. Combin. Comput. 9, 105–113 (1989)

    MathSciNet  Google Scholar 

  8. Capocelli R.M., De Santis A., Gargano L., Vaccaro U.: On the size of shares of secret sharing schemes. J. Cryptology 6(3), 157–168 (1993)

    Article  MATH  Google Scholar 

  9. Chor B., Kushilevitz E.: Secret sharing over infinite domains. J. Cryptology 6, 87–96 (1993)

    Article  MATH  MathSciNet  Google Scholar 

  10. Csirmaz L.: The size of a share must be large. J. Cryptology 10(4), 223–231 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  11. Csirmaz L.: Secret sharing schemes on graphs. Studia Sci. Math. Hungar. 44, 297–306 (2007)—available as IACR preprint http://eprint.iacr.org/2005/059.

  12. Csirmaz L., Ligeti P.: On an infinite families of graphs with information ratio 2 − 1/k (Special Issue of Computing on the occasion CECC’08 to appear).

  13. Csiszár I., Körner J.: Information Theory. Coding Theorems for Discrete Memoryless Systems. Academic Press, New York (1981)

    MATH  Google Scholar 

  14. Ferrás O., Martí-Farré J., Padró C.: Ideal multipartite secret sharing schemes. In: Advances in Cryptology—EUROCRYPT 2007, LNCS, vol. 4515, pp. 448–465 (2007).

  15. Ingleton A.W.: Conditions for representability and transversability of matroids. In: Proc. Fr. Br. Conf. 1970, pp. 62–27. Springer-Verlag (1971).

  16. Jackson W., Martin K.M.: Perfect secret sharing schemes on five participants. Des. Codes Cryptogr. 9, 233–250 (1996)

    MATH  Google Scholar 

  17. Karchmer M., Widgerson A.: On span programs. In: Proc. of the 8th IEEE Trans. on Information Theory, vol. 29, pp. 102–111 (1993).

  18. Martí-Farré J., Padró C.: Secret sharing schemes with three or four minimal qualified subsets. Des. Codes Cryptogr. 34, 17–34 (2005)

    Article  MATH  MathSciNet  Google Scholar 

  19. Martí-Farré J., Padró C.: On secret sharing schemes, matroids and polymatroids. In: TCC 2007, Lecture Notes in Comput. Sci., vol. 4392, pp. 273–290 (2007).

  20. Matus F.: Matroid representations by partitions. Discrete Math. 203, 169–194 (1999)

    Article  MATH  MathSciNet  Google Scholar 

  21. Matus F.: Adhesivity of polymatroids. Discrete Math. 307(21), 2464–2477 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  22. Shamir A.: How to share a secret. Commun. ACM 22, 612–613 (1979)

    Article  MATH  MathSciNet  Google Scholar 

  23. Stinson D.R.: Decomposition constructions for secret sharing schemes. IEEE Trans. Inform. Theory 40, 118–125 (1994)

    Article  MATH  MathSciNet  Google Scholar 

  24. van Dijk M.: On the information rate of perfect secret sharing schemes. Des. Codes Cryptogr. 12, 143–169 (1997)

    Article  Google Scholar 

  25. van Dijk M., Kevenaar T., Schrijen G., Tuyls P.: Improved constructions of secret sharing schemes by applying (λ,ω)-decompositions. Inform. Process. Lett. 99(4), 154–157 (2006)

    Article  MathSciNet  Google Scholar 

  26. Welsh D.J.A.: Matroid Theory. Academic Press, London (1976)

    MATH  Google Scholar 

  27. Zhang Z., Yeung R.W.: On characterization of entropy function via information inequalities. IEEE Trans. Inform. Theory 44, 1440–1452 (1998)

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Central European University, Budapest, Hungary

    László Csirmaz

  2. University of Debrecen, Debrecen, Hungary

    László Csirmaz

Authors
  1. László Csirmaz
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to László Csirmaz.

Additional information

Communicated by Daniel Panario.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Csirmaz, L. An impossibility result on graph secret sharing. Des. Codes Cryptogr. 53, 195–209 (2009). https://doi.org/10.1007/s10623-009-9304-0

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10623-009-9304-0

Keywords

Mathematics Subject Classifications (2000)

Search

Navigation