On attraction of linearly constrained Lagrangian methods and of stabilized and quasi-Newton SQP methods to critical multipliers

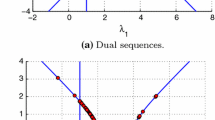
Abstract

It has been previously demonstrated that in the case when a Lagrange multiplier associated to a given solution is not unique, Newton iterations [e.g., those of sequential quadratic programming (SQP)] have a tendency to converge to special multipliers, called critical multipliers (when such critical multipliers exist). This fact is of importance because critical multipliers violate the second-order sufficient optimality conditions, and this was shown to be the reason for slow convergence typically observed for problems with degenerate constraints (convergence to noncritical multipliers results in superlinear rate despite degeneracy). Some theoretical and numerical validation of this phenomenon can be found in Izmailov and Solodov (Comput Optim Appl 42:231–264, 2009; Math Program 117:271–304, 2009). However, previous studies concerned the basic forms of Newton iterations. The question remained whether the attraction phenomenon still persists for relevant modifications, as well as in professional implementations. In this paper, we answer this question in the affirmative by presenting numerical results for the well known MINOS and SNOPT software packages applied to a collection of degenerate problems. We also extend previous theoretical considerations to the linearly constrained Lagrangian methods and to the quasi-Newton SQP, on which MINOS and SNOPT are based. Experiments also show that in the stabilized version of SQP the attraction phenomenon still exists but appears less persistent.