An extended EDAS method under four-branch fuzzy environments and its application in credit evaluation for micro and small entrepreneurs | Soft Computing Skip to main content
Springer Nature Link
Log in

An extended EDAS method under four-branch fuzzy environments and its application in credit evaluation for micro and small entrepreneurs

  • Methodologies and Application
  • Published:
Soft Computing Aims and scope Submit manuscript

Abstract

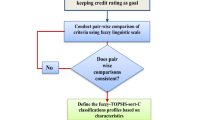
With Chinese economic development in a new normal state, micro and small corporations have increasingly played an important role. The credit evaluation for micro and small corporations can contribute to efficient financial decisions. Traditional methods for the corporate credit evaluation rarely consider fuzzy features of micro and small entrepreneurs. Since the credit evaluation procedure for entrepreneurs consists of some alternatives and influential indicators, it can be recognized as a multi-criteria decision-making (MCDM) problem. To better express uncertainty information, a four-branch fuzzy set (FBFS) is presented to deal with the issue, which is characterized by a truth-membership function, an unknown-membership function and a falsity-membership function. In the paper, to handle the MCDM problems under four-branch fuzzy environments, the evaluation based on distance from average solution (EDAS) method is extended and utilized. These obvious improvement places include integrating an interval weight vector, determining an average solution, transforming a decision matrix and analyzing a change trend of the coefficients. To demonstrate the applicability and rationality of the proposed method in practice, an illustrative example is implemented, concerning a credit evaluation for some micro and small entrepreneurs. Its feasibility and validity are further verified by a sensitivity analysis and a comparative analysis. The results show the FBFSs and extended EDAS method can well match the reality and appropriately handle the credit evaluation problems for micro and small entrepreneurs.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  • Atanassov KT (1986) Intuitionistic fuzzy sets. Fuzzy Sets Syst 20:87–96

    Article  MATH  Google Scholar 

  • Atanassov KT (1989) More on intuitionistic fuzzy sets. Fuzzy Sets Syst 33:37–46

    Article  MathSciNet  MATH  Google Scholar 

  • Atanassov KT, Gargov G (1989) Interval-valued intuitionistic fuzzy sets. Fuzzy Sets Syst 31:343–349

    Article  MathSciNet  MATH  Google Scholar 

  • Bustince H, Burillo P (1996) Vague sets are intuitionistic fuzzy sets. Fuzzy Sets Syst 79:403–405

    Article  MathSciNet  MATH  Google Scholar 

  • Cao YX, Zhou H, Wang JQ (2016) An approach to interval-valued intuitionistic stochastic multi-criteria decision-making using set pair analysis. Int J Mach Learn Cybern. https://doi.org/10.1007/s13042-016-0589-9

    Article  Google Scholar 

  • Chen N, Xu ZS, Xia MM (2013) Correlation coefficients of hesitant fuzzy sets and their applications to clustering analysis. Appl Math Model 37:2197–2211

    Article  MathSciNet  MATH  Google Scholar 

  • Chen ZC, Liu PH, Pei Z (2015) An approach to multiple attribute group decision making based on linguistic intuitionistic fuzzy numbers. Int J Comput Intell Syst 8:747–760

    Article  Google Scholar 

  • Cuong BC (2014) Picture fuzzy sets. J Comput Sci Cybern 30:409–420

    Google Scholar 

  • Farhadinia B (2014) Correlation for dual hesitant fuzzy sets and dual interval-valued hesitant fuzzy sets. Int J Intell Syst 29:184–205

    Article  Google Scholar 

  • Gau WL, Buehrer DJ (1993) Vague sets. IEEE Trans Syst Man Cybern 23:610–614

    Article  MATH  Google Scholar 

  • Grzegorzewski P (2004) Intuitionistic fuzzy numbers-principles, metrics and ranking. In: Atanassov KT, Hryniewicz O, Kacprzyk J (eds) Soft computing foundations and theoretical aspects. Academic House Exit, Warszawa, pp 235–249

    Google Scholar 

  • Hajek P, Michalak K (2013) Feature selection in corporate credit rating prediction. Knowl-Based Syst 51:72–84

    Article  Google Scholar 

  • He Y, Xu ZS, Gu J (2016) An approach to group decision making with hesitant information and its application in credit risk evaluation of enterprises. Appl Soft Comput 43:159–169

    Article  Google Scholar 

  • Ji Y, Huang GH, Sun W (2015) Risk assessment of hydropower stations through an integrated fuzzy entropy-weight multiple criteria decision making method: a case study of the Xiangxi River. Expert Syst Appl 42(12):5380–5389

    Article  Google Scholar 

  • Keshavarz-Ghorabaee M, Zavadskas EK, Amiri M, Turskis Z (2016) Extended EDAS method for fuzzy multi-criteria decision-making: an application to supplier selection. Int J Comput Commun Control 11:358–371

    Article  Google Scholar 

  • Keshavarz-Ghorabaee M, Zavadskas EK, Olfat L, Turskis Z (2015) Multicriteria inventory classification using a new method of evaluation based on distance from average solution (EDAS). Informatica 26:435–451

    Article  Google Scholar 

  • Khashman A (2010) Neural networks for credit risk evaluation: investigation of different neural models and learning schemes. Expert Syst Appl 37(9):6233–6239

    Article  Google Scholar 

  • Kim KJ, Ahn HC (2012) A corporate credit rating model using multi-class support vector machines with an ordinal pairwise partitioning approach. Comput Oper Res 39(8):1800–1811

    Article  MathSciNet  MATH  Google Scholar 

  • Lee YC (2007) Application of support vector machines to corporate credit rating prediction. Expert Syst Appl 33(1):67–74

    Article  Google Scholar 

  • Li YY, Zhang HY, Wang JQ (2017) Linguistic neutrosophic sets and its application to multi-criteria decision-making problems. Int J Uncertain Quant. https://doi.org/10.1615/Int.J.UncertaintyQuantification.2017019627

    Article  Google Scholar 

  • Liang RX, Wang JQ, Zhang HY (2017) A multi-criteria decision-making method based on single-valued trapezoidal neutrosophic preference relations with complete weight information. Neural Comput Appl. https://doi.org/10.1007/s00521-017-2925-8

    Article  Google Scholar 

  • Liu PD, Shi LL (2015) The generalized hybrid weighted average operator based on interval neutrosophic hesitant set and its application to multiple attribute decision making. Neural Comput Appl 26:457–471

    Article  Google Scholar 

  • Luo CC, Wu DS, Wu DX (2017) A deep learning approach for credit scoring using credit default swaps. Eng Appl Artif Intell 65:465–470

    Article  Google Scholar 

  • Lupiánez FG (2009) Interval neutrosophic sets and topology. Kybernetes 38:621–624

    Article  MATH  Google Scholar 

  • Meng FY, Chen XH, Zhang Q (2014) Multi-attribute decision analysis under a linguistic hesitant fuzzy environment. Inf Sci 267:287–305

    Article  MathSciNet  MATH  Google Scholar 

  • Nikolic N, Zarkic-Joksimovic N, Stojanovski D, Joksimovic I (2013) The application of brute force logistic regression to corporate credit scoring models: evidence from Serbian financial statements. Expert Syst Appl 40(15):5932–5944

    Article  Google Scholar 

  • Peng JJ, Wang JQ, Wang J, Chen XH (2014) Multicriteria decision-making approach with hesitant interval-valued intuitionistic fuzzy sets. Sci World J. https://doi.org/10.1155/2014/868515

    Article  Google Scholar 

  • Peng JJ, Wang JQ, Yang LJ, Qian J (2017a) A novel multi-criteria group decision-making approach using simplified neutrosophic information. Int J Uncertain Quant. https://doi.org/10.1615/Int.J.UncertaintyQuantification2017020126

    Article  Google Scholar 

  • Peng JJ, Wang JQ, Yang WE (2017b) A multi-valued neutrosophic qualitative flexible approach based on likelihood for multi-criteria decision-making problems. Int J Syst Sci 48:425–435

    Article  MathSciNet  MATH  Google Scholar 

  • Petropoulos A, Chatzis SP, Xanthopoulos S (2016) A novel corporate credit rating system based on Student’s-t hidden Markov models. Expert Syst Appl 53:87–105

    Article  Google Scholar 

  • Siddiqi N (2005) Credit risk scorecards: developing and implementing intelligent credit scoring. Wiley, London

    Google Scholar 

  • Singh P (2015) Correlation coefficients for picture fuzzy sets. J Intell Fuzzy Syst 28:591–604

    Article  MathSciNet  MATH  Google Scholar 

  • Smarandache F (2003) A unifying field in logics: neutrosophic logic, neutrosophy, neutrosophic set, neutrosophic probability. American Research Press, Rehoboth, Mass, USA

    MATH  Google Scholar 

  • Smarandache F (2004) Neutrosophic set-a generalization of the intuitionistic fuzzy set. J Def Resour Manag 24:38–42

    Google Scholar 

  • Smarandache F, Kandasamy WBV (1999) Analysis of social aspects of migrant labourers living with hiv/aids using fuzzy theory and neutrosophic cognitive maps. IEEE Softw 16:115–117

    Google Scholar 

  • Son LH (2015) DPFCM: a novel distributed picture fuzzy clustering method on picture fuzzy sets. Expert Syst Appl 42:51–66

    Article  Google Scholar 

  • Stević Ž, Tanackov I, Vasiljević M, Vesković S (2016) Evaluation in logistics using combined AHP and EDAS method. International Symposium on Operational Research SYM-OP-IS. Tara, Serbia, pp 309–313

    Google Scholar 

  • Tian ZP, Wang J, Zhang HY, Wang JQ (2016) Multi-criteria decision-making based on generalized prioritized aggregation operators under simplified neutrosophic uncertain linguistic environment. Int J Mach Learn Cybern. https://doi.org/10.1007/s13042-016-0552-9

    Article  Google Scholar 

  • Torra V (2010) Hesitant fuzzy sets. Int J Intell Syst 25:529–539

    MATH  Google Scholar 

  • Torra V, Narukawa Y (2009) On hesitant fuzzy sets and decision. The 18th IEEE International Conference on Fuzzy Systems. Jeju Island, South Korea, pp 1378–1382

    Google Scholar 

  • Wan SP, Dong JY (2014) A possibility degree method for interval-valued intuitionistic fuzzy multi-attribute group decision making. J Comput Syst Sci 80:237–256

    Article  MathSciNet  MATH  Google Scholar 

  • Wang CH, Wang JQ (2016) A multi-criteria decision-making method based on triangular intuitionistic fuzzy preference information. Intell Autom Soft Comput 22:473–482

    Article  Google Scholar 

  • Wang HB, Smarandache F,Zhang YQ,Sunderraman R (2005) Interval neutrosophic sets and logic: theory and applications in computing. Neutrosophic Book Series: No. 5. Hexis, Phoenix, Ariz, USA

  • Wang HB, Smarandache F, Zhang YQ, Sunderraman R (2010) Single valued neutrosophic sets. Multispace Multistruct 4:410–413

    MATH  Google Scholar 

  • Wang JQ, Wu JT, Wang J, Zhang HY, Chen XH (2014) Interval-valued hesitant fuzzy linguistic sets and their applications in multi-criteria decision-making problems. Inf Sci 288:55–72

    Article  MathSciNet  MATH  Google Scholar 

  • Wang JQ, Yang Y, Li L (2016) Multi-criteria decision-making method based on single valued neutrosophic linguistic Maclaurin symmetric mean operators. Neural Comput Appl. https://doi.org/10.1007/s00521-016-2747-0

    Article  Google Scholar 

  • Wu TC, Hsu MF (2012) Credit risk assessment and decision making by a fusion approach. Knowl-Based Syst 35:102–110

    Article  Google Scholar 

  • Wu XH, Wang JQ (2017) Cross-entropy measures of multi-valued neutrosophic sets and its application in selecting middle-level manager. Int J Uncertain Quant 7(2):155–176

    Article  Google Scholar 

  • Wu ZB, Xu JP (2016) Possibility distribution-based approach for MAGDM with hesitant fuzzy linguistic information. IEEE Trans Cybern 46(3):694–705

    Article  Google Scholar 

  • Xia YF, Liu CZ, Li YY, Liu NN (2017) A boosted decision tree approach using Bayesian hyper-parameter optimization for credit scoring. Expert Syst Appl 78:225–241

    Article  Google Scholar 

  • Yager RR (2013) Pythagorean fuzzy subsets. in: IFSA World Congress and NAFIPS Annual Meeting (IFSA/NAFIPS), Edmonton, AB, Canada, pp 57–61

  • Yager RR (2014) Pythagorean membership grades in multi-criteria decision making. IEEE Trans Fuzzy Syst 22:958–965

    Article  Google Scholar 

  • Ye J (2014a) A multicriteria decision-making method using aggregation operators for simplified neutrosophic sets. J Intell Fuzzy Syst Appl Eng Technol 26:2459–2466

    MathSciNet  MATH  Google Scholar 

  • Ye J (2014b) Correlation coefficient of dual hesitant fuzzy sets and its application to multiple attribute decision making. Appl Math Model 38:659–666

    Article  MathSciNet  MATH  Google Scholar 

  • Ye J (2014c) Multiple-attribute decision-making method under a single-valued neutrosophic hesitant fuzzy environment. J Intell Syst 24:23–36

    Article  Google Scholar 

  • Yu LA, Wang SY, Lai KK (2009) An intelligent-agent-based fuzzy group decision making model for financial multicriteria decision support: The case of credit scoring. Eur J Oper Res 195(3):942–959

    Article  MathSciNet  MATH  Google Scholar 

  • Zadeh LA (1965) Fuzzy sets. Inf Control 8:338–356

    Article  MATH  Google Scholar 

  • Zadeh LA (1973) Outline of a new approach to the analysis of complex systems and decision processes interval-valued fuzzy sets. IEEE Trans Syst Man Cybern 3:28–44

    Article  MathSciNet  MATH  Google Scholar 

  • Zhang HY, Peng HG, Wang J, Wang JQ (2017) An extended outranking approach for multi-criteria decision-making problems with linguistic intuitionistic fuzzy numbers. Appl Soft Comput 59:462–474

    Article  Google Scholar 

  • Zhang XL (2016) Multicriteria Pythagorean fuzzy decision analysis: a hierarchical QUALIFLEX approach with the closeness index-based ranking methods. Inf Sci 330:104–124

    Article  Google Scholar 

  • Zhao XF, Lin R, Wei GW (2014) Hesitant triangular fuzzy information aggregation based on Einstein operations and their application to multiple attribute decision making. Expert Syst Appl 41:1086–1094

    Article  Google Scholar 

  • Zhong HM, Miao CY, Shen ZQ, Feng YH (2014) Comparing the learning effectiveness of BP, ELM, I-ELM, and SVM for corporate credit ratings. Neurocomputing 128:285–295

    Article  Google Scholar 

  • Zhu XQ, Li JP, Wu DS, Wang HY, Liang CZ (2013) Balancing accuracy, complexity and interpretability in consumer credit decision making: a C-TOPSIS classification approach. Knowl-Based Syst 52:258–267

    Article  Google Scholar 

Download references

Acknowledgements

This work was supported by the National Social Science Foundation of China (Grant No. 15BJY163), Natural Science Foundation of Hunan Province (Grant No. 2018JJ2198), and Key Scientific Research Projects of Hunan Education Department (Grant No. 19A276).

Author information

Authors and Affiliations

  1. Key Laboratory of Hunan Province for New Retail Virtual Reality Technology, Hunan University of Technology and Business, Changsha, 410205, China

    Jian Ren & Shao-qian Yu

  2. Mobile E-Business Collaborative Innovation Center of Hunan Province, Hunan University of Technology and Business, Changsha, 410205, China

    Chun-hua Hu

  3. School of Business, Hunan University of Science and Technology, Xiangtan, 411201, China

    Peng-fei Cheng

Authors
  1. Jian Ren
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Chun-hua Hu
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Shao-qian Yu
    View author publications

    You can also search for this author inPubMed Google Scholar

  4. Peng-fei Cheng
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Jian Ren.

Ethics declarations

Conflict of interest

Authors Ren, Hu, Yu, and Cheng declare they have no conflict of interest regarding the publication of this paper.

Ethical approval

All procedures performed in studies involving human participants were in accordance with the ethical standards of the institutional and/or national research committee and with the 1964 Helsinki declaration and its later amendments or comparable ethical standards. This article does not contain any studies with animals performed by any of the authors.

Informed consent

Informed consent was obtained from all individual participants included in the study.

Additional information

Communicated by V. Loia.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ren, J., Hu, Ch., Yu, Sq. et al. An extended EDAS method under four-branch fuzzy environments and its application in credit evaluation for micro and small entrepreneurs. Soft Comput 25, 2777–2792 (2021). https://doi.org/10.1007/s00500-020-05337-1

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00500-020-05337-1

Keywords