2-D DOA Estimation Algorithm for Non-circular Signal Based on Fourth-Order Cumulant | Circuits, Systems, and Signal Processing Skip to main content
Springer Nature Link
Log in

2-D DOA Estimation Algorithm for Non-circular Signal Based on Fourth-Order Cumulant

  • Short Paper
  • Published:
Circuits, Systems, and Signal Processing Aims and scope Submit manuscript

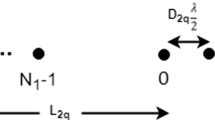
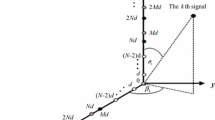
Abstract

Currently, the covariance matrix (CM) or pseudo CM of the received signal is processed to improve the degrees of freedom (DOFs) and estimation accuracy. This is where the second-order cumulant (SOC) in the two-dimensional (2-D) direction-of-arrival (DOA) estimation algorithm based on sparse arrays comes into play. This paper proposes a 2-D DOA estimation approach for non-circular (NC) signals based on fourth-order cumulant (FOC), which fully exploits its inherent benefits in virtual array expansion and denoising white Gaussian noise in comparison with SOC. The proposed algorithm, on the other hand, discards the original 2-D spectral peak search theory and uses the properties of the NC signal and parallel sparse array (PSA) to place the 2-D angle information in Toeplitz matrices for estimation, greatly reducing the computational complexity of processing the FOC without compromising estimation accuracy. Simulation results demonstrate that the proposed algorithm outperforms the conventional algorithms in terms of estimation accuracy while utilizing fewer physical sensors and a smaller array aperture.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6

Similar content being viewed by others

Availability of Data and Materials

Not applicable.

References

  1. J. Cai, W. Liu, R. Zong, B. Wu, Sparse array extension for non-circular signals with subspace and compressive sensing based DOA estimation methods. Signal Process. 145, 59–67 (2018)

    Article  Google Scholar 

  2. E.J. Candes, J. Romberg, T. Tao, Robust uncertainty principles: exact signal reconstruction from highly incomplete frequency information. IEEE Trans. Inf. Theory 52(2), 489–509 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  3. M.C. Dogan, J.M. Mendel, Applications of cumulants to array processing. I. Aperture extension and array calibration. IEEE Trans. Signal Process. 43(5), 1200–1216 (1995)

    Article  Google Scholar 

  4. D.L. Donoho, Compressed sensing. IEEE Trans. Inf. Theory 52(4), 1289–1306 (2006)

    Article  MathSciNet  MATH  Google Scholar 

  5. Z. Huang, X. Li, P. Huang, W. Wang, 2-D DOA estimation for incoherently distributed sources considering mixed circular and noncircular signals in massive MIMO system. IEEE Access 7, 106900–106911 (2019)

    Article  Google Scholar 

  6. B. Li, X. Zhang, J. Li, P. Ma, DOA estimation of non-circular source for large uniform linear array with a single snapshot: extended DFT method. IEEE Commun. Lett. 25(12), 3843–3847 (2021)

    Article  Google Scholar 

  7. L. Li, M. Jia, J. Wang, DOA estimation of multiple speech sources based on the single-source point detection using an FOA microphone. Appl. Acoust. 195, 108830 (2022)

    Article  Google Scholar 

  8. Z. Li, X. Zhang, J. Shen, 2D-DOA estimation of strictly noncircular sources utilizing connection-matrix for L-shaped array. IEEE Wirel. Commun. Lett. 10(2), 296–300 (2020)

    Article  Google Scholar 

  9. S. Liu, J. Zhao, Y. Zhang, D. Wu, 2D DOA estimation algorithm by nested acoustic vector-sensor array. Circuits Syst. Signal Process. 41(2), 1115–1130 (2022)

    Article  Google Scholar 

  10. T. Long, J. Chen, G. Huang, J. Benesty, I. Cohen, Acoustic source localization based on geometric projection in reverberant and noisy environments. IEEE J. Sel. Top. Signal Process. 13(1), 143–155 (2019)

    Article  Google Scholar 

  11. S. Qin, Y.D. Zhang, M.G. Amin, Generalized coprime array configurations for direction-of-arrival estimation. IEEE Trans. Signal Process. 63(6), 1377–1390 (2015)

    Article  MathSciNet  MATH  Google Scholar 

  12. S. Qin, Y.D. Zhang, M.G. Amin, Improved two-dimensional DOA estimation using parallel coprime arrays. Signal Process. 172, 107428 (2020)

    Article  Google Scholar 

  13. Q. Shen, W. Liu, W. Cui, W. Siliang, Extension of co-prime arrays based on the fourth-order difference co-array concept. IEEE Signal Process. Lett. 23(5), 615–619 (2016)

    Article  Google Scholar 

  14. P.P. Vaidyanathan, P. Pal, Sparse sensing with co-prime samplers and arrays. IEEE Trans. Signal Process. 59(2), 573–586 (2010)

    Article  MathSciNet  MATH  Google Scholar 

  15. Z. Zheng, M. Shilin, Two-dimensional DOA estimation using two parallel nested arrays. IEEE Commun. Lett. 24(3), 568–571 (2019)

    Article  Google Scholar 

  16. Z. Zheng, M. Shilin, 2-D direction finding with pair-matching operation for L-shaped nested array. IEEE Commun. Lett. 25(3), 975–979 (2020)

    Article  Google Scholar 

Download references

Funding

Not applicable.

Author information

Authors and Affiliations

  1. Sichuan University, Chengdu, 610065, China

    Jiawei Wang & Qin Shu

  2. Southwest Institute of Technical Physics, Chengdu, 610065, China

    Yunxiu Yang

  3. The Beijing Institute of Remote Sensing Equipment, Beijing, 100005, China

    Wendong Chen

Authors
  1. Jiawei Wang
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Yunxiu Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Wendong Chen
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Qin Shu
    View author publications

    You can also search for this author in PubMed Google Scholar

Contributions

JW and QS contributed to the conception of the study; JW performed the data analyses and wrote the manuscript; YY and WC contributed significantly to analysis and manuscript preparation.

Corresponding author

Correspondence to Qin Shu.

Ethics declarations

Competing interests

Not applicable.

Ethics Approval

Not applicable.

Consent to Participate

Not applicable.

Consent for Publication

Not applicable.

Code Availability

Not applicable.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Wang, J., Yang, Y., Chen, W. et al. 2-D DOA Estimation Algorithm for Non-circular Signal Based on Fourth-Order Cumulant. Circuits Syst Signal Process 42, 2480–2493 (2023). https://doi.org/10.1007/s00034-022-02218-w

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00034-022-02218-w

Keywords

Search

Navigation