On a lattice point problem of L. Moser II | Combinatorica Skip to main content
Springer Nature Link
Log in

On a lattice point problem of L. Moser II

  • Published:
Combinatorica Aims and scope Submit manuscript

Abstract

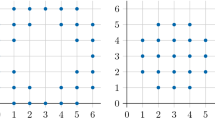
In this paper we complete the proof of the following conjecture of L. Moser: Any convex region of arean can be placed on the plane so as to cover ≧n+f(n) lattice points, wheref(n) →∞.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. J. Beck, On a lattice point problem of L. Moser, I,Combinatorica 8 (1988), 21–47.

    Google Scholar 

  2. W. Blaschke,Kreis und Kugel, Leipzig: Gröschen 1916, New York: Chelsea 1949, Berlin: de Gruyter 1956.

    Google Scholar 

  3. W. Blaschke,Differentialgeometrie II, Affine Differentialgeometrie, Berlin: Springer 1923.

    Google Scholar 

  4. L. Fejes Tóth,Lagerungen in der Ebene, auf der Kugel und im Raum, Berlin: Springer 1953, 1972.

    Google Scholar 

  5. D. Koutroufiotis, On Blaschke's rolling theorem,Arch. Math. 23 (1972), 655–660.

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Eötvös Loránd University, Budapest, Hungary

    József Beck

Authors
  1. József Beck
    View author publications

    You can also search for this author in PubMed Google Scholar

Rights and permissions

Reprints and permissions

About this article

Cite this article

Beck, J. On a lattice point problem of L. Moser II. Combinatorica 8, 159–176 (1988). https://doi.org/10.1007/BF02122797

Download citation

  • Received:

  • Revised:

  • Issue Date:

  • DOI: https://doi.org/10.1007/BF02122797

AMS subject classification

Search

Navigation