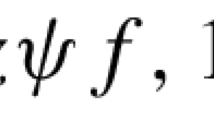Abstract
The problem of approximating Hankel operators of infinite rank by finite-rank Hankel operators is considered. For efficiency, truncated infinite Hankel matrices Γn of Γ are utilized. In this paper for any compact Hankel operator Γ of the Wiener class, we derive the rate of l2-convergence of the Schmidt pairs of Γn to the corresponding Schmidt pairs of Γ. For a certain subclass of Hankel operators of the Wiener class, we also obtain the rate of l1-convergence. In addition, an upper bound for the rate of uniform convergence of the rational symbols of best rank-k Hankel approximants of Γn to the corresponding rational symbol of the best rank-k Hankel approximant to Γ asn → ∞ is derived.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
V. M. Adamjan, D. Z. Arov, and M. G. Krein, Analytic properties of Schmidt pairs for a Hankel operator and the generalized Schur-Takagi problem,Math. USSR-Sb.,15 (1971), 31–73.
C. K. Chui, X. Li, and J. D. Ward, System reduction via truncated Hankel matrices,Math. Control Signals Systems,4 (1991), 161–175.
F. R. Gantmacher,Theory of Matrices, 2nd edn., Nauka, Moscow, 1966; English transl. of 1st edn., Chelsea, New York, 1959.
K. Glover, All optimal Hankel-norm approximations of linear multivariable systems and their L∞ error bounds,Internat. J. Control,39 (1984), 1115–1193.
K. Glover, J. Lam, and J. Partington, Rational approximation of a class of infinite dimensional systems: the L2 case,J. Approx. Theory, to appear.
I. C. Gohberg and M. G. Krein,Introduction to the Theory of Linear Nonselfadjoint Operators, Nauka, Moscow, 1965; English transl., Translations of Mathematical Monographs, Vol. 18, American Mathematical Society, Providence, RI, 1969.
E. Hayashi, L. N. Trefethen, and M. H. Gutknecht, The CF table,Constr. Approx.,6 (1990), 195–223.
S. Y. Kung, Optimal Hankel-norm model reductions: Scalar systems,Proceedings of the 1980 Joint Automatic Control Conference, Vol. 2, San Francisco, CA, paper FA8-D.
Z. Nehari, On bounded bilinear forms,Ann. of Math.,65 (1957), 153–162.
Author information
Authors and Affiliations
Additional information
Supported by SDIO/IST managed by the U.S. Army under Contract No. DAAL03-87-K-0025 and also supported by the National Science Foundation under Grant No. DMS 89-01345.
Rights and permissions
About this article
Cite this article
Chui, C.K., Li, X. & Ward, J.D. Rate of convergence of schmidt pairs and rational functions corresponding to best approximants of truncated hankel operators. Math. Control Signal Systems 5, 67–79 (1992). https://doi.org/10.1007/BF01211976
Received:
Revised:
Issue Date:
DOI: https://doi.org/10.1007/BF01211976