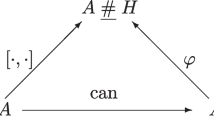
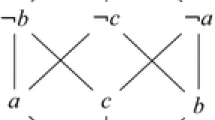
Abstract
An equational theory (a ‘Birkhoff theorem’) for functorial partial algebras is established via the corresponding theory for functorial total algebras.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
References
Burmeister, P.: A model theoretic oriented approach to partial algebras, Introduction to theory and application of partial algebras, Pt. 1, Mathematical Research 32, Berlin, 1986.
Hoehnke, H.-J.: Allgemeine Algebra der Automaten, in L. Budach and H.-J. Hoehnke, Automaten und Funktoren, Teil A, Berlin, 1975.
Hoehnke, H.-J.: On partial recursive definitions and programs, in Fundamentals of Computation Theory, Internat. FCT-Conference, Poznán-Kórnik, 1977, Lecture Notes in Computer Science, Vol. 56, Berlin, 1977, pp. 260–274.
Hoehnke, H.-J.: On partial algebras, in Universal Algebra, Esztergom, 1977, Colloq. Math. Soc. J. Bolyai 29, Amsterdam, 1981, pp. 373–412.
Hoehnke, H.-J.: Monoidal Structure of Mal'cev Clones, Their Theories and Completions, Preprint P-Math-34/87, Karl-Weierstraß-Inst. f. Math., Akad. d. Wiss. d. DDR, Berlin, 1987.
Hoehnke, H.-J.: On certain classes of categories and monoids constructed from abstract Mal'cev clones, I, in Universal and Applied Algebra, Turawa, Poland, 1988, Proceedings of the V Universal Algebra Symposium, Singapore, 1989, pp. 149–176.
Hoehnke, H.-J.: On certain classes of categories and monoids constructed from abstract Mal'cev clones, IV, in Proceedings of the IV Conference on Discrete Mathematics, Potsdam, 1993, General Algebra and Discrete Mathematics, Berlin, 1995, pp. 137–167.
Hoehnke, H.-J.: Monoidal structure of Mal'cev clones and their completions, submitted.
Hoehnke, H.-J.: Remarks and problems on varieties and quasi-varieties, clones and quasiclones, submitted.
Schreckenberger, J.: Über die Einbettung von dht-symmetrischen Kategorien in die Kategorie 021 der partiellen Abbildungen zwischen Mengen, Berlin: Akad. d. Wiss. d. DDR, Zentralinst. f. Math., 1980, Preprint P-12/80.
Schreckenberger, J.: Zur Theorie der dht-symmetrischen Kategorien, Diss. (B): Päd. Hochschule Potsdam, Math.-Naturwiss. Fak., Potsdam, 1984.
Schreckenberger, J.: Zur Axiomatik von Kategorien partieller Morphismen, Beiträge Algebra Geom. 24 (1987), 83–98.
Schreckenberger, J.: Mehrfache Partialisierung von Kategorien, Beiträge Algebra Geom. 25 (1987), 109–122.
Vogel, H.-J.: Eine kategorientheoretische Sprache zur Beschreibung von Birkhoff-Algebren, 021 Berlin: Akad. d. Wiss. d. DDR, Inst. f. Math., 1984. (Report; R-MATH-06/84)=Diss. (B): Päd Hochschule Potsdam, Math.-Naturwiss. Fak., Potsdam, 1983.
Author information
Authors and Affiliations
Additional information
This work was done with partial support of the DFG (BRD).
Rights and permissions
About this article
Cite this article
Hoehnke, HJ. A Birkhoff theorem for partial algebras via completion. Appl Categor Struct 4, 371–386 (1996). https://doi.org/10.1007/BF00122685
Received:
Accepted:
Issue Date:
DOI: https://doi.org/10.1007/BF00122685