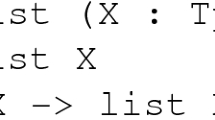What Are Iteration Theories? | SpringerLink
Skip to main content
Springer Nature Link
Log in

What Are Iteration Theories?

  • Conference paper
Mathematical Foundations of Computer Science 2007 (MFCS 2007)

Part of the book series: Lecture Notes in Computer Science ((LNTCS,volume 4708))

  • 1237 Accesses

Abstract

We prove that iteration theories can be introduced as algebras for the monad on the category of signatures assigning to every signature  the rational--tree signature. This supports the result that iteration theories axiomatize precisely the equational properties of least fixed points in domain theory:  is the monad of free rational theories and every free rational theory has a continuous completion.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Institutional subscriptions

Preview

Unable to display preview. Download preview PDF.

Unable to display preview. Download preview PDF.

Similar content being viewed by others

References

  1. Adámek, J., Milius, S., Velebil, J.: Elgot Algebras. Logical Methods in Computer Science 2(5:4), 1–31 (2006)

    Google Scholar 

  2. Bénabou, J.: Structures algébriques dans les catégories. Cah. Topol. Géom. Différ. Catég. 10, 1–126 (1968)

    Google Scholar 

  3. Bloom, S.L., Ésik, Z.: Iteration Theories. Springer, Heidelberg (1993)

    MATH  Google Scholar 

  4. Bloom, S.L., Ésik, Z.: Fixed-point operations on ccc’s, Part I. Theoret. Comput. Sci. 155, 1–38 (1996)

    Article  MATH  MathSciNet  Google Scholar 

  5. Bloom, S.L., Ésik, Z.: The equational logic of fixed points. Theoret. Comput. Sci. 179, 1–60 (1997)

    Article  MATH  MathSciNet  Google Scholar 

  6. Ésik, Z.: Axiomatizing iteration categories. Acta Cybernetica 14, 65–82 (1999)

    MATH  MathSciNet  Google Scholar 

  7. Ginali, S.: Regular trees and the free iterative theory. J. Comput. Syst. Sci. 18, 228–242 (1979)

    Article  MATH  MathSciNet  Google Scholar 

  8. Kelly, G.M., Power, J.: Adjunctions whose units are coequalizers and presentations of finitary enriched monads. J. Pure Appl. Algebra 89, 163–179 (1993)

    Article  MATH  MathSciNet  Google Scholar 

  9. MacLane, S.: Categories for the Working Mathematician, 2nd edn. Springer, Heidelberg (1998)

    Google Scholar 

  10. Simpson, A., Plotkin, G.: Complete axioms for categorical fixed-point operators. In: IEEE Symposium Logic in Computer Science, pp. 30–41 (1998)

    Google Scholar 

  11. Wright, J.B., Thatcher, J.W., Wagner, E.G., Goguen, J.A.: Rational algebraic theories and fixed-point solutions. In: Proc. 17th IEEE Symposium on Foundations of Computing, Houston, Texas, pp. 147–158. IEEE Computer Society Press, Los Alamitos (1976)

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institute of Theoretical Computer Science, TU Braunschweig, Germany

    Jiří Adámek & Stefan Milius

  2. Department of Mathematics, ČVUT Prague, Czech Republic

    Jiří Velebil

Authors
  1. Jiří Adámek
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Stefan Milius
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Jiří Velebil
    View author publications

    You can also search for this author in PubMed Google Scholar

Editor information

Luděk Kučera Antonín Kučera

Rights and permissions

Reprints and permissions

Copyright information

© 2007 Springer-Verlag Berlin Heidelberg

About this paper

Cite this paper

Adámek, J., Milius, S., Velebil, J. (2007). What Are Iteration Theories?. In: Kučera, L., Kučera, A. (eds) Mathematical Foundations of Computer Science 2007. MFCS 2007. Lecture Notes in Computer Science, vol 4708. Springer, Berlin, Heidelberg. https://doi.org/10.1007/978-3-540-74456-6_23

Download citation

  • DOI: https://doi.org/10.1007/978-3-540-74456-6_23

  • Publisher Name: Springer, Berlin, Heidelberg

  • Print ISBN: 978-3-540-74455-9

  • Online ISBN: 978-3-540-74456-6

  • eBook Packages: Computer ScienceComputer Science (R0)

Publish with us

Policies and ethics