Performance of an Energy Detector with Generalized Selection Combining for Spectrum Sensing

Abstract

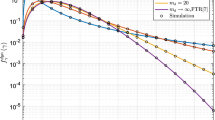
Diversity reception schemes are well-known to have the ability to mitigate the adverse effects of multipath wireless channels. This paper analyzes the performance of an energy detector with generalized selection combining (GSC) over a Rayleigh fading channel and compares the results with those of the conventional diversity combining schemes such as, maximal-ratio combining (MRC) and the selection combining (SC). Novel closed-form expressions have been derived for the average detection probability over the independently, identically distributed (i.i.d) diversity paths. Receiver operating characteristics (ROCs) and average detection probability versus SNR curves have been presented for different scenarios of interest.

Appendices

A Appendix

1.1 Evaluation of $A_{1}$ in (10)

Using [8, (5)], $A_{1}$ can be written as:

$$\begin{aligned} A_{1} = \left( {\begin{array}{c}L\\ L_{c}\end{array}}\right) \dfrac{1}{\overline{\gamma }^{L_{c}}(L_{c}-1)!}&\int _{0}^{\infty }\Bigg [ 1- \exp \left( -\dfrac{2\gamma _{\tiny {\text{ GSC }}} + \lambda }{2}\right) \sum _{n = N}^{\infty }\left( \dfrac{\sqrt{\lambda }}{\sqrt{2\gamma _{\tiny {\text{ GSC }}}}}\right) ^{n} \nonumber \\&\cdot I_{n}\left( \sqrt{2\gamma _{\tiny {\text{ GSC }}} \lambda }\right) \Bigg ] \gamma _{\tiny {\text{ GSC }}}^{L_{c}-1} \exp \left( -\dfrac{\gamma _{\tiny {\text{ GSC }}}}{\overline{\gamma }}\right) d\gamma _{\tiny {\text{ GSC }}} \end{aligned}$$

(16)

where, $I_{n}(\cdot )$ is the modified Bessel function of order n. Using transformation and change of variable, (16) can be written as:

$$\begin{aligned} A_{1} =&2\left( {\begin{array}{c}L\\ L_{c}\end{array}}\right) \dfrac{1}{\overline{\gamma }^{L_{c}}(L_{c}-1)!}\int _{0}^{\infty }Q_{N}\left( \sqrt{2}\gamma _{\tiny {\text{ GSC }}}, \sqrt{\lambda }\right) \gamma _{\tiny {\text{ GSC }}}^{\left( 2L_{c}-1\right) } \exp \left( -\dfrac{\gamma _{\tiny {\text{ GSC }}}^{2}}{\overline{\gamma }}\right) d\gamma _{\tiny {\text{ GSC }}} \nonumber \\ =&2\left( {\begin{array}{c}L\\ L_{c}\end{array}}\right) \dfrac{1}{\overline{\gamma }^{L_{c}}(L_{c}-1)!}\cdot G_{N} \end{aligned}$$

(17)

From [8, (29)], the above equation becomes equal to (13) where, $G_{1}$ can be defined as [8, (25)]:

$$\begin{aligned} G_{1} = \dfrac{2^{L_{c}-1}\left( L_{c}-1\right) !}{\left( 2/\overline{\gamma }\right) ^{L_{c}}}\left( \dfrac{\overline{\gamma }}{1+\overline{\gamma }}\right) \exp \left( -\dfrac{\lambda }{2\left( 1+\overline{\gamma }\right) }\right)&\sum _{k = 0}^{L_{c}-1} \epsilon _{k} \left( \dfrac{1}{1+\overline{\gamma }}\right) ^{k} \nonumber \\&\cdot L_{k}\left( -\dfrac{\lambda \overline{\gamma }}{2\left( 1+\overline{\gamma }\right) }\right) \end{aligned}$$

(18)

where,

$$\begin{aligned} \epsilon _{k}\equiv {\left\{ \begin{array}{ll} 1;&{} k<L_{c}-1\\ 1+\dfrac{1}{\overline{\gamma }};&{} k = L_{c}-1 \end{array}\right. } \end{aligned}$$

(19)

and $L_{k}(\cdot )$ is the Laguerre polynomial of degree k.

B Appendix

1.1 Evaluation of $A_{2}$ in (11)

From (11), $A_{2}$ can be given as:

$$\begin{aligned} A_{2} =&\left( {\begin{array}{c}L\\ L_{c}\end{array}}\right) \dfrac{1}{\overline{\gamma }}\sum _{l = 1}^{L - L_{c}}(-1)^{L_{c}-l+1}\left( {\begin{array}{c}L-L_{c}\\ l\end{array}}\right) \left( \dfrac{L_{c}}{l}\right) ^{L_{c}-1} \int _{0}^{\infty }\Bigg [ 1 - \exp \left( -\dfrac{2\gamma _{\tiny {\text{ GSC }}}+\lambda }{2}\right) \nonumber \\&\cdot \sum _{n= N}^{\infty }\left( \dfrac{\sqrt{\lambda }}{\sqrt{2\gamma }}\right) ^{n} I_{n}\left( \sqrt{2\gamma _{\tiny {\text{ GSC }}}\lambda }\right) \Bigg ] \exp \left[ -\dfrac{\gamma _{\tiny {\text{ GSC }}}}{\overline{\gamma }}\left( 1+\dfrac{l}{L_{c}}\right) \right] d\gamma _{\tiny {\text{ GSC }}} \nonumber \\ =&2 \left( {\begin{array}{c}L\\ L_{c}\end{array}}\right) \dfrac{1}{\overline{\gamma }}\sum _{l = 1}^{L - L_{c}}(-1)^{L_{c}-l+1}\left( {\begin{array}{c}L-L_{c}\\ l\end{array}}\right) \left( \dfrac{L_{c}}{l}\right) ^{L_{c}-1} \int _{0}^{\infty }Q_{N}\left( \sqrt{2}\gamma _{\tiny {\text{ GSC }}}, \sqrt{\lambda }\right) \nonumber \\&\cdot \exp \left[ -\dfrac{\gamma _{\tiny {\text{ GSC }}}^{2}}{\overline{\gamma }}\left( 1+\dfrac{l}{L_{c}}\right) \right] \gamma _{\tiny {\text{ GSC }}} d\gamma _{\tiny {\text{ GSC }}} \nonumber \\ =&2 \left( {\begin{array}{c}L\\ L_{c}\end{array}}\right) \dfrac{1}{\overline{\gamma }}\sum _{l = 1}^{L - L_{c}}(-1)^{L_{c}-l+1}\left( {\begin{array}{c}L-L_{c}\\ l\end{array}}\right) \left( \dfrac{L_{c}}{l}\right) ^{L_{c}-1}\cdot D_{N} \end{aligned}$$

(20)

From [8, (29)], the above equation becomes equal to (14) where, $D_{1}$ can be defined as [8, (25)]:

$$\begin{aligned} D_{1} =&\dfrac{\left( \overline{\gamma }L_{c}\right) ^{2}}{2\left( l+Lc\right) \left( l+L_{c}+\overline{\gamma }L_{c}\right) } \exp \left( -\dfrac{\lambda \left( l+L_{c}\right) }{2\left( l+L_{c}+\overline{\gamma }L_{c}\right) }\right) \cdot \Bigg [\left( 1+\dfrac{l+L_{c}}{\overline{\gamma }L_{c}}\right) \Bigg ] \end{aligned}$$

(21)

In the above equation, it is important to note that the value of Laguerre polynomial for order 0 becomes 1.

C Appendix

1.1 Evaluation of $A_{3}$ in (12)

Following the same analogy as in Appendix A, (12) can be written as:

$$\begin{aligned} A_{3} =&-2 \left( {\begin{array}{c}L\\ L_{c}\end{array}}\right) \dfrac{1}{\overline{\gamma }}\sum _{l = 1}^{L - L_{c}}(-1)^{L_{c}-l+1} \left( {\begin{array}{c}L-L_{c}\\ l\end{array}}\right) \left( \dfrac{L_{c}}{l}\right) ^{L_{c}-1} \cdot \sum _{m = 0}^{L_{c}-2}\dfrac{1}{m!}\left( \dfrac{-l}{L_{c}\overline{\gamma }}\right) ^{m} \nonumber \\&\cdot \int _{0}^{\infty }Q_{N}\left( \sqrt{2}\gamma _{\tiny {\text{ GSC }}},\sqrt{\lambda }\right) \exp \left( -\gamma _{\tiny {\text{ GSC }}}^{2}/\overline{\gamma }\right) \gamma _{\tiny {\text{ GSC }}}^{2m+1}d\gamma _{\tiny {\text{ GSC }}} \nonumber \\ =&-2 \left( {\begin{array}{c}L\\ L_{c}\end{array}}\right) \dfrac{1}{\overline{\gamma }}\sum _{l = 1}^{L - L_{c}}(-1)^{L_{c}-l+1} \left( {\begin{array}{c}L-L_{c}\\ l\end{array}}\right) \left( \dfrac{L_{c}}{l}\right) ^{L_{c}-1} \cdot \sum _{m = 0}^{L_{c}-2}\dfrac{1}{m!}\left( \dfrac{-l}{L_{c}\overline{\gamma }}\right) ^{m} \cdot J_{N} \end{aligned}$$

(22)

From [8, (29)], the above equation becomes equal to (15) where, $J_{1}$ can be defined as [8, (25)]:

$$\begin{aligned} J_{1} =&\dfrac{2^{m}m!}{\left( 2/\overline{\gamma }\right) ^{(m+1)}}\dfrac{\overline{\gamma }}{1+\overline{\gamma }}\exp \left( -\dfrac{\lambda }{2\left( 1+\overline{\gamma }\right) }\right) \sum _{k = 0}^{m}\phi _{k}\left( \dfrac{1}{1+\overline{\gamma }}\right) ^{k}L_{k}\left( -\dfrac{\lambda \overline{\gamma }}{2\left( 1+\overline{\gamma }\right) }\right) \end{aligned}$$

(23)

where,

$$\begin{aligned} \phi _{k}\equiv {\left\{ \begin{array}{ll} 1;&{} k<m\\ 1+\dfrac{1}{\overline{\gamma }};&{} k = m \end{array}\right. } \end{aligned}$$

(24)