Abstract
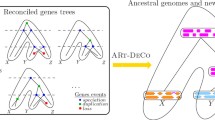
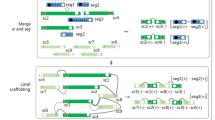
Ancestral genome reconstruction is an important step in analyzing the evolution of genomes. Recent progress in sequencing ancient DNA led to the publication of so-called paleogenomes and allows the integration of this sequencing data in genome evolution analysis. However, the assembly of ancient genomes is fragmented because of DNA degradation over time. Integrated phylogenetic assembly addresses the issue of genome fragmentation in the ancient DNA assembly while improving the reconstruction of all ancient genomes in the phylogeny. The fragmented assembly of the ancient genome can be represented as an assembly graph, indicating contradicting ordering information of contigs.
In this setting, our approach is to compare the ancient data with extant finished genomes. We generalize a reconstruction approach minimizing the Single-Cut-or-Join rearrangement distance towards multifurcating trees and include edge lengths to avoid a sparse reconstruction in practice. When also including the additional conflicting ancient DNA data, we can still ensure consistent reconstructed genomes.
This is a preview of subscription content, log in via an institution to check access.
Access this chapter
Tax calculation will be finalised at checkout
Purchases are for personal use only
Preview
Unable to display preview. Download preview PDF.
Similar content being viewed by others
References
Bergeron, A., Blanchette, M., Chateau, A., Chauve, C.: Reconstructing ancestral gene orders using conserved intervals. In: Jonassen, I., Kim, J. (eds.) WABI 2004. LNCS (LNBI), vol. 3240, pp. 14–25. Springer, Heidelberg (2004)
Bertrand, D., Gagnon, Y., Blanchette, M., El-Mabrouk, N.: Reconstruction of ancestral genome subject to whole genome duplication, speciation, rearrangement and loss. In: Moulton, V., Singh, M. (eds.) WABI 2010. LNCS (LNBI), vol. 6293, pp. 78–89. Springer, Heidelberg (2010)
Bos, K.I., Schuenemann, V.J., Golding, G.B., et al.: A draft genome of yersinia pestis from victims of the black death. Nature 478(7370), 506–510 (2011)
Chauve, C., Tannier, E.: A methodological framework for the reconstruction of contiguous regions of ancestral genomes and its application to mammalian genomes. PLoS Computational Biology 4(11), e1000234 (2008)
Drancourt, M., Raoult, D.: Palaemicrobiology: current issues and perspectives. Nature Rev. Microbiol. 3, 23–35 (2005)
Feijão, P., Meidanis, J.: SCJ: a breakpoint-like distance that simplifies several rearrangement problems. IEEE/ACM Trans. Comput. Biol. and Bioinf. 8(5), 1318–1329 (2011)
Fitch, W.M.: Toward defining the course of evolution: minimum change for a specific tree topology. Systematic Biology 20(4), 406–416 (1971)
Hartigan, J.A.: Minimum mutation fits to a given tree. Biometrics, 53–65 (1973)
Ma, J., Zhang, L., Suh, B.B., et al.: Reconstructing contiguous regions of an ancestral genome. Genome Res. 16(12), 1557–1565 (2006)
Maňuch, J., Patterson, M., Wittler, R., Chauve, C., Tannier, E.: Linearization of ancestral multichromosomal genomes. BMC Bioinformatics 13(19), S11 (2012)
Rajaraman, A., Tannier, E., Chauve, C.: FPSAC: fast phylogenetic scaffolding of ancient contigs. Bioinformatics 29(23), 2987–2994 (2013)
Stoye, J., Wittler, R.: A unified approach for reconstructing ancient gene clusters. IEEE/ACM Trans. Comput. Biol. Bioinf. 6(3), 387–400 (2009)
Zheng, C., Sankoff, D.: On the pathgroups approach to rapid small phylogeny. BMC Bioinformatics 12(S-1), S4 (2011)
Author information
Authors and Affiliations
Editor information
Editors and Affiliations
Rights and permissions
Copyright information
© 2014 Springer International Publishing Switzerland
About this paper
Cite this paper
Luhmann, N., Chauve, C., Stoye, J., Wittler, R. (2014). Scaffolding of Ancient Contigs and Ancestral Reconstruction in a Phylogenetic Framework. In: Campos, S. (eds) Advances in Bioinformatics and Computational Biology. BSB 2014. Lecture Notes in Computer Science(), vol 8826. Springer, Cham. https://doi.org/10.1007/978-3-319-12418-6_17
Download citation
DOI: https://doi.org/10.1007/978-3-319-12418-6_17
Publisher Name: Springer, Cham
Print ISBN: 978-3-319-12417-9
Online ISBN: 978-3-319-12418-6
eBook Packages: Computer ScienceComputer Science (R0)