Optimal Proof Systems and Sparse Sets | SpringerLink
Skip to main content
Springer Nature Link
Log in

Optimal Proof Systems and Sparse Sets

  • Conference paper
  • First Online:
STACS 2000 (STACS 2000)

Part of the book series: Lecture Notes in Computer Science ((LNCS,volume 1770))

Included in the following conference series:

  • 888 Accesses

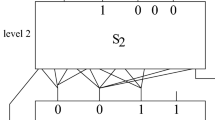
Abstract

We exhibit a relativized world where NP ∩ SPARSE has no complete sets. This gives the first relativized world where no optimal proof systems exist.

We also examine under what reductions NP ∩ SPARSE can have complete sets. We show a close connection between these issues and reductions from sparse to tally sets. We also consider the question as to whether the NP ∩ SPARSE languages have a computable enumeration.

Several proofs have been omitted to conserve space. A full version can be found at http://www.neci.nj.nec.com/homepages/fortnow/papers.

Supported in part by NSF grants CCR-9501794 and CCR-9996310.

Supported in part by NSF grant CCR-9732922.

Supported in part by NSF grant CCR-9732922.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Chapter
JPY 3498
Price includes VAT (Japan)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever
eBook
JPY 11439
Price includes VAT (Japan)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever
Softcover Book
JPY 14299
Price includes VAT (Japan)
  • Compact, lightweight edition
  • Dispatched in 3 to 5 business days
  • Free shipping worldwide - see info

Tax calculation will be finalised at checkout

Purchases are for personal use only

Institutional subscriptions

Preview

Unable to display preview. Download preview PDF.

Unable to display preview. Download preview PDF.

Similar content being viewed by others

References

  1. H. Buhrman, E. Hemaspaandra, and L. Longpré. SPARSE reduces conjunctively to TALLY. SIAM Journal on Computing, 24(3):673–681, 1995.

    Article  MATH  MathSciNet  Google Scholar 

  2. R. Book and K. Ko. On sets truth-table reducible to sparse sets. SIAM Journal on Computing, 17(5):903–919, 1988.

    Article  MATH  MathSciNet  Google Scholar 

  3. S. Cook and R. Reckhow. The relative effciency of propositional proof systems. Journal of Symbolic Logic, 44:36–50, 1979.

    Article  MATH  MathSciNet  Google Scholar 

  4. L. Fortnow. The role of relativization in complexity theory. Bulletin of the European Association for Theoretical Computer Science, 52:229–244, February 1994.

    MATH  Google Scholar 

  5. J. Hartmanis and L. Hemachandra. Complexity classes without machines: On complete languages for UP. Theoretical Computer Science, 34:17–32, 1984.

    Article  MATH  MathSciNet  Google Scholar 

  6. L. Hemaspaandra, S. Jain, and N. Vereshchagin. Banishing robust turing completeness. International Journal of Foundations of Computer Science, 4(3):245–265, 1993.

    Article  MATH  MathSciNet  Google Scholar 

  7. J. Hartmanis and Y. Yesha. Computation times of NP sets of different densities. Theoretical Computer Science, 34(1–2):17–32, November 1984.

    Article  MATH  MathSciNet  Google Scholar 

  8. K. Ko. Distinguishing conjunctive and disjunctive reducibilities by sparse sets. Information and Computation, 81(1):62–87, 1989.

    Article  MATH  MathSciNet  Google Scholar 

  9. J. Krajíček and P. Pudlák. Propositional proof systems, the consistency of first order theories and the complexity of computations. Journal of Symbolic Logic, 54:1063–1079, 1989.

    Article  MATH  MathSciNet  Google Scholar 

  10. A. Klivans and D. van Melkebeek. Graph nonisomorhism has subexponential size proofs unless the polynomial-time hierarchy collapses. In Proceedings of the 31st ACM Symposium on the Theory of Computing, pages 659–667. ACM, New York, 1999.

    Google Scholar 

  11. M. Li and P. Vitányi. An Introduction to Kolmogorov Complexity and Its Applications. Graduate Texts in Computer Science. Springer, New York, second edition, 1997.

    MATH  Google Scholar 

  12. J. Messner and J. Torán. Optimal proof systems for propositional logic and complete sets. In Proceedings of the 15th Symposium on Theoretical Aspects of Computer Science, volume 1373 of Lecture Notes in Computer Science, pages 477–487. Springer, 1998.

    Google Scholar 

  13. S. Saluja. Relativized limitations of left set technique and closure classes of sparse sets. In Proceedings of the 8th IEEE Structure in Complexity Theory Conference, pages 215–223. IEEE, New York, 1993.

    Google Scholar 

  14. U. Schöning. On random reductions from sparse sets to tally sets. Information Processing Letters, 46(5):239–241, July 1993.

    Article  MATH  Google Scholar 

  15. J. Seiferas, M. Fischer, and A. Meyer. Separating nondeterministic time complexity classes. Journal of the ACM, 25(1):146–167, 1978.

    Article  MATH  MathSciNet  Google Scholar 

  16. S. Žàk. A Turing machine time hierarchy. Theoretical Computer Science, 26(3):327–333, 1983.

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Author notes

  1. Lance Fortnow

    Present address: NEC Research, 4 Independence Way, Princeton, NJ, 08540

  2. Dieter van Melkebeek

    Present address: DIMACS Center, Rutgers University, 96 Frelinghuysen Road, Piscataway, NJ, 08854

Authors and Affiliations

  1. CWI, INS4, P.O. Box 94079, 1090 GB, Amsterdam, The Netherlands

    Harry Buhrman

  2. Department of Computer Science, The University of South Carolina, Columbia, SC, 29208

    Steve Fenner

  3. University of Chicago, Chicago

    Lance Fortnow

  4. University of Chicago and DIMACS, Chicago

    Dieter van Melkebeek

Authors
  1. Harry Buhrman
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Steve Fenner
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Lance Fortnow
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Dieter van Melkebeek
    View author publications

    You can also search for this author in PubMed Google Scholar

Editor information

Editors and Affiliations

  1. Faculty of Computer Science, Dept. of Theoretical Computer Science, Universität Dresden, 01062, Dresden, Germany

    Horst Reichel

  2. Université de Lille, LIFL - UPRESA 8022, CNRS, Bât. M3 - UFR IEEA, 59655, Villeneuve d’ASCQ Cedex, France

    Sophie Tison

Rights and permissions

Reprints and permissions

Copyright information

© 2000 Springer-Verlag Berlin Heidelberg

About this paper

Cite this paper

Buhrman, H., Fenner, S., Fortnow, L., van Melkebeek, D. (2000). Optimal Proof Systems and Sparse Sets. In: Reichel, H., Tison, S. (eds) STACS 2000. STACS 2000. Lecture Notes in Computer Science, vol 1770. Springer, Berlin, Heidelberg. https://doi.org/10.1007/3-540-46541-3_34

Download citation

  • DOI: https://doi.org/10.1007/3-540-46541-3_34

  • Published:

  • Publisher Name: Springer, Berlin, Heidelberg

  • Print ISBN: 978-3-540-67141-1

  • Online ISBN: 978-3-540-46541-6

  • eBook Packages: Springer Book Archive

Publish with us

Policies and ethics