Multi-joint Arm Trajectory Formation Based on the Minimization Principle Using the Euler-Poisson Equation

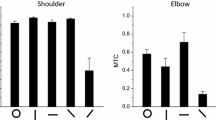
Abstract

In previous research, criteria based on optimal theories were examined to explain trajectory features in time and space in multi joint arm movements. Four criteria have been proposed. They were the minimum hand jerk criterion, the minimum angle jerk criterion, the minimum torque change criterion, and the minimum commanded torque change criterion. Optimal trajectories based on the two former criteria can be calculated analytically. In contrast, optimal trajectories based on the minimum commanded torque change criterion are difficult to be calculated even with numerical methods. In some cases, they can be computed by a Newton-like method or a steepest descent method combined with a penalty method. However, for a realistic physical parameter range, a former becomes unstable quite often, and the latter is unreliable about the optimality of the obtained solution. In this paper, we propose a new method to stably calculate optimal trajectories based on the minimum commanded torque change criterion. The method can obtain trajectories satisfying Euler-Poisson equations with a sufficiently high accuracy. In the method, a joint angle trajectory, which satisfies the boundary conditions strictly, is expressed by using orthogonal polynomials. The coefficients of the orthogonal polynomials are estimated by using a linear iterative calculation so as to satisfy the Euler-Poisson equations with a sufficiently high accuracy. In numerical experiments, we show that the optimal solution can be computed in a wide work space and can also be obtained in a short time compared with the previous methods.