Geometric Invariant Domain for Image Watermarking | SpringerLink
Skip to main content
Springer Nature Link
Log in

Geometric Invariant Domain for Image Watermarking

  • Conference paper
Digital Watermarking (IWDW 2006)

Part of the book series: Lecture Notes in Computer Science ((LNSC,volume 4283))

Included in the following conference series:

Abstract

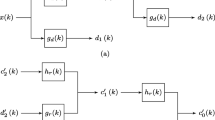
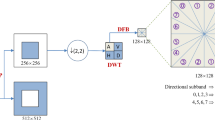
To enable copyright protection and authentication, robust digital watermark can be embedded into multimedia contents imperceptibly. However, geometric distortions pose a significant threat to robust image watermarking because it can desynchronize the watermark information while preserving the visual quality. To overcome this, we developed an invariant domain with three transforms; Fast Fourier Transform (FFT), Log-Polar Mapping (LPM), and Dual Tree-Complex Wavelet Transform (DT-CWT). Shift invariance is obtained using FFT. Rotation and scaling invariance are achieved by taking the DT-CWT of a LPM output. Unlike most invariant schemes, our method eliminates explicit re-synchronization. The method resists geometric distortions at both global and local scales. It is also robust against JPEG compression and common image processing. In addition, it exploits perceptual masking property of the DT-CWT subbands, and its watermark detection step does not require the cover image. Experiment on a large set of natural images shows the robustness of the new scheme.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Institutional subscriptions

Preview

Unable to display preview. Download preview PDF.

Unable to display preview. Download preview PDF.

Similar content being viewed by others

References

  1. Bas, P., Chassery, J.-M., Macq, B.: Geometrically invariant watermarking using feature points. IEEE Transactions on Image Processing 11(9), 1014–1028 (2002)

    Article  Google Scholar 

  2. Pawlak, M., Xin, Y.: Robust image watermarking: an invariant domain approach. In: Canadian Conference on Electrical and Computer Engineering, 2002. IEEE CCECE 2002, vol. 2, pp. 885–888 (2002)

    Google Scholar 

  3. Ruanaidh, J.J.K.O., Pun, T.: Rotation, scale and translation invariant spread spectrum digital image watermarking. Signal Processing 66(3), 303–317 (1998)

    Article  MATH  Google Scholar 

  4. Lin, C.-Y., et al.: Rotation, scale, and translation resilient watermarking for images. IEEE Transactions on Image Processing 10(5), 767–782 (2001)

    Article  MATH  Google Scholar 

  5. Zheng, D., Zhao, J.: RST invariant digital image watermarking based on resynchronization. In: Canadian Conference on Electrical and Computer Engineering, vol. 3, pp. 1281–1284 (2004)

    Google Scholar 

  6. Zheng, D., Liu, Y., Zhao, J.: RST invariant digital image watermarking based on a new phase-only filtering method. In: Proceedings of 7th International Conference on Signal Processing, 2004. ICSP 2004, vol. 1, pp. 25–28 (2004)

    Google Scholar 

  7. Liu, Y., Zhao, J.: A new filtering method for RST invariant image watermarking. In: Proceedings of the 2nd IEEE Internatioal Workshop on Haptic, Audio and Visual Environments and Their Applications, 2003. HAVE 2003, pp. 101–106 (2003)

    Google Scholar 

  8. Xuan, J., Zhang, H., Wang, L.: Rotation, scaling and translation invariant image watermarking based on Radon transform. In: Visual Communications and Image Processing 2005. 2005: SPIE–The International Society for Optical Engineering, pp. 1499–1505 (2005)

    Google Scholar 

  9. Kim, B.-S., et al.: Robust digital image watermarking method against geometrical attacks. Real-Time Imaging 9(2), 139–149 (2003)

    Article  Google Scholar 

  10. Kim, B.-S., Choi, J.-G., Park, K.-H.: RST-Resistant Image Watermarking Using Invariant Centroid and Reordered Fourier-Mellin Transform. In: Kalker, T., Cox, I., Ro, Y.M. (eds.) IWDW 2003. LNCS, vol. 2939, pp. 370–381. Springer, Heidelberg (2004)

    Chapter  Google Scholar 

  11. Kim, H.S., Lee, H.-K.: Invariant image watermark using Zernike moments. IEEE Transactions on Circuits and Systems for Video Technology 13(8), 766–775 (2003)

    Article  Google Scholar 

  12. Kingsbury, N.G.: Complex wavelets for shift invariant analysis and filtering of signals. Journal of Applied and Computational Harmonic Analysis 10(3), 234–253 (2001)

    Article  MATH  MathSciNet  Google Scholar 

  13. Selesnick, I.W., Baraniuk, R.G., Kingsbury, N.C.: The dual-tree complex wavelet transform. Signal Processing Magazine, IEEE 22(6), 123–151 (2005)

    Article  Google Scholar 

  14. Loo, P., Kingsbury, N.G.: Motion estimation based registration of geometrically distorted images for watermark recovery. In: SPIE Conference on Security and Watermarking of Multimedia Contents III. SPIE, San Diego, USA, pp. 606–617 (2001)

    Google Scholar 

  15. Day, M.-L., Lee, S.-Y., Jou, I.-C.: Watermark Re-synchronization Using Sinusoidal Signals in DT-CWT Domain. In: Aizawa, K., Nakamura, Y., Satoh, S. (eds.) PCM 2004. LNCS, vol. 3333, pp. 394–401. Springer, Heidelberg (2004)

    Chapter  Google Scholar 

  16. Serdean, C., et al.: Protecting Intellectual Rights: Digital Watermark in the Wavelet Domain. In: IEEE Int. Workshop on Trends and Recent Achievements in IT, Cluj-Napoca, Romania (2002)

    Google Scholar 

  17. Kingsbury, N.: Shift invariant properties of the dual-tree complex wavelet transform. In: 1999 IEEE International Conference on Acoustics, Speech, and Signal Processing. ICASSP 1999. Proceedings, Phoenix, Arizona, USA, pp. 1221–1224 (1999)

    Google Scholar 

  18. Terzija, N., Geisselhardt, W.: Digital image watermarking using complex wavelet transform. In: Proceedings of the 2004 workshop on Multimedia and security, International Multimedia Conference, pp. 193–198. ACM Press, Magdeburg (2004)

    Chapter  Google Scholar 

  19. Barni, M., Bartolini, F., Piva, A.: Improved wavelet-based watermarking through pixel-wise masking. IEEE Transactions on Image Processing 10(5), 783–791 (2001)

    Article  MATH  Google Scholar 

  20. Woo, C.-S., Du, J., Pham, B.: Performance Factors Analysis of a Wavelet-based Watermarking Method. In: The Third Australasian Information Security Workshop (AISW 2005), pp. 89–97. Australian Computer Society, Newcastle, NSW, Australia (2005)

    Google Scholar 

  21. Cox, I., Miller, M.L., Bloom, J.A.: Digital watermarking, p. 539. Morgan Kaufmann Publishers Inc., San Francisco (2001)

    Google Scholar 

  22. Petitcolas, F.A.P.: Watermarking schemes evaluation. Signal Processing Magazine 17(5), 58–64 (2000)

    Article  Google Scholar 

  23. Petitcolas, F.A.P., Anderson, R.J., Kuhn, M.G.: Attacks on Copyright Marking Systems. In: Aucsmith, D. (ed.) IH 1998. LNCS, vol. 1525, pp. 218–239. Springer, Heidelberg (1998)

    Chapter  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Information Security Institute (ISI), Faculty of Information Technology, Queensland University of Technology, GPO Box 2434, Brisbane, QLD4001, Australia

    Chaw-Seng Woo & Jiang Du

  2. Faculty of Information Technology, Queensland University of Technology, GPO Box 2434, Brisbane, QLD4001, Australia

    Binh Pham

Authors
  1. Chaw-Seng Woo
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jiang Du
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Binh Pham
    View author publications

    You can also search for this author in PubMed Google Scholar

Editor information

Editors and Affiliations

  1. New Jersey Institute of Technology,, Newark, New Jersey, USA

    Yun Qing Shi

  2. Sung Kyun Kwan University, 300 Chunchun-dong, Jangan-gu, Suwon, Korea

    Byeungwoo Jeon

Rights and permissions

Reprints and permissions

Copyright information

© 2006 Springer-Verlag Berlin Heidelberg

About this paper

Cite this paper

Woo, CS., Du, J., Pham, B. (2006). Geometric Invariant Domain for Image Watermarking. In: Shi, Y.Q., Jeon, B. (eds) Digital Watermarking. IWDW 2006. Lecture Notes in Computer Science, vol 4283. Springer, Berlin, Heidelberg. https://doi.org/10.1007/11922841_24

Download citation

  • DOI: https://doi.org/10.1007/11922841_24

  • Publisher Name: Springer, Berlin, Heidelberg

  • Print ISBN: 978-3-540-48825-5

  • Online ISBN: 978-3-540-48827-9

  • eBook Packages: Computer ScienceComputer Science (R0)

Publish with us

Policies and ethics

Search

Navigation