Self-stabilizing Checkpointing Algorithm in Ring Topology | SpringerLink
Skip to main content
Springer Nature Link
Log in

Self-stabilizing Checkpointing Algorithm in Ring Topology

  • Conference paper
Distributed Computing – IWDC 2005 (IWDC 2005)

Part of the book series: Lecture Notes in Computer Science ((LNTCS,volume 3741))

Included in the following conference series:

Abstract

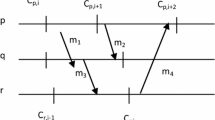
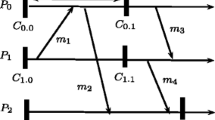
If the variables used for a checkpointing algorithm have data faults, the algorithm may fail. In this paper, a self-stabilizing checkpointing algorithm is proposed for handling data faults in a ring network. The proposed algorithm can deal with concurrent initiations of checkpointing and at most one data fault per process. However, several processes may be faulty.

This is a preview of subscription content, log in via an institution to check access.

Access this chapter

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Chapter
JPY 3498
Price includes VAT (Japan)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever
eBook
JPY 11439
Price includes VAT (Japan)
  • Available as PDF
  • Read on any device
  • Instant download
  • Own it forever
Softcover Book
JPY 14299
Price includes VAT (Japan)
  • Compact, lightweight edition
  • Dispatched in 3 to 5 business days
  • Free shipping worldwide - see info

Tax calculation will be finalised at checkout

Purchases are for personal use only

Institutional subscriptions

Preview

Unable to display preview. Download preview PDF.

Unable to display preview. Download preview PDF.

Similar content being viewed by others

References

  1. Dijkstra, E.W.: Self stabilizing systems in spite of distributed control. Communications of the ACM 17, 643–644 (1974)

    Article  MATH  Google Scholar 

  2. Ghosh, S., He, X.: Scalable Self-Stabilization. Journal of Parallel and Distributed Computing 62(5), 945–960 (2002)

    Article  MATH  Google Scholar 

  3. Ghosh, S., Gupta, A., Herman, T., Pemmaraju, S.V.: Fault-containing self-stabilizing algorithms. In: Proc. 15th ACM Symp. Princ. of Distrib. Comput., pp. 45–54 (1996)

    Google Scholar 

  4. Schneider, M.: Self-Stabilization. ACM Computing Surveys 25(1), 45–67 (1993)

    Article  Google Scholar 

  5. Chandy, K.M., Lamport, L.: Distributed snapshots: Determining global states of distributed systems. ACM Trans. Comput. Syst. 3(1), 63–75 (1985)

    Article  Google Scholar 

  6. Manivannan, D., Singhal, M.: Quasi-synchronous checkpointing: Models, characterization, and classification. IEEE Trans. on Parallel and Distributed Systems 10(7), 703–713 (1999)

    Article  Google Scholar 

  7. Vidya, N.H.: Staggered consistent checkpointing. IEEE Trans. on Parallel and Distributed Systems 10(7), 694–702 (1999)

    Article  Google Scholar 

  8. Mandal, P.S., Mukhopadhyaya, K.: Concurrent checkpoint initiation and recovery algorithms on asynchronous ring networks. Journal of Parallel and Distributed Computing 64(5), 649–661 (2004)

    Article  MATH  Google Scholar 

  9. Mandal, P.S., Mukhopadhyaya, K.: Self-Stabilizing checkpointing algorithm in ring topology, TR: ACMU/2005/01. Indian Statistical Institute, Kolkata (2005)

    Google Scholar 

  10. Spezialetti, M., Kearns, P.: Efficient distributed snapshots. In: Proc. 6th International Conference on Distributed Computing Systems, pp. 382–388 (1986)

    Google Scholar 

  11. Prakash, R., Singhal, M.: Maximal global snapshot with concurrent initiators. In: Proc. 6th IEEE Symp. Parallel and Distrib, October 1994, pp. 334–351 (1994)

    Google Scholar 

  12. Manivannan, D., Singhal, M.: Asynchronous recovery without using vector timestamps. J. Parallel Distrib. Comput. 62(12), 1695–1728 (2002)

    Article  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Advanced Computing and Microelectronics Unit, Indian Statistical Institute, 203, B T Road, Kolkata, 700108, India

    Partha Sarathi Mandal & Krishnendu Mukhopadhyaya

Authors
  1. Partha Sarathi Mandal
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Krishnendu Mukhopadhyaya
    View author publications

    You can also search for this author in PubMed Google Scholar

Editor information

Editors and Affiliations

  1. Department of Computer Science and Engineering, Indian Institute of Technology, Kharagpur, West Bengal, India

    Ajit Pal

  2. Department of Computer Science, University of Illinois at Chicago, 60607, Chicago, Illinois

    Ajay D. Kshemkalyani

  3. Computer Science and Engineering Department, Indian Institute of Technology, 721 302, Kharagpur, WB, India

    Rajeev Kumar

  4. Department of Computer Science and Engineering, Indian Institute of Technology, 721 302, Kharagpur, India

    Arobinda Gupta

Rights and permissions

Reprints and permissions

Copyright information

© 2005 Springer-Verlag Berlin Heidelberg

About this paper

Cite this paper

Mandal, P.S., Mukhopadhyaya, K. (2005). Self-stabilizing Checkpointing Algorithm in Ring Topology. In: Pal, A., Kshemkalyani, A.D., Kumar, R., Gupta, A. (eds) Distributed Computing – IWDC 2005. IWDC 2005. Lecture Notes in Computer Science, vol 3741. Springer, Berlin, Heidelberg. https://doi.org/10.1007/11603771_16

Download citation

  • DOI: https://doi.org/10.1007/11603771_16

  • Publisher Name: Springer, Berlin, Heidelberg

  • Print ISBN: 978-3-540-30959-8

  • Online ISBN: 978-3-540-32428-7

  • eBook Packages: Computer ScienceComputer Science (R0)

Publish with us

Policies and ethics

Search

Navigation