Repeatability of Linear and Nonlinear Elastic Modulus Maps From Repeat Scans in the Breast - PubMed Skip to main page content
U.S. flag

An official website of the United States government

Dot gov

The .gov means it’s official.
Federal government websites often end in .gov or .mil. Before sharing sensitive information, make sure you’re on a federal government site.

Https

The site is secure.
The https:// ensures that you are connecting to the official website and that any information you provide is encrypted and transmitted securely.

Access keys NCBI Homepage MyNCBI Homepage Main Content Main Navigation
. 2021 Feb;40(2):748-757.
doi: 10.1109/TMI.2020.3036032. Epub 2021 Feb 2.

Repeatability of Linear and Nonlinear Elastic Modulus Maps From Repeat Scans in the Breast

Daniel I Gendin, Rohit Nayak, Yuqi Wang, Mahdi Bayat, Robert T Fazzio, Assad A Oberai, Timothy J Hall, Paul E Barbone, Azra Alizad, Mostafa Fatemi

Repeatability of Linear and Nonlinear Elastic Modulus Maps From Repeat Scans in the Breast

Daniel I Gendin et al. IEEE Trans Med Imaging. 2021 Feb.

Abstract

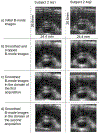
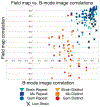
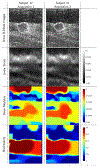
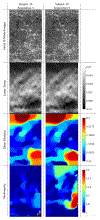
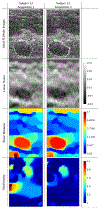
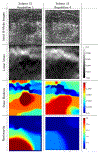
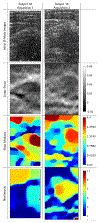
Compression elastography allows the precise measurement of large deformations of soft tissue in vivo. From an image sequence showing tissue undergoing large deformation, an inverse problem for both the linear and nonlinear elastic moduli distributions can be solved. As part of a larger clinical study to evaluate nonlinear elastic modulus maps (NEMs) in breast cancer, we evaluate the repeatability of linear and nonlinear modulus maps from repeat measurements. Within the cohort of subjects scanned to date, 20 had repeat scans. These repeated scans were processed to evaluate NEM repeatability. In vivo data were acquired by a custom-built, digitally controlled, uniaxial compression device with force feedback from the pressure-plate. RF-data were acquired using plane-wave imaging, at a frame-rate of 200 Hz, with a ramp-and-hold compressive force of 8N, applied at 8N/sec. A 2D block-matching algorithm was used to obtain sample-level displacement fields which were then tracked at subsample resolution using 2D cross correlation. Linear and nonlinear elasticity parameters in a modified Veronda-Westmann model of tissue elasticity were estimated using an iterative optimization method. For the repeated scans, B-mode images, strain images, and linear and nonlinear elastic modulus maps are measured and compared. Results indicate that when images are acquired in the same region of tissue and sufficiently high strain is used to recover nonlinearity parameters, then the reconstructed modulus maps are consistent.

PubMed Disclaimer

Figures

Fig. 1:
Fig. 1:
The process of registering B-mode images for acquisitions 1 and 2 of subject 2. a) The initial B-mode images for acquisitions 1 and 2 respectively. b) The initial B-mode images smoothed to avoid registering speckle noise and cropped to avoid edge artifacts from the smoothing. c) The smoothed B-mode image for acquisition 1 is reproduced from line (b); the smoothed B-mode image for acquisition 2 is shifted to be in the domain of acquisition 1. d) The smoothed B-mode image for acquisition 2 is reproduced from line (b); the smoothed B-mode image for acquisition 1 is shifted to be in the domain of acquisition 2.
Fig. 2:
Fig. 2:
Comparison of correlations between initial B-mode images, strain fields, shear modulus maps, and elastic nonlinearity maps, for both repeat acquisitions (blue markers), and those between distinct subjects (orange markers).
Fig. 3:
Fig. 3:
B-mode images, strain images, linear elastic shear modulus images, and elastic nonlinearity parameter images for subject 12. (ROI: 24 × 28mm) This panel shows excellent agreement between B-mode images (correlation 0.966), which suggests that the same ROIs were well targeted in both acquisitions. The correlations between the strain images (0.853) and between the shear modulus images (0.933) were correspondingly high. Furthermore sufficiently high strain (≥ 14%) was available to reliably reconstruct the elastic nonlinearity, which also shows excellent agreement (correlation 0.988). All color bars are dimensionless; strain and nonlinearity parameters are shown in absolute terms; linear shear modulus is normalized to unit geometric mean.
Fig. 4:
Fig. 4:
B-mode images and reconstructions for repeat acquisitions of subject 10, (ROI: 22 × 36mm) showing strong agreement between B-mode echo data acquisitions (correlation 0.934), between linear strain fields (0.935), and between linear elastic shear modulus reconstructions (0.976). A low level of maximum frame-average strain, however, gave nonlinearity parameters that were quite different (correlation 0.359).
Fig. 5:
Fig. 5:
B-mode images and reconstructions for repeat acquisitions from subject 13 (ROI: 21 × 31mm) This panel shows excellent agreement between B-mode images (correlation 0.967), which suggests that the same ROIs were well targeted in both acquisitions. The correlations between the strain images (0.828) and shear modulus images (0.956) are correspondingly high. The correlation between elastic nonlinearity reconstructions, however, was relatively low (0.528), mostly likely due to an insufficiently high final strain to recover nonlinearity well.
Fig. 6:
Fig. 6:
B-mode images and reconstructions for repeat acquisitions of subject 15 (ROI: 23 × 24mm) showing relatively poor agreement between the acquisitions (B-mode image correlation 0.665). The correlations for the strain (0.735), linear shear modulus (0.848), and nonlinearity parameter (0.785) images show moderately good agreement, despite a low level of maximum frame-average strain.
Fig. 7:
Fig. 7:
B-mode images, strain images, linear elastic shear modulus images, and elastic nonlinearity parameter images for repeat acquisitions of subject 18 showing exceptionally poor agreement between the acquisitions. In this example, the original ROI was poorly targeted in the repeat acquisition, and so all four image types show large differences. Correlations between repeat acquisitions are 0.704, 0.259, 0.415, −0.155, between repeated acquisition of B-mode images, strain images, shear modulus images, and elastic nonlinearity parameter images. We note in particular that the nonlinearity maps are negatively correlated.
See this image and copyright information in PMC

Similar articles

See all similar articles

Cited by

References

    1. Qiu Y, Sridhar M, Tsou JK, Lindfors KK, and Insana MF, “Ultrasonic viscoelasticity imaging of nonpalpable breast tumors: preliminary results,” Academic Radiology, vol. 15, no. 12, pp. 1526–1533, 2008. - PMC - PubMed
    1. Moinfar F, Man YG, Arnould L, Bratthauer GL, Ratschek M, and Tavassoli FA, “Concurrent and independent genetic alterations in the stromal and epithelial cells of mammary carcinoma: implications for tumorigenesis,” Cancer Research, vol. 60, no. 9, pp. 2562–2566, 2000. - PubMed
    1. Sarvazyan AP, “Elastic properties of soft tissue,” in Handbook of elastic properties of solid, liquid and gases - Vol III: Elastic properties of solids: Biological and organical materials, earth and marine sciences, Levy Bass, and Stern, Eds. New York: Academic, 2001, ch. 5.
    1. Krouskop TA, Wheeler TM, Kallel F, Garra BS, and Hall T, “Elastic moduli of breast and prostate tissues under compression,” Ultrasonic Imaging, vol. 20, no. 4, pp. 260–274, 1998. - PubMed
    1. Berg WA et al., “Shear-wave elastography improves the specificity of breast US: the BE1 multinational study of 939 masses,” Radiology, vol. 262, no. 2, pp. 435–449, 2012. - PubMed

Publication types