Spatiotemporal ontogeny of brain wiring - PubMed Skip to main page content
U.S. flag

An official website of the United States government

Dot gov

The .gov means it’s official.
Federal government websites often end in .gov or .mil. Before sharing sensitive information, make sure you’re on a federal government site.

Https

The site is secure.
The https:// ensures that you are connecting to the official website and that any information you provide is encrypted and transmitted securely.

Access keys NCBI Homepage MyNCBI Homepage Main Content Main Navigation
. 2019 Jun 12;5(6):eaav9694.
doi: 10.1126/sciadv.aav9694. eCollection 2019 Jun.

Spatiotemporal ontogeny of brain wiring

A Goulas  1 , R F Betzel  2   3   4 , C C Hilgetag  1   5
Affiliations

Spatiotemporal ontogeny of brain wiring

A Goulas et al. Sci Adv. .

Abstract

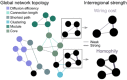
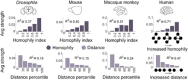
The wiring of vertebrate and invertebrate brains provides the anatomical skeleton for cognition and behavior. Connections among brain regions are characterized by heterogeneous strength that is parsimoniously described by the wiring cost and homophily principles. Moreover, brains exhibit a characteristic global network topology, including modules and hubs. However, the mechanisms resulting in the observed interregional wiring principles and network topology of brains are unknown. Here, with the aid of computational modeling, we demonstrate that a mechanism based on heterochronous and spatially ordered neurodevelopmental gradients, without the involvement of activity-dependent plasticity or axonal guidance cues, can reconstruct a large part of the wiring principles (on average, 83%) and global network topology (on average, 80%) of diverse adult brain connectomes, including fly and human connectomes. In sum, space and time are key components of a parsimonious, plausible neurodevelopmental mechanism of brain wiring with a potential universal scope, encompassing vertebrate and invertebrate brains.

PubMed Disclaimer

Figures

Fig. 1
Fig. 1. Brain connectomes are characterized by interregional connectivity strength heterogeneity and exhibit characteristic global network topology.
Heterogeneity of interregional connectivity strength is parsimoniously described by the wiring cost and connectional homophily principles (right). Schematic depiction illustrates characteristic global network topology features observed in diverse brain connectomes (left). For simplicity, directionality of connections is omitted.
Fig. 2
Fig. 2. Homophily, physical distance, and strength heterogeneity of empirical brain connectomes.
Increased homophily entails increased connectivity strength. Increased physical distance entails decreased connectivity strength. Depicted R2 values are derived from univariate regression. See the “Homophily and wiring cost principles” section for results and R2 values concerning multivariate regression. Note that the correlation, and thus the reported R2 values, between the strength of connections, physical distance, and homophily was computed on the nonbinned data and that the binning was performed for aiding visualization. Drosophila brain drawing from (6).
Fig. 3
Fig. 3. Developmental modeling approach.
(A) Neurodevelopmental gradients were simulated in a synthetic 2D brain. Each surface unit was characterized by a time window that indicates at a given time point the probability of a neuronal population migrating to each surface unit. Each time window is shaped by the distance of each surface unit from the root(s), that is, origin(s), of the neurodevelopmental gradients. For instance, the surface unit close to the neurodevelopmental origin (petrol green) is more probable to be populated earlier than the surface unit further from the neurodevelopmental origin (magenta). (B) Heterochronous and spatially ordered ontogeny of synthetic connectomes. (C) Heterochronous, spatially random, and (D) tautochronous ontogeny of synthetic connectomes. (E) Creation of the synthetic connectome. Note that time units depicted in (A) and (B) denote “developmental ticks” occurring in a time frame from the onset of the simulation of development and connectivity formation until the end of the simulation (for details, see the “Modeling neurodevelopmental gradients and connectivity formation” section).
Fig. 4
Fig. 4. Interregional wiring principles captured by the synthetic connectomes.
Summary of R2 values for the predictions of empirical connectivity from parameters calculated exclusively from the synthetic connectomes. Values for R2 are depicted for the (A) Drosophila, (B) mouse, (C) macaque monkey, and (D) human connectomes when considering the homophily or wiring cost principles separately or simultaneously. Note the better fit of the heterochronously generated connectomes when considering simultaneously the wiring cost and homophily principles. Note that the predictions from model parameters estimated exclusively from the synthetic connectomes result in R2 values very close to R2 values obtained when using exclusively the empirical data (gray bars), thus replicating a high percentage of the empirically observed relations between connectivity strength, homophily, and distance across vertebrate and invertebrate brains. Drosophila brain drawing from (6).
Fig. 5
Fig. 5. Network topology morphospace of synthetic connectomes and classification of empirical connectomes.
(A) Heterochronously and tautochronously generated connectomes are separated on the basis of their global network properties across the first component. Core size (max clique size) and characteristic path length (average shortest path length) are the major network metrics differentiating the groups. (B) Posterior probabilities indicate that the global network topology signature of all empirical connectomes is more reminiscent of connectomes with a heterochronous ontogeny. Drosophila brain drawing from (6).
Fig. 6
Fig. 6. Global network topology metrics for the synthetic and empirical connectomes.
The percentages below each bar denote the percentage of the values obtained for each network metric in relation to the empirical values. Thus, values over or below 100% denote overestimation or underestimation of the network metric, respectively. Drosophila brain drawing from (6).
See this image and copyright information in PMC

Similar articles

See all similar articles

Cited by

See all "Cited by" articles

References

    1. Geschwind N., Disconnexion syndromes in animals and man. I. Brain 88, 237–294 (1965). - PubMed
    1. Oh S. W., Harris J. A., Ng L., Winslow B., Cain N., Mihalas S., Wang Q., Lau C., Kuan L., Henry A. M., Mortrud M. T., Ouellette B., Nguyen T. N., Sorensen S. A., Slaughterbeck C. R., Wakeman W., Li Y., Feng D., Ho A., Nicholas E., Hirokawa K. E., Bohn P., Joines K. M., Peng H., Hawrylycz M. J., Phillips J. W., Hohmann J. G., Wohnoutka P., Gerfen C. R., Koch C., Bernard A., Dang C., Jones A. R., Zeng H., A mesoscale connectome of the mouse brain. Nature 508, 207–214 (2014). - PMC - PubMed
    1. Markov N. T., Ercsey-Ravasz M. M., Ribeiro Gomes A. R., Lamy C., Magrou L., Vezoli J., Misery P., Falchier A., Quilodran R., Gariel M. A., Sallet J., Gamanut R., Huissoud C., Clavagnier S., Giroud P., Sappey-Marinier D., Barone P., Dehay C., Toroczkai Z., Knoblauch K., van Essen D. C., Kennedy H., A weighted and directed interareal connectivity matrix for macaque cerebral cortex. Cereb. Cortex 24, 17–36 (2014). - PMC - PubMed
    1. Chiang A.-S., Lin C.-Y., Chuang C.-C., Chang H.-M., Hsieh C.-H., Yeh C.-W., Shih C.-T., Wu J.-J., Wang G.-T., Chen Y.-C., Wu C.-C., Chen G.-Y., Ching Y.-T., Lee P.-C., Lin C.-Y., Lin H.-H., Wu C.-C., Hsu H.-W., Huang Y.-A., Chen J.-Y., Chiang H.-J., Lu C.-F., Ni R.-F., Yeh C.-Y., Hwang J.-K., Three-dimensional reconstruction of brain-wide wiring networks in Drosophila at single-cell resolution. Curr. Biol. 21, 1–11 (2011). - PubMed
    1. Rubinov M., Ypma R. J. F., Watson C., Bullmore E. T., Wiring cost and topological participation of the mouse brain connectome. Proc. Natl. Acad. Sci. U.S.A. 112, 10032–10037 (2015). - PMC - PubMed

Publication types

LinkOut - more resources