Deep temporal models and active inference
- PMID: 28416414
- PMCID: PMC5461873
- DOI: 10.1016/j.neubiorev.2017.04.009
Deep temporal models and active inference
Erratum in
-
Deep temporal models and active inference.Neurosci Biobehav Rev. 2018 Jul;90:486-501. doi: 10.1016/j.neubiorev.2018.04.004. Epub 2018 May 8. Neurosci Biobehav Rev. 2018. PMID: 29747865 Free PMC article.
Abstract
How do we navigate a deeply structured world? Why are you reading this sentence first - and did you actually look at the fifth word? This review offers some answers by appealing to active inference based on deep temporal models. It builds on previous formulations of active inference to simulate behavioural and electrophysiological responses under hierarchical generative models of state transitions. Inverting these models corresponds to sequential inference, such that the state at any hierarchical level entails a sequence of transitions in the level below. The deep temporal aspect of these models means that evidence is accumulated over nested time scales, enabling inferences about narratives (i.e., temporal scenes). We illustrate this behaviour with Bayesian belief updating - and neuronal process theories - to simulate the epistemic foraging seen in reading. These simulations reproduce perisaccadic delay period activity and local field potentials seen empirically. Finally, we exploit the deep structure of these models to simulate responses to local (e.g., font type) and global (e.g., semantic) violations; reproducing mismatch negativity and P300 responses respectively.
Keywords: Active inference; Bayesian; Free energy; Hierarchical; MMN; P300; Reading; Violation.
Copyright © 2017 The Authors. Published by Elsevier Ltd.. All rights reserved.
Figures
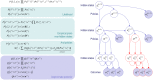
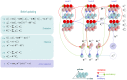
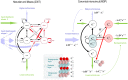
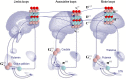

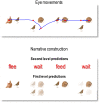

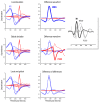
Similar articles
-
Generative models, linguistic communication and active inference.Neurosci Biobehav Rev. 2020 Nov;118:42-64. doi: 10.1016/j.neubiorev.2020.07.005. Epub 2020 Jul 17. Neurosci Biobehav Rev. 2020. PMID: 32687883 Free PMC article. Review.
-
Deep temporal models and active inference.Neurosci Biobehav Rev. 2018 Jul;90:486-501. doi: 10.1016/j.neubiorev.2018.04.004. Epub 2018 May 8. Neurosci Biobehav Rev. 2018. PMID: 29747865 Free PMC article.
-
Human visual exploration reduces uncertainty about the sensed world.PLoS One. 2018 Jan 5;13(1):e0190429. doi: 10.1371/journal.pone.0190429. eCollection 2018. PLoS One. 2018. PMID: 29304087 Free PMC article.
-
Deep Active Inference and Scene Construction.Front Artif Intell. 2020 Oct 28;3:509354. doi: 10.3389/frai.2020.509354. eCollection 2020. Front Artif Intell. 2020. PMID: 33733195 Free PMC article.
-
Biased belief in the Bayesian brain: A deeper look at the evidence.Conscious Cogn. 2019 Feb;68:107-114. doi: 10.1016/j.concog.2019.01.006. Epub 2019 Jan 19. Conscious Cogn. 2019. PMID: 30665186 Review.
Cited by
-
Generative models, linguistic communication and active inference.Neurosci Biobehav Rev. 2020 Nov;118:42-64. doi: 10.1016/j.neubiorev.2020.07.005. Epub 2020 Jul 17. Neurosci Biobehav Rev. 2020. PMID: 32687883 Free PMC article. Review.
-
Commentary: A Compositional Neural Architecture for Language.Front Psychol. 2020 Sep 2;11:2101. doi: 10.3389/fpsyg.2020.02101. eCollection 2020. Front Psychol. 2020. PMID: 32982860 Free PMC article. No abstract available.
-
Incremental Learning of Goal-Directed Actions in a Dynamic Environment by a Robot Using Active Inference.Entropy (Basel). 2023 Oct 31;25(11):1506. doi: 10.3390/e25111506. Entropy (Basel). 2023. PMID: 37998198 Free PMC article.
-
Presence, flow, and narrative absorption: an interdisciplinary theoretical exploration with a new spatiotemporal integrated model based on predictive processing.Open Res Eur. 2021 Jul 23;1:28. doi: 10.12688/openreseurope.13193.2. eCollection 2021. Open Res Eur. 2021. PMID: 37645177 Free PMC article.
-
Am I Self-Conscious? (Or Does Self-Organization Entail Self-Consciousness?).Front Psychol. 2018 Apr 24;9:579. doi: 10.3389/fpsyg.2018.00579. eCollection 2018. Front Psychol. 2018. PMID: 29740369 Free PMC article.
References
-
- Arikuni T., Kubota K. The organization of prefrontocaudate projections and their laminar origin in the macaque monkey: a retrograde study using HRP-gel. J. Comp. Neurol. 1986;244:492–510. - PubMed
-
- Barlow H. Possible principles underlying the transformations of sensory messages. In: Rosenblith W., editor. Sensory Communication. MIT Press; Cambridge, MA: 1961. pp. 217–234.
-
- Barlow H.B. Inductive inference, coding, perception, and language. Perception. 1974;3:123–134. - PubMed
-
- Beal M.J. University College London; 2003. Variational Algorithms for Approximate Bayesian Inference. PhD Thesis.
Publication types
MeSH terms
Grants and funding
LinkOut - more resources
Full Text Sources
Other Literature Sources
Miscellaneous

