Abstract
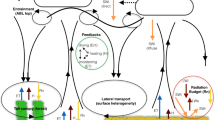
The relationship between potential evaporation and arealevaporation is assessed using a closed-box model of the convectiveboundary layer (CBL). Potential evaporation is defined as theevaporation that would occur from a hypothetical saturated surface,with radiative properties similar to those of the whole area, and smallenough that the excess moisture flux does not modify thecharacteristics of the CBL. It is shown that the equilibrium rate ofpotential evaporation is given by Ep0=αE0,where E0 is the equilibrium evaporation (radiative termof the Penman formula), and α is a coefficient similar to thePriestley-Taylor coefficient. Its expression is \(\alpha = 1 + \left[ {1/(\varepsilon + 1)} \right]\left( {\left\langle {r_s } \right\rangle /r_a } \right)\), where \(\left\langle {r_s } \right\rangle \) is the areal surface resistance, ra is the localaerodynamic resistance, and ε is the dimensionless slope of thesaturation specific humidity at the temperature of the air. Itscalculated value is around 1 for any saturated surface surrounded bywater, about 1.3 for saturated grass surrounded by well-watered grassand can be greater than 3 over saturated forest surrounded by forest.The formulation obtained provides a theoretical basis to the overallmean value of 1.26, empirically found by Priestley and Taylor for thecoefficient α. Examining, at the light of this formulation, thecomplementary relationship between potential and actual evaporation(as proposed by Bouchet and Morton), it appears that the sum ofthese two magnitudes is not a constant at equilibrium, but depends onthe value of the areal surface resistance.
Similar content being viewed by others
References
Bouchet, R. J.: 1963, 'Evapotranspiration Réelle et Potentielle. Signification Climatique’, in: Proc. Berkeley, Calif. Symp. IAHS, Publ. 62, 134–142.
Brutsaert, W.: 1982, Evaporation into the atmosphere. D. Reidel Pub. Co., Dordrecht, 299 pp.
Brutsaert, W. and Stricker, H.: 1979, ‘An Advection-Aridity Approach to Estimate Actual Regional Evapotranspiration’, Water Resour.Res. 15, 443–450.
Culf, A. D.: 1994, ‘Equilibrium Evaporation Beneath a Growing Convective Boundary Layer’, Boundary Layer Meteorol. 71, 37–49.
Davies, J. A. and Allen, C. D.: 1973, ‘Equilibrium, Potential and Actual Evaporation from Cropped Surfaces in Southern Ontario’, J. Appl. Meteorol. 12, 649–657.
J. Climate and Appl. Meteorol. 22, 572–578.
De Bruin, H. A. R.: 1989, ‘Physical Aspects of the Planetary Boundary Layer with Special Reference to Regional Evapotranspiration’, in: Estimation of Areal Evapotranspiration, IAHS, Publ. 177.
De Bruin, H. A. R. and Keijman, J. Q.: 1979, ‘The Priestley-Taylor Evaporation Model Applied to Large, Shallow Lake in the Netherlands’, J. Appl. Meteorol. 18, 898–903.
Fortin, J. P. and Seguin, B.: 1975, 'Estimation de l'ETR régionale à Partir de l'ETP Locale: Utilisation de la Relation de Bouchet à Différentes Échelles de Temps’, Ann. Agron. 26, 537–554.
Garratt, J. R.: 1994, The Atmospheric Boundary Layer. Cambridge University Press, 316 pp.
Granger, R. J.: 1989, ‘An Examination of the Concept of Potential Evaporation’, J. Hydrol. 111, 9–19.
Granger, R. J.: 1989, ‘A Complementary Relationship Approach for Evaporation from Non-saturated Surfaces’, J. Hydrol. 111, 31–38.
Jacobs, C. M. J. and de Bruin, H. A. R.: 1992, ‘The Sensitivity of Regional Transpiration to Land-Surface Characteristics: Significance of Feedback’, J. Climate 5, 683–698.
LeDrew, E. F.: 1979, ‘A Diagnostic Examination of a Complementary Relationship Between Actual and Potential Evapotranspiration’, J. Appl. Meteorol. 18, 495–501.
McIlroy, I. C. and Angus, D. E.: 1964, ‘Grass, Water and Soil Evaporation at Aspendale’, Agric. Meteorol. 1, 201–224.
McMillan, W. D. and Burgy, R. H.: 1960, ‘Interception Loss from Grass’, J. Geophys. Res. 65, 2389–2394.
McNaughton, K. G.: 1976, ‘Evaporation and Advection I: Evaporation from Extensive Homogeneous Surfaces’, Quarti J. Roy. Meteorol. Soc. 102, 181–191.
McNaughton, K. G.: 1989, 'Regional Interactions Between Canopies and the Atmosphere', in G. Russel et al. (ed.), Plant Canopies: Their Growth, Form and Function, SEB seminar series, 31. Cambridge University Press, pp. 63–82.
McNaughton, K. G. and Jarvis, P. G.: 1983, ‘Predicting the Effects of Vegetation Changes on Transpiration and Evaporation', in: T. T. Kozlowski (ed.), Water Deficits and Plant Growth, Vol. VII. Academic Press, London, pp. 1–47.
McNaughton, K. G. and Spriggs, T. W.: 1986, ‘A Mixed-Layer Model for Regional Evaporation’, Boundary Layer Meteorol. 34, 243–262.
McNaughton, K. G. and Spriggs, T. W.: 1989, ‘An Evaluation of the Priestley-Taylor Equation and the Complementary Relationship Using Results from a Mixed-Layer Model of the Convective Boundary Layer’, in Estimation of Areal Evapotranspiration, IAHS, Publ. 177.
Monteith, J. L.: 1981, 'Evaporation and Surface Temperature', Quart. J. Roy. Meteorol. Soc. 107, 1–27.
Monteith, J. L.: 1985, ‘Evaporation from Land Surfaces: Progress in Analysis and Prediction since 1948’, in Advances in Evapotranspiration, ASAE, pp. 4–12.
Moore, C. J.: 1976, ‘A Comparative Study of Radiation Balance Above Forest and Grassland’,Quart. J. Roy. Meteorol. Soc. 102, 889–899.
Morton, F. I.: 1969, ‘Potential Evaporation as a Manifestation ofRegional Evaporation’,Water Resour. Res. 5, 1244–1255.
Morton, F. I.: 1975, ‘Estimating Evaporation and Transpiration from Climatological Observations’, J. Appl. Meteorol. 14, 488–497.
Morton, F. I.: 1983, ‘Operational Estimates of Areal Evapotranspiration and their Significance to the Science and Practice of Hydrology’, J. Hydrol. 66, 1–76.
Nash, J. E.: 1989, ‘Potential Evaporation and "The Complementary Relationship"’, J. Hydrol. 111, 1–7.
Penman, H. L.: 1956, ‘Evaporation: An Introductory Survey’, Netherlands J. Agric. Sci. 1, 9–29.
Perrier, A.: 1980, 'Etude Microclimatique des Relations Entre les Propriétés de Surface et les Caract éristiques de l'Air’, in: Météorologie et Environnement, Evry, France.
Perrier, A.: 1982, ‘Land Surface Processes: Vegetation’, in: P. S. Eagleson (ed.), Land Surface Processes in Atmospheric General Circulation Models, Cambridge University Press.
Priestley, C. H. B. and Taylor, R. J.: 1972, ‘On the Assessment of Surface Heat Flux and Evaporation Using Large-Scale Parameters’, Mon. Wea. Rev. 100, 81–92.
Raupach, M. R.: 1991, ‘Vegetation-Atmosphere Interaction in Homogeneous and Heterogeneous Terrain: Some Implications of Mixed-Layer Dynamics’, Vegetatio 91, 105–120.
Seguin, B.: 1975, 'Influence de l' évapotranspiration Régionale sur la Mesure Locale d' évapotranspiration Potentielle’, Agric. Meteorol. 15, 355–370.
Slatyer, R. O. and McIlroy, I. C.: 1961, Practical Micrometeorology, CSIRO, Melbourne, Australia. 310 pp.
Stewart, J. B.: 1977, ‘Evaporation from the Wet Canopy of a Pine Forest’, Water Resour. Res. 13, 915–921.
Thornthwaite, C. W.: 1948, ‘An Approach Toward a Rational Classification of Climate’, Geogr. Rev. 38, 55–94.
Author information
Authors and Affiliations
Rights and permissions
About this article
Cite this article
LHOMME, JP. A THEORETICAL BASIS FOR THE PRIESTLEY-TAYLOR COEFFICIENT. Boundary-Layer Meteorology 82, 179–191 (1997). https://doi.org/10.1023/A:1000281114105
Issue Date:
DOI: https://doi.org/10.1023/A:1000281114105