Abstract
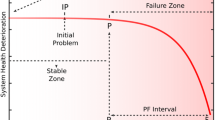
In the paper presented a novel technique of intelligent constructing exact statistical prediction and tolerance limits on future random quantities for prognostics and health management of complex systems under parametric uncertainty is proposed. The aim of this technique is to develop and publish original scientific contributions and industrial applications dealing with the topics covered by Prognostics and Health Management (PHM) of complex systems. PHM is a set of means, approaches, methods and tools that allows monitoring and tracking the health state of a system in order to detect, diagnose and predict its failures. This information is then exploited to take appropriate decisions to increase the system’s availability, reliability and security while reducing its maintenance costs. The proposed technique allows one to construct developments and results in the areas of condition monitoring, fault detection, fault diagnostics, fault prognostics and decision support.
Similar content being viewed by others
REFERENCES
Patel, J.K., Tolerance limits: A review, Commun. Stat.: Theory Methodol., 1986, vol. 15, pp. 2719–2762.
Dunsmore, J.R., Some approximations for tolerance factors for the two parameter exponential distribution, Technometrics, 1978, vol. 20, pp. 317–318.
Guenther, W.C., Patil, S.A., and Uppuluri, V.R.R., One-sided β-content tolerance factors for the two parameter exponential distribution, Technometrics, 1976, vol. 18, pp. 333–340.
Engelhardt, M. and Bain, L.J., Tolerance limits and confidence limits on reliability for the two-parameter exponential distribution, Technometrics, 1978, vol. 20, pp. 37–39.
Guenther, W.C., Tolerance intervals for univariate distributions, Naval Res. Logist. Q., 1972, vol. 19, pp. 309–333.
Hahn, G.J. and Meeker, W.Q., Statistical Intervals: A Guide for Practitioners, New York: John Wiley and Sons, 1991.
Nechval, K.N. and Nechval, N.A., Constructing lower simultaneous prediction limits on observations in future samples from the past data, Comput. Ind. Eng., 1999, vol. 137, pp. 133–136.
Nechval, N.A. and Vasermanis, E.K., Improved Decisions in Statistics, Riga: Izglitibas soli, 2004.
Nechval, K.N. and Nechval, N.A., Technique of constructing predictive inferences for future outcomes with applications to inventory management problems, Adv. Syst. Sci. Appl., 2013, vol. 13, pp. 355–368.
Nechval, N.A. and Nechval, K.N., A new approach to constructing simultaneous prediction limits on future outcomes under parametric uncertainty of underlying models, in IAENG Transactions on Engineering Sciences, Ao Sio-long, Chan Alan Hoi-Shou, Katagiri Hideki, and Xu Li, Eds., London: Taylor and Francis Group, 2014, pp. 1–13.
Nechval, N.A. and Nechval, K.N., Tolerance limits on order statistics in future samples coming from the two-parameter exponential distribution, Am. J. Theor. Appl. Stat., 2016, vol. 5, pp. 1–6.
Nechval, N.A., Nechval, K.N., Prisyazhnyuk, S.P., and Strelchonok, V.F., Tolerance limits on order statistics in future samples coming from the Pareto distribution, Autom. Control Comput. Sci., 2016, vol. 50, no. 6, pp. 423–431.
Nechval, N.A., Nechval, K.N., and Strelchonok, V.F., A new approach to constructing tolerance limits on order statistics in future samples coming from a normal distribution, Adv. Image Video Process., 2016, vol. 4, pp. 47–61.
Nechval, N.A., Berzins, G., Balina, S., Steinbuka, I., and Nechval, K.N., Constructing unbiased prediction limits on future outcomes under parametric uncertainty of underlying models via pivotal quantity averaging approach, Autom. Control Comput. Sci., 2017, vol. 51, no. 5, pp. 331–346.
Nechval, N.A., Nechval, K.N., and Berzins, G., A new technique for constructing exact tolerance limits on future outcomes under parametric uncertainty, in Advanced Mathematical Techniques in Engineering Sciences, Ram, M. and Davim, J.P., Eds., London: Taylor and Francis Group, 2018, pp. 203–226.
Nechval, N.A., Berzins, G., Nechval, K.N., and Krasts, J., A new technique of intelligent constructing unbiased prediction limits on future order statistics coming from an inverse Gaussian distribution under parametric uncertainty, Autom. Control Comput. Sci., 2019, vol. 53, no. 3, pp. 223–235.
Mann, N.R. and Saunders, S.C., On evaluation of warranty assurance when life has a Weibull distribution, Biometrika, 1969, vol. 56, pp. 615–625.
Lawless, J.F., On the estimation of safe life when the underlying life distribution is Weibull, Technometrics, 1973, vol. 15, pp. 857–865.
Mee, R.W. and Kushary, D., Prediction limits for the Weibull distribution utilizing simulation, Comput. Stat. Data Anal., 1994, vol. 17, pp. 327–336.
Nechval, N.A., Nechval, K.N., Danovich, V., and Berzins, G., Predictive inferences for future order statistics coming from an inverse Gaussian distribution, Lecture Notes in Engineering and Computer Science: Proceedings of The World Congress on Engineering, London, 2014, pp. 888–893.
Nechval, N.A., Nechval, K.N., and Berzins, G., A new technique for intelligent constructing exact γ-content tolerance limits with expected (1 – α)-confidence on future outcomes in the Weibull case using complete or Type II censored data, Autom. Control Comput. Sci., 2014, vol. 52, pp. 476–488.
Nechval, N.A. and Nechval, K.N., Improved planning in-service inspections of fatigued aircraft structures under parametric uncertainty of underlying lifetime models, in Numerical Methods for Reliability and Safety Assessment: Multiscale and Multiphysics Systems, Kadry, S. and Hami, A.El., Eds., Springer International Publishing, 2015, pp. 647–674.
Nechval, N.A., Berzins, G., and Nechval, K.N., Intelligent planning reliability-based inspections of fatigued structures for the crack initiation period in the Weibull case under parametric uncertainty, Autom. Control Comput. Sci., 2018, vol. 52, no. 3, pp. 184–197.
Nechval, K.N., Nechval, N.A., Berzins, G., and Purgailis, M., Optimal statistical decisions in a new product lifetime testing, Proceedings of the Fourth International Conference on Maintenance and Facility Management, Rome, 2009, pp. 135–140.
McGill, J.I. and Van Ryzin, G.J., Revenue management: Research overview and prospects, Transp. Sci., 1999, vol. 33, pp. 233–256.
Littlewood, K., Forecasting and control of passenger bookings, Proceedings of the 12th AGIFORS Symposium, New York, 1972, pp. 95–117.
Richter, H., The differential revenue method to determine optimal seat allotments by fare type, Proceedings of the XXII AGIFORS Symposium, New York, 1982, pp. 339–362.
Belobaba, P.P., Airline yield management: An overview of seat inventory control, Transp. Sci., 1987, vol. 21, pp. 66–73.
Curry, R.E., Optimal airline seat allocation with fare classes nested by origins and destinations, Transp. Sci., 1990, vol. 24, pp. 193–203.
Wollmer, R.D., An airline seat management model for a single leg route when lower fare classes book first, Oper. Res., 1992, vol. 40, pp. 26–37.
Brumelle, S.L. and McGill, J.I., Airline seat allocation with multiple nested fare classes, Oper. Res., 1993, vol. 41, pp. 127–137.
Nechval, N.A, Nechval, K.N., and Vasermanis, E.K., Optimal control of airline booking process, Proceedings of the 15th IFAC World Congress, Barcelona, Spain, 21–26 July 2002, vol. D: Optimal Control, 2003, pp. 1–6.
Nechval, K.N., Nechval, N.A., Rozite, K., and Vasermanis, E.K., Optimal airline seat inventory control for multi-leg flights, Proceedings of the 16th IFAC World Congress, 2005, vol. 38, no. 1, pp. 31–36.
Nechval, N.A., Rozite, K., and Strelchonok, V.F., Optimal airline multi-leg flight seat inventory control, in Computing Anticipatory Systems, Dubois, D.M., Ed.; AIP (American Institute of Physics) Proceedings, Melville, New York, 2006, vol. 839, pp. 591–600.
Nechval, N.A., Berzins, G., and Nechval, K.N., Improved airline seat inventory control under incomplete information, in IAENG Transactions on Engineering Sciences, Sio-long Ao, Alan Hoi-Shou Chan, Hideki Katagiri, and Li Xu, Eds., London: Taylor and Francis Group, 2014, pp. 269–277.
Nechval, N.A., Berzins, G., and Danovics, V., A new dynamic model for anticipatory adaptive control of airline seat reservation via order statistics of cumulative customer demand, in Advances in Artificial Intelligences, Salem Benferhat, Karim Tabia, and Moonis Ali, Eds., Springer International Publishing AG, 2017, pp. 359–370.
Nechval, N.A., Berzins, G., and Nechval, K.N., A new intelligent technique of constructing optimal airline seat protection levels for multiple nested fare classes of single-leg flights, Proceedings of the IEEE—6th 2019 International Conference on Control, Decision and Information Technologies (CoDIT'19), Paris, 2019.
Nechval, N.A., Berzins, G., and Nechval, K.N., A novel intelligent technique for product acceptance process optimization on the basis of misclassification probability in the case of log-location-scale distributions, in Advances and Trends in Artificial Intelligence. From Theory to Practice, Wotawa, F., et al., Eds., Springer Nature Switzerland AG; Lect. Notes Comput. Sci., 2019, vol. 11606, pp. 801–818.
ACKNOWLEDGMENTS
The authors would like to thank the anonymous reviewers for their valuable comments that helped to improve the presentation of this paper.
Author information
Authors and Affiliations
Corresponding author
Ethics declarations
The authors declare that they have no conflicts of interest regarding the publication of this paper.
About this article
Cite this article
Nechval, N.A., Berzins, G. & Nechval, K.N. Intelligent Constructing Exact Statistical Prediction and Tolerance Limits on Future Random Quantities for Prognostics and Health Management of Complex Systems. Aut. Control Comp. Sci. 53, 532–549 (2019). https://doi.org/10.3103/S0146411619060087
Received:
Revised:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.3103/S0146411619060087