Asynchronous Finite-Time Exponential Dissipative Filtering for Stochastic Fuzzy Markov Jump Systems Against Deception Attacks Based on Event-Triggered Scheme | International Journal of Fuzzy Systems Skip to main content
Springer Nature Link
Log in

Asynchronous Finite-Time Exponential Dissipative Filtering for Stochastic Fuzzy Markov Jump Systems Against Deception Attacks Based on Event-Triggered Scheme

  • Published:
International Journal of Fuzzy Systems Aims and scope Submit manuscript

Abstract

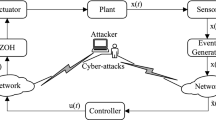
This note aims to investigate finite-time exponential dissipative (FTED) filtering for stochastic fuzzy Markov jump systems (MJSs) subject to multiplicative noise and deception attacks. A mode-dependent event-triggered mechanism (ETM) is adopted to mitigate the pressure of network transmission, and cyber attacks are characterized in the communication channel. Besides, a hidden Markov model(HMM) is employed to describe that the system modes and filter modes are not synchronized. According to ETM and HMM, a hidden networked filtering error system model with network-induced delay and stochastic deception attacks is established. Sufficient conditions of finite-time stochastic boundedness (FTSB) are then provided for the resulting filtering error system. Furthermore, under randomly occurring deception attacks, mode-dependent event-triggered matrices and asynchronous fuzzy filter gains are co-designed, which guarantee that the filtering error system is FTSB as well as FTED. Finally, an example is presented to verify the effectiveness of obtained results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11
Fig. 12
Fig. 13
Fig. 14
Fig. 15
Fig. 16

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Narendra, K.S., Tripathi, S.S.: Identification and optimization of aircraft dynamics. J. Aircr. 10(4), 193–199 (1973)

    Google Scholar 

  2. Xue, M., Yan, H.C., Wang, M., Shen, H., Shi, K.B.: LSTM-based intelligent fault detection for fuzzy Markov jump systems and its application to tunnel diode circuits. IEEE Trans. Circ. Syst. II 69(3), 1099–1103 (2022)

    Google Scholar 

  3. Kuppusamy, S., Joo, Y.H., Kim, H.S.: Asynchronous control for discrete-time hidden Markov jump power systems. IEEE Trans. Cybern. 52(9), 9943–9948 (2022)

    Google Scholar 

  4. Shi, P., Li, F.B.: A survey on Markovian jump systems: modeling and design. Int. J. Control 13(1), 1–16 (2015)

    Google Scholar 

  5. Shen, Y., Wu, Z.G., Shi, P., Shu, Z., Karimi, H.R.: \(H_{\infty }\) control of Markov jump time-delay systems under asynchronous controller and quantizer. Automatica 99, 352–360 (2019)

    MathSciNet  Google Scholar 

  6. Pan, Y.N., Yang, G.H.: Event-triggered fault detection filter design for nonlinear networked systems. IEEE Trans. Syst. Man Cybern. Syst. 48(11), 1851–1862 (2017)

    Google Scholar 

  7. Takagi, T., Sugeno, M.: Fuzzy identification of systems and its applications to modeling and control. IEEE Trans. Syst. Man Cybern. Syst. 15(1), 116–132 (1985)

    Google Scholar 

  8. Xue, M., Yan, H.C., Zhang, H., Li, Z.C., Chen, S.M., Chen, C.Y.: Event-triggered guaranteed cost controller design for T-S fuzzy Markovian jump systems with partly unknown transition probabilities. IEEE Trans. Fuzzy Syst. 29(5), 1052–1064 (2020)

    Google Scholar 

  9. Wu, Z.G., Dong, S.L., Shi, P., Su, H.Y., Huang, T.W., Lu, R.Q.: Fuzzy-model-based nonfragile guaranteed cost control of nonlinear Markov jump systems. IEEE Trans. Syst. Man Cybern. Syst. 47(8), 2388–2397 (2017)

    Google Scholar 

  10. Zhang, M., Shi, P., Ma, L.H., Cai, J.P., Su, H.Y.: Quantized feedback control of fuzzy Markov jump systems. IEEE Trans. Cybern. 49(9), 3375–3384 (2019)

    Google Scholar 

  11. Tian, Y.F., Wang, Z.S.: A switched fuzzy filter approach to \(H_{\infty }\) filtering for Takagi-Sugeno fuzzy Markov jump systems with time delay: The continuous-time case. Inf. Sci. 557, 236–249 (2021)

    MathSciNet  Google Scholar 

  12. Hua, M.G., Qian, Y.Y., Deng, F.Q., Fei, J.T., Cheng, P., Chen, H.: Filtering for discrete-time Takagi-Sugeno fuzzy nonhomogeneous Markov jump systems with quantization effects. IEEE Trans. Cybern. 52(2), 982–995 (2022)

    Google Scholar 

  13. Zhang, M., Shi, P., Liu, Z.T., Cai, J.P., Su, H.Y.: Dissipativity-based asynchronous control of discrete-time Markov jump systems with mixed time delays. Int. J. Robust Nonlinear Control 28(6), 2161–2171 (2018)

    MathSciNet  Google Scholar 

  14. Shen, Y., Wu, Z.G., Shi, P., Shu, Z., Karimi, H.R.: \(H_{\infty }\) control of Markov jump time-delay systems under asynchronous controller and quantizer. Automatica 99, 352–360 (2019)

    MathSciNet  Google Scholar 

  15. Stadtmann, F., Costa, O.L.: \(H_2\)-control of continuous-time hidden Markov jump linear systems. IEEE Trans. Autom. Control 62(8), 4031–4037 (2016)

    Google Scholar 

  16. Wu, Z.G., Shi, P., Shu, Z., Su, H.Y., Lu, R.Q.: Passivity-based asynchronous control for Markov jump systems. IEEE Trans. Autom. Control 62(4), 2020–2025 (2016)

    MathSciNet  Google Scholar 

  17. Wu, Z.G., Dong, S.L., Su, H.Y., Li, C.D.: Asynchronous dissipative control for fuzzy Markov jump systems. IEEE Trans. Cybern. 48(8), 2426–2436 (2017)

    Google Scholar 

  18. Dong, S.L., Wu, Z.G., Pan, Y.J., Su, H.Y., Liu, Y.: Hidden Markov model-based asynchronous filter design of nonlinear Markov jump systems in continuous-time domain. IEEE Trans. Cybern. 49(6), 2294–2304 (2019)

    Google Scholar 

  19. Xue, M., Yan, H.C., Zhang, H., Sun, J., Lam, H.K.: Hidden Markov model-based asynchronous \(H_ {\infty }\) tracking control of fuzzy Markov jump systems. IEEE Trans. Fuzzy Syst. 29(5), 1081–1092 (2020)

    Google Scholar 

  20. Yue, D., Tian, E.G., Han, Q.L.: A delay system method for designing event-triggered controllers of networked control systems. IEEE Trans. Autom. Control 58(2), 475–481 (2012)

    MathSciNet  Google Scholar 

  21. Zhang, X.M., Han, Q.L., Zhang, B.L.: An overview and deep investigation on sampled-data-based event-triggered control and filtering for networked systems. IEEE Trans. Ind. Inform. 13(1), 4–16 (2016)

    Google Scholar 

  22. Tao, J., Xiao, Z., Chen, J., Lin, M., Lu, R., Shi, P., Wang, X.: Event-triggered control for Markov jump systems subject to mismatched modes and strict dissipativity. IEEE Trans. Cybern. (2023). https://doi.org/10.1109/TCYB.2021.3105179

    Article  Google Scholar 

  23. Wang, Y., Yan, H.C., Zhang, H., Shen, H., Lam, H.K.: Interval type-2 fuzzy control for HMM-based multiagent systems via dynamic event-triggered scheme. IEEE Trans. Fuzzy Syst. 30(8), 3063–3073 (2022)

    Google Scholar 

  24. Liu, J., Ran, G.T., Huang, Y.Q., Han, C.S., Yu, Y., Sun, C.Y.: Adaptive event-triggered finite-time dissipative filtering for interval type-2 fuzzy Markov jump systems with asynchronous modes. IEEE Trans. Cybern. 52(9), 9709–9721 (2021)

    Google Scholar 

  25. Zhang, Y.Q., Shi, P., Basin, M.V.: Event-based finite-time \(H_\infty\) filtering of discrete-time singular jump network systems. Int. J. Robust Nonlinear Control 32(6), 4038–4054 (2022)

    MathSciNet  Google Scholar 

  26. Su, L., Ye, D.: Static output feedback control for discrete-time hidden Markov jump systems against deception attacks. Int. J. Robust Nonlinear Control 29(18), 6616–6637 (2019)

    MathSciNet  Google Scholar 

  27. Liu, J.L., Zhang, N., Li, Y., Xie, X.P.: \(H_\infty\) filter design for discrete-time networked systems with adaptive event-triggered mechanism and hybrid cyber attacks. J. Franklin Inst. 358, 9325–9345 (2021)

    MathSciNet  Google Scholar 

  28. Ran, G.T., Li, C.J., Sakthivel, R., Han, C.S., Wang, B.H., Liu, J.: Adaptive event-triggered asynchronous control for interval type-2 fuzzy Markov jump systems with cyber-attacks. IEEE Trans. Control. Netw. Syst. 9(1), 88–99 (2022)

    MathSciNet  Google Scholar 

  29. Wu, J.C., Peng, C., Zhang, J., Zhang, B.L.: Event-triggered finite-time \(H_{\infty }\) filtering for networked systems under deception attacks. J. Franklin Inst. 357(6), 3792–3808 (2020)

    MathSciNet  Google Scholar 

  30. Sakthivel, R., Kwon, O.M., Park, M.J., Choi, S.G., Sakthivel, R.: Robust asynchronous filtering for discrete-time T-S fuzzy complex dynamical networks against deception attacks. IEEE Trans. Fuzzy Syst. 30(8), 3257–3269 (2021)

    Google Scholar 

  31. Xu, Y., Wu, Z.G., Sun, J.: Security-based passivity analysis of Markov jump systems via asynchronous triggering control. IEEE Trans. Cybern. 53(1), 151–160 (2023)

    Google Scholar 

  32. Zhang, R.M., Wang, H.X., Park, J.H., He, P.S., et al.: Fuzzy secure control for nonlinear \(N\)-D parabolic PDE-ODE coupled systems under stochastic deception attacks. IIEEE Trans. Fuzzy Syst. 30(8), 3347–3359 (2021)

    Google Scholar 

  33. Yang, N., Zhang, S., Su, H.: Event-triggered impulsive control for stability of stochastic delayed complex networks under deception attacks. Eng. Appl. Artif. Intell. 121, 105953 (2023)

    Google Scholar 

  34. Ma, Y.M., Sun, S.L.: Distributed optimal and self-tuning filters based on compressed data for networked stochastic uncertain systems with deception attacks. Sensors 23(1), 1–19 (2023)

    Google Scholar 

  35. Wu, J., He, F.R., He, X.G., et al.: Dynamic event-triggered fuzzy adaptive control for non-strict-feedback stochastic nonlinear systems with injection and deception attacks. Int. J. Fuzzy Syst. 25(3), 1144–1155 (2023)

    Google Scholar 

  36. Zhang, H.G., Guo, X.Y., Sun, J.Y., Zhou, Y.: Event-triggered cooperative adaptive fuzzy control for stochastic nonlinear systems with measurement sensitivity and deception attacks. IEEE Trans. Fuzzy Syst. 31(3), 774–785 (2023)

    Google Scholar 

  37. Willems, J.: Dissipative dynamical systems. Part I: general theory. Arch. Ration. Mech. Anal. 45(5), 321–351 (1972)

    Google Scholar 

  38. Liu, Y.J., Guo, B.Z., Park, J.H., Lee, S.: Event-based reliable dissipative filtering for T-S fuzzy systems with asynchronous constraints. IEEE Trans. Fuzzy Syst. 26(4), 2089–2098 (2017)

    Google Scholar 

  39. Zhang, Y.Q., Shi, P., Agarwal, R.K., Shi, Y.: Event-based dissipative analysis for discrete time-delay singular jump neural networks. IEEE Trans. Neural Netw. Learn. Syst. 31(4), 1232–1241 (2019)

    MathSciNet  Google Scholar 

  40. Li, L.L., Yang, R.N., Feng, Z.G., Wu, L.G.: Event-triggered dissipative control for 2-D switched systems. Inf. Sci. 589, 802–812 (2022)

    Google Scholar 

  41. Xue, H.Y., Popov, M.: Analysis of switching transient overvoltages in the power system of floating production storage and offloading vessel. Electr. Power Syst. Res. 115, 3–10 (2014)

    Google Scholar 

  42. Amato, F., Ariola, M., Dorato, P.: Finite-time control of linear systems subject to parametric uncertainties and disturbances. Automatica 37(9), 1459–1463 (2001)

    Google Scholar 

  43. Amato, F., Ariola, M., Cosentino, C.: Finite-time stabilization via dynamic output feedback. Automatica 42(2), 337–342 (2006)

    MathSciNet  Google Scholar 

  44. Yan, Z.G., Zhang, W.H., Zhang, G.S.: Finite-time stability and stabilization of Itô stochastic systems with Markovian switching: mode-dependent parameter approach. IEEE Trans. Autom. Control 60(9), 2428–2433 (2014)

    Google Scholar 

  45. Song, J., Niu, Y.G., Wang, S.B.: Robust finite-time dissipative control subject to randomly occurring uncertainties and stochastic fading measurements. J. Franklin Inst. 354(9), 3706–3723 (2017)

    MathSciNet  Google Scholar 

  46. Han, H.X., Zhang, X.H., Zhang, W.D.: Finite-time dissipative filtering for uncertain discrete-time systems with state and disturbance-dependent noise over fading channels. ISA Trans. 86, 134–143 (2019)

    Google Scholar 

  47. Gao, X.B., Deng, F.Q.: Event-triggered finite-time reliable filtering of Markov jump nonlinear systems subject to exponential dissipativity. Int. J. Robust Nonlinear Control 31(7), 2810–2826 (2021)

    MathSciNet  Google Scholar 

  48. Wu, C.W., Li, H.Y., Lam, H.K., Karimi, H.R.: Fault detection for nonlinear networked systems based on quantization and dropout compensation: An interval type-2 fuzzy-model method. Neurocomputing 191, 409–420 (2016)

    Google Scholar 

Download references

Funding

This research was supported by the National Natural Science Foundation of China under Grant (No.61703248,61973198,62273212), Shandong Provincial Natural Science Foundation, China (No.ZR2020MF062).

Author information

Authors and Affiliations

  1. College of Mathematics and Systems Science, Shandong University of Science and Technology, Qingdao, 266590, China

    Yong Zhao, Jiaxin Luo & Xiangyun Lin

  2. College of Electrical Engineering and Automation, Shandong University of Science and Technology, Qingdao, 266590, China

    Weihai Zhang

Authors
  1. Yong Zhao
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Jiaxin Luo
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Weihai Zhang
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Xiangyun Lin
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Yong Zhao.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zhao, Y., Luo, J., Zhang, W. et al. Asynchronous Finite-Time Exponential Dissipative Filtering for Stochastic Fuzzy Markov Jump Systems Against Deception Attacks Based on Event-Triggered Scheme. Int. J. Fuzzy Syst. 26, 735–752 (2024). https://doi.org/10.1007/s40815-023-01631-w

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s40815-023-01631-w

Keywords

Search

Navigation