A hybrid numerical method to solve nonlinear parabolic partial differential equations of time-arbitrary order | Computational and Applied Mathematics Skip to main content
Springer Nature Link
Log in

A hybrid numerical method to solve nonlinear parabolic partial differential equations of time-arbitrary order

  • Published:
Computational and Applied Mathematics Aims and scope Submit manuscript

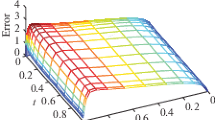
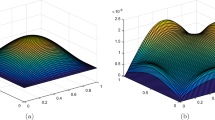
Abstract

In this study, the Quasi-Linearization Method (QLM) is combined with the collocation method, based on the bivariate generalized fractional order of the Chebyshev functions (B-GFCFs), to solve higher order nonlinear partial differential equations (NPDEs) of the evolution parabolic kind with the time-arbitrary (integer or fractional) order, where are very important because the description of various mechanisms in physics and engineering. The method is used for solving some famous NPDEs, such as the generalized Burgers–Fisher equation, the Fisher equation, the Burgers–Huxley equation, and the modified KdV–Burgers equation. First, the QLM converts the nonlinear equation to a sequence of linear partial differential equations (LPDEs), and then these LPDEs are solved using the B-GFCFs collocation method. In the time-fractional case, we use the Caputo fractional derivative operational matrix to get rid of the fractional derivative. An algorithm is presented by examining its complexity order for the method, as also, the convergence analysis, error analysis, and error estimate for the method are briefly investigated. The results in the examples are compared with the exact solutions and the methods are used in other literature to show accuracy, convergence, and effectiveness of the proposed method, and also the very good approximation solutions are obtained.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10

Similar content being viewed by others

References

  • Abbasbandy S (2007) The application of homotopy analysis method to solve a generalized Hirota-Satsuma coupled KdV equation. Phys Lett A Gen Atom Sol Phys 361(6):478–483

    MATH  Google Scholar 

  • Abdel-Gawad HI, Osman M (2014) Exact solutions of the Korteweg–de Vries equation with space and time dependent coefficients by the extended unified method. Indian J Pure Appl Math 45(1):1–12

    Article  MathSciNet  MATH  Google Scholar 

  • Abd-Elhameed WM, Youssri YH (2017) Generalized Lucas polynomial sequence approach for fractional differential equations. Nonlinear Dyn 89(2):1341–1355

    Article  MathSciNet  MATH  Google Scholar 

  • Abd-Elhameed WM, Youssri YH (2018) Fifth-kind orthonormal Chebyshev polynomial solutions for fractional differential equations. Comput Appl Math 37(3):2897–2921

    Article  MathSciNet  MATH  Google Scholar 

  • Adomian G (1983) Stochastic systems, mathematics in science and engineering, vol 169. Academic Press, Orlando

    Google Scholar 

  • Adomian G (1988) A review of the decomposition method in applied mathematics. J Math Anal Appl 135(2):501–544

    Article  MathSciNet  MATH  Google Scholar 

  • Argyris J, Haase M (1987) An engineer’s guide to soliton phenomena: application of the finite element method. Comput Method Appl Mech Eng 61(1):71–122

    Article  MathSciNet  MATH  Google Scholar 

  • Babolian E, Javadi S, Moradi E (2016) RKM for solving Bratu-type differential equations of fractional order. Math Methods Appl Sci 39(6):1548–1557

    Article  MathSciNet  MATH  Google Scholar 

  • Bellman RE, Kalaba RE (1965) Quasilinearization and nonlinear boundary-value problems. Elsevier Publishing Company, New York

    MATH  Google Scholar 

  • Bhrawy AH, Alofi AS (2013) The operational matrix of fractional integration for shifted Chebyshev polynomials. Appl Math Lett 26:25–31

    Article  MathSciNet  MATH  Google Scholar 

  • Bougoffa L, Rach RC (2013) Solving nonlocal initial-boundary value problems for linear and nonlinear parabolic and hyperbolic partial differential equations by the adomian decomposition method. Appl Math Comput 225:50–61

    MathSciNet  MATH  Google Scholar 

  • Boyd JP (2000) Chebyshev and Fourier spectral methods, 2nd edn. Dover Publications, New York

    Google Scholar 

  • Burgers JM (1948) A mathematical model illustrating the theory of turbulence. Adv Appl Mech 1:171–199

    Article  MathSciNet  Google Scholar 

  • Canuto C, Hussaini MY, Quarteroni A, Zang TA (1987) Spectral methods in fluid dynamic. Springer, New York

    MATH  Google Scholar 

  • Carey GF, Shen Y (1991) Approximations of the KdV equation by least squares finite elements. Comput Method Appl Mech Eng 93(1):1–11

    Article  MATH  Google Scholar 

  • Celik I (2012) Haar wavelet method for solving generalized Burgers–Huxley equation. Arab J Math Sci 18(1):25–37

    MathSciNet  MATH  Google Scholar 

  • Chandraker V, Awasthi A, Jayaraj S (2015) A numerical treatment of Fisher equation. Procedia Eng 127:1256–1262

    Article  Google Scholar 

  • Chun C (2008) Solitons and periodic solutions for the fifth-order KdV equation with the Exp-function method. Phys Lett A 372(16):2760–2766

    Article  MathSciNet  MATH  Google Scholar 

  • Cole JD (1951) On a quasi-linear parabolic equation occurring in aerodynamics. Q Appl Math 9:225–236

    Article  MathSciNet  MATH  Google Scholar 

  • Conte SD, de Boor C (1981) Elementary numerical analysis. McGraw-Hill International Editions, New York

    Google Scholar 

  • Delkhosh M (2013) Introduction of derivatives and integrals of fractional order and its applications. Appl Math Phys 1(4):103–119

    Google Scholar 

  • Delkhosh M, Parand K (2018) A new computational method to solve fractional differential equations: theory and applications (Submitted)

  • Djidjeli K, Price WG, Temarel P, Twizell EH (1998) A linearized implicit pseudo-spectral method for certain nonlinear water wave equations. Commun Numer Sci World J 14(10):977–993

    MATH  Google Scholar 

  • Doha EH, Bhrawy AH, Ezz-Eldien SS (2011) A Chebyshev spectral method based on operational matrix for initial and boundary value problems of fractional order. Comput Math Appl 62:2364–2373

    Article  MathSciNet  MATH  Google Scholar 

  • Duffy BR, Parkes EJ (1996) Travelling solitary wave solutions to a seventh-order generalized KdV equation. Phys Lett A 214(5–6):271–272

    Article  MathSciNet  MATH  Google Scholar 

  • Elbarbary EME, El-Kady M (2003) Chebyshev finite difference approximation for the boundary value problems. Appl Math Comput 139(2–3):513–523

    MathSciNet  MATH  Google Scholar 

  • Fan E (2002) Traveling wave solutions for nonlinear equations using symbolic computation. Comput Math Appl 43(6–7):671–680

    Article  MathSciNet  MATH  Google Scholar 

  • Golbabai A, Javidi M (2009) A spectral domain decomposition approach for the generalized Burger’s–Fisher equation. Chaos Soliton Fractal 39(1):385–392

    Article  MathSciNet  MATH  Google Scholar 

  • Hafez RM, Youssri YH (2018) Jacobi collocation scheme for variable-order fractional reaction-subdiffusion equation. Comput Appl Math 37(4):5315–5333

    Article  MathSciNet  MATH  Google Scholar 

  • Hammad DA, El-Azab MS (2015) 2N order compact finite difference scheme with collocation method for solving the generalized Burger’s–Huxley and Burger’s–Fisher equations. Appl Math Comput 258:296–311

    MathSciNet  MATH  Google Scholar 

  • Hariharan G, Kannan K, Sharma KR (2009) Haar wavelet method for solving Fisher’s equation. Appl Math Comput 211(2):284–292

    MathSciNet  MATH  Google Scholar 

  • Hashim I, Abdulaziz O, Momani S (2009) Homotopy analysis method for fractional IVPs. Commun Nonlinear Sci Numer Simul 14:674–684

    Article  MathSciNet  MATH  Google Scholar 

  • He J (2005) Application of homotopy perturbation method to nonlinear wave equations. Chaos Soliton Fractal 26(3):695–700

    Article  MATH  Google Scholar 

  • Helal MA, Mehanna MS (2006) A comparison between two different methods for solving KdV-Burgers equation. Chaos Soliton Fractal 28(2):320–326

    Article  MathSciNet  MATH  Google Scholar 

  • Kalaba R (1957) On nonlinear differential equations, the maximum operation and monotone convergence. RAND Corporation, Santa Monica, CA. https://www.rand.org/pubs/papers/P1163.html

  • Kazem S, Abbasbandy S, Kumar S (2013) Fractional-order Legendre functions for solving fractional-order differential equations. Appl Math Model 37:5498–5510

    Article  MathSciNet  MATH  Google Scholar 

  • Kilbas AA, Srivastava HM, Trujillo JJ (2006) Theory and applications of fractional differential equations. Elsevier, San Diego

    MATH  Google Scholar 

  • Krivec R, Mandelzweig VB (2008) Quasilinearization approach to computations with singular potentials. Comput Phys Commun 179(12):865–867

    Article  MathSciNet  MATH  Google Scholar 

  • Kyrychko YN, Bartuccelli MV, Blyuss KB (2005) Persistence of travelling wave solutions of a fourth order diffusion system. J Comput Appl Math 176(2):433–443

    Article  MathSciNet  MATH  Google Scholar 

  • Lakshmikantham V, Vatsala AS (1998) Generalized quasilinearization for nonlinear problems, mathematics and its applications, vol 440. Kluwer Academic Publishers, Dordrecht

    Book  MATH  Google Scholar 

  • Leibniz GW (1965) Letter from Hanover, Germany, to G.F.A. L’Hopital, September 30; 1695, in Mathematische Schriften, 1849; reprinted 1962, Olms verlag; Hidesheim, Germany, vol 2, pp 301–302

  • Lepik U (2007) Numerical solution of evolution equations by the Haar wavelet method. Appl Math Comput 185(1):695–704

    MathSciNet  MATH  Google Scholar 

  • Liao SJ (2014) Advances in homotopy analysis method. World Scientific Publishing, Singapore

    Book  MATH  Google Scholar 

  • Liverts EZ, Mandelzweig VB (2009) Analytical computation of amplification of coupling in relativistic equations with Yukawa potential. Ann Phys New York 324(2):388–407

    Article  MathSciNet  MATH  Google Scholar 

  • Mandelzweig VB, Tabakinb F (2001) Quasilinearization approach to nonlinear problems in physics with application to nonlinear ODEs. Comput Phys Commun 141:268–281

    Article  MathSciNet  MATH  Google Scholar 

  • Motsa SS, Magagula VM, Sibanda P (2014) A bivariate Chebyshev spectral collocation quasilinearization method for nonlinear evolution parabolic equations. Sci World J. https://doi.org/10.1155/2014/581987

    Article  Google Scholar 

  • Odabasi M, Misirli E (2018) On the solutions of the nonlinear fractional differential equations via the modified trial equation method. Math Methods Appl Sci 41(3):904–911

    Article  MathSciNet  MATH  Google Scholar 

  • Odibat Z, Momani S (2008) An algorithm for the numerical solution of differential equations of fractional order. J Appl Math Info 26:15–27

    MATH  Google Scholar 

  • Osman MS, Korkmaz A, Rezazadeh H, Mirzazadeh M, Eslami M, Zhou Q (2018) The unified method for conformable time fractional Schrodinger equation with perturbation terms. Chin J Phys 56(5):2500–2506

    Article  MathSciNet  Google Scholar 

  • Osman MS, Machado JAT (2018) New nonautonomous combined multi-wave solutions for (2+1)-dimensional variable coefficients KdV equation. Nonlinear Dyn 93(2):733–740

    Article  MATH  Google Scholar 

  • Osman MS, Machado JAT (2018) The dynamical behavior of mixed-type soliton solutions described by (2+1)-dimensional Bogoyavlensky–Konopelchenko equation with variable coefficients. J Electromagn Wave Appl 32(11):1457–1464

    Article  Google Scholar 

  • Parand K, Delkhosh M (2016) Operational matrices to solve nonlinear Volterra-Fredholm integro-differential equations of multi-arbitrary order. Gazi Univ J Sci 29(4):895–907

    Google Scholar 

  • Parand K, Delkhosh M (2016) Solving Volterra’s population growth model of arbitrary order using the generalized fractional order of the Chebyshev functions. Ric Mat 65(1):307–328

    Article  MathSciNet  MATH  Google Scholar 

  • Parand K, Delkhosh M (2017) Accurate solution of the Thomas-Fermi equation using the fractional order of rational Chebyshev functions. J Comput Appl Math 317:624–642

    Article  MathSciNet  MATH  Google Scholar 

  • Parand K, Delkhosh M (2017) Solving the nonlinear Schlomilch’s integral equation arising in ionospheric problems. Afr Mat 28:459–480

    Article  MathSciNet  MATH  Google Scholar 

  • Parand K, Khaleqi S (2016) The rational Chebyshev of second kind collocation method for solving a class of astrophysics problems. Eur Phys J Plus 131:1–24

    Article  Google Scholar 

  • Parand K, Rad JA (2012) Exp-function method for some nonlinear PDE’s and a nonlinear ODE’s. J King Saud Univ Sci 24:1–10

    Article  Google Scholar 

  • Parand K, Rezaei AR, Taghavi A (2010) Numerical approximations for population growth model by rational Chebyshev and Hermite functions collocation approach: a comparison. Math Methods Appl Sci 33(17):2076–2086

    Article  MathSciNet  MATH  Google Scholar 

  • Parand K, Ghasemi M, Rezazadeh S, Peiravi A, Ghorbanpour A, Tavakoli Golpaygani A (2010) Quasilinearization approach for solving Volterra’s population model. Appl Comput Math 9(1):95–103

    MathSciNet  MATH  Google Scholar 

  • Parand K, Rad JA, Rezaei A (2011) Application of exp-function method foa a class of nonlinear PDE’s arising in mathematical physics. J Appl Math Inform 29(3):763–779

    MathSciNet  MATH  Google Scholar 

  • Parand K, Abbasbandy S, Kazem S, Rezaei AR (2011) An improved numerical method for a class of astrophysics problems based on radial basis functions. Phys Scr 83(1):015011 11 pages

    Article  MATH  Google Scholar 

  • Parand K, Delkhosh M, Nikarya M (2017) Novel orthogonal functions for solving differential equations of arbitrary order. Tbilisi Math J 10(1):31–55

    Article  MathSciNet  MATH  Google Scholar 

  • Parkes EJ, Duffy BR (1996) An automated tanh-function method for finding solitary wave solutions to non-linear evolution equations. Comput Phys Commun 98(3):288–300

    Article  MATH  Google Scholar 

  • Podlubny I (1999) Fractional differential equations. Academic Press, San Diego

    MATH  Google Scholar 

  • Rad JA, Kazem S, Shaban M, Parand K, Yildirim A (2014) Numerical solution of fractional differential equations with a Tau method based on Legendre and Bernstein polynomials. Math Methods Appl Sci 37:329–342

    Article  MathSciNet  MATH  Google Scholar 

  • Rezaei A, Baharifard F, Parand K (2011) Quasilinearization-Barycentric approach for numerical investigation of the boundary value fin problem. Int J Comput Electr Autom Control Info Eng 5(2):194–201

    Google Scholar 

  • Saeed U, Rehman M (2015) Haar wavelet Picard method for fractional nonlinear partial differential equations. Appl Math Comput 264:310–322

    MathSciNet  MATH  Google Scholar 

  • Sahin A, Ozmen O (2014) Usage of higher order B-splines in numerical solution of Fishers equation. Int J Nonlinear Sci 17(3):241–253

    MathSciNet  MATH  Google Scholar 

  • Sari M, Grarslan G, Dag I (2010) A compact finite difference method for the solution of the generalized Burgers–Fisher equation. Numer Methods Partial Differ Equ 26:125–134

    Article  MathSciNet  Google Scholar 

  • Vliegenthart AC (1971) On finite-difference methods for the Korteweg–de Vries equation. J Eng Math 5:137–155

    Article  MathSciNet  MATH  Google Scholar 

  • Wazwaz AM, Osman MS (2018) Analyzing the combined multi-waves polynomial solutions in a two-layer-liquid medium. Comput Math Appl 76(2):276–283

    Article  MathSciNet  MATH  Google Scholar 

  • Wazwaz A, Gorguis A (2004) An analytic study of Fisher’s equation by using adomian decomposition method. Appl Math Comput 154(3):609–620

    MathSciNet  MATH  Google Scholar 

  • Wubs FW, deGoede ED (1992) An explicit-implicitmethod for a class of time-dependent partial differential equations. Appl Numer Math 9(2):157–181

    Article  MathSciNet  Google Scholar 

  • Youssri YH (2017) A new operational matrix of Caputo fractional derivatives of Fermat polynomials: an application for solving the Bagley–Torvik equation. Adv Differ Equ 2017:73

  • Yuksel G, Rasit Isik O, Sezer M (2015) Error analysis of the Chebyshev collocation method for linear second-order partial differential equations. Int J Comput Math 92(10):2121–2138

    Article  MathSciNet  MATH  Google Scholar 

  • Yuzbasi S (2016) A numerical method for solving second-order linear partial differential equations under Dirichlet, Neumann and Robin boundary conditions. Int J Comput Methods 14(2):20 Article ID 1750015

    MathSciNet  MATH  Google Scholar 

  • Yuzbasi S, Sahin N, Sezer M (2012) A Bessel collocation method for numerical solution of generalized pantograph equations. Numer Methods Part Differ Equ 28(4):1105–1123

    Article  MathSciNet  MATH  Google Scholar 

  • Zhao S, Wei GW (2003) Comparison of the discrete singular convolution and three other numerical schemes for solving Fisher’s equation. SIAM J Sci Comput 25:127–147

    Article  MathSciNet  MATH  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics and Computer Sciences, Bardaskan Branch, Islamic Azad University, Bardaskan, Iran

    Mehdi Delkhosh

  2. Department of Computer Sciences, Shahid Beheshti University, G.C., Tehran, Iran

    Kourosh Parand

  3. Department of Cognitive Modelling, Institute for Cognitive and Brain Sciences, Shahid Beheshti University, G.C., Tehran, Iran

    Kourosh Parand

Authors
  1. Mehdi Delkhosh
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Kourosh Parand
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Mehdi Delkhosh.

Additional information

Communicated by José Tenreiro Machado.

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Delkhosh, M., Parand, K. A hybrid numerical method to solve nonlinear parabolic partial differential equations of time-arbitrary order. Comp. Appl. Math. 38, 76 (2019). https://doi.org/10.1007/s40314-019-0840-6

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s40314-019-0840-6

Keywords

Mathematics Subject Classification

Search

Navigation