Abstract
In this article, we investigate the behavior of the coefficient of restitution (COR) which is an important parameter in many impact-related fields. In many cases, the COR is considered as a constant value, but it varies according to many variables. In this paper, we introduce an analytical variable COR model considering aerodynamics along with its verification through experiment. To introduce and analyze the variable characteristic of the COR model, the collision phenomenon between a pendulum and two kinds of ball is employed as an example and aerodynamics such as drag force is considered for analyzing the after-effect of the collision. Collision velocity of the pendulum, dynamic parameters of colliding bodies, contact time, drag coefficient, the air density, and the cross-sectional area of the ball are found as the typical variables of analytical COR model. This observation generalizes the result in previous researches. To verify new COR model, the travel distances for the curve-fitted constant COR model and the curve-fitted variable COR model are compared through simulation and experiment. Moreover, comparison between constant COR and variable COR is presented in several points of view. Finally, using the variable COR model, the travel distance of the ball for given collision velocity can be estimated.































Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.Abbreviations
- \(\tilde{\underline{F}}_\mathrm{{ext}}\) :
-
External impulse
- \({\underline{F}}_\mathrm{{ext}}\) :
-
External force
- \(\Delta t\) :
-
Contact time during collision
- e :
-
Coefficient of restitution (COR)
- n :
-
Contact time during collision
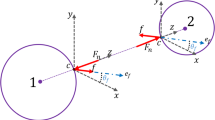
- \(v_M \) :
-
Absolute velocity of the manipulator
- \(v_B \) :
-
Absolute velocity of the environment
- \(\Delta v_M \) :
-
Velocity variation of the manipulator after collision
- \(\Delta v_B \) :
-
Velocity variation of the environment after collision
- \(F_D \) :
-
The magnitude of the drag force
- \(\rho \) :
-
Density of the air \((1.226\,\mathrm{{kg}}/\mathrm{{m}}^{3}\) in \(15\,^{\circ }\mathrm{{C}}, 1013\,\mathrm{{hpa}}\))
- S :
-
The cross-sectional area of the ball
- \(C_D \) :
-
Coefficient of the drag force
- \(v_\mathrm{{air}}\) :
-
The relative velocity of the air with respect to the ball
- \(N_\mathrm{{Red}}\) :
-
Reynolds number which is the ratio of the inertial force to the viscous force
- V :
-
The average velocity of the air (if there is no wind, \(V=v_\mathrm{{air}})\)
- D :
-
The diameter of the ball
- \(\mu \) :
-
The coefficient of the air viscosity (\(1.83\times 10^{-5}\mathrm{{kg}}/\mathrm{{m}}\,\mathrm{{s}}\) in \(15\,^{\circ }\mathrm{{C}})\)
- \(F_\mathrm{{mag}} \) :
-
The force caused by the Magnus effect
- \(C_\mathrm{{mag}}(C_L)\) :
-
The coefficient caused by the Magnus effect
- Sp :
-
Spin parameter
- w :
-
The angular velocity of the ball
- r :
-
The radius of the ball
- \(M_E \) :
-
Effective mass of the impacting pendulum at the position of impact
- M :
-
The mass attached to the distal end of the pendulum
- m :
-
The mass of the pendulum bar
- \(l(=L_E)\) :
-
The length of the pendulum bar
- \(\underline{{\varvec{\upsilon }}}_B^0\) :
-
Velocity of the ball before the impact
- \(\underline{{\varvec{\upsilon }}}_M^0\) :
-
The velocity of the pendulum before the impact
- \({\varvec{\upsilon }}_M^\mathrm{{avg}}\) :
-
The average velocity of the pendulum during the period of impact \(({=}{({\varvec{\upsilon }}_{M}^{0} +{\varvec{\upsilon }}_{M})}/2)\)
- \(h_{s}\) :
-
Height of the supporter
- \(\theta _1 \) :
-
The back swing angle for initial setting
- \(\theta _2 \) :
-
The forward swing angle after the impact (\(\theta _1 >\theta _2 )\)
- \(w_1 \) :
-
The angular velocity of the pendulum immediately before the impact
- \(w_2 \) :
-
The angular velocity of the pendulum immediately after the impact
- \(E_\mathrm{{loss}} \) :
-
Energy loss
References
Youcef-Toumi K, Gutz DA (1989) Impact and force control. In: Proceedings of the IEEE international conference on robotics and automation, pp 410–416
Walker ID (1990) The use of kinematic redundancy in reducing impact and contact effects in manipulation. In: Proceedings of the IEEE international conference on robotics and automation, pp 434–439
Walker ID (1994) Impact configurations and measures for kinematically redundant and multiple armed robot systems. IEEE Trans Robot Autom 12(5):670–683
So BR, Yi B-J, Kim WK (2002) Impulse analysis and its applications to dynamic environment. In: Proceedings of the ASME conference on biennial mechanism, pp 279–286
Stronge WJ (2003) Chain reaction from impact on aggregate of elasto-plastic rigid bodies. Int J Impact Eng 28(3):291–302
Choi JY, So BR, Yi B-J, Kim WK, Suh IH (2005) Impact based trajectory planning of a soccer ball in a kicking robot. In: Proceedings of the 2005 IEEE international conference on robotics and automation, pp 2834–2840
Choi JY, Yi B-J (2009) Dynamics and impact control of a flying soccer ball. J Korean Phys Soc 54:75–84
Greenwood DT (1988) Principles of dynamics, 2nd edn. Prentice-Hall, Upper Saddle River
Imre B (2008) A coefficient of restitution of rock materials. J Comput Geosci 34(4):339–350
American Society for Testing and Materials, Designation F1887-98, Standard Test Method for Measuring the Coefficient of Restitution (COR) of Baseballs and Softballs (2002)
Andersen TB, Sorensen H, Kristensen LB (2008) Biomechanical differences between toe and instep kicking; influence of contact area on the coefficient of restitution. J Footb Sci 5:45–50
Goff JE, Carre MJ (2009) Trajectory analysis of a soccer ball. Am J Phys 77(11):1020–1027
Wadhwa A (2012) Measuring the rebound resilience of a bouncing ball. J. Phys Educ 47(5):620–626
Chiu J-T, Shen C-L (2005) Analysis of the restitution characteristics of a golf ball colliding with a club-head. Jpn J Ind Appl Math 22(3):429–442
Hill BP (2010) A finite element method study of coefficients of restitution in Golf Driver Clubfaces. Thesis in Hartford Connecticut, pp 1–37
Kanda Y, Naruo T, Kida T (2006) Effect of a metal bat stiffness for rubber-ball baseball on coefficient of restitution. Sym. Nihon Kikai Gakkai Supotsu Kogaku Shinpojiumu, pp 94–97
Price D, Jones R, Harland A, Silberschmidt V (2008) Viscoelasticity of multi-layer textile reinforced polymer composites used in soccer balls. J Mater Sci 43(8):2833–2843
Sissler L, Jones R, Leaney PG, Harland A (2010) Viscoelastic modelling of tennis ball properties. IOP Conf Ser Mater Sci Eng 10(1):1–9
Ranga D, Strangwood M (2010) Finite element modelling of the quasi-static and dynamic behavior of a solid sports ball based on component material properties. Proc Conf Int Sports Eng Assoc 2(2):3287–3292
Aryaei A, Hashemnia K, Jafarpur K (2010) Experimental and numerical study of ball size effect on restitution coefficient in low velocity impacts. Int J Impact Eng 37(10):1037–1044
Pagilla PR, Yu B (2004) An experimental study of planar impact of a robot manipulator. IEEE/ASME Trans Mechatron 9(1):123–128
Katta RR, Polycarpou AA, Hanchi JV, Crone RM (2009) High velocity oblique impact and coefficient of restitution for head disk interface operational shock. J Tribol 131(2):021903-1–021903-9
Cross R (2007) Vertical bounce of two vertically aligned balls. Am J Phys 75(11):1009–1016
Cross R (2002) Measurements of the horizontal coefficient of restitution for a superball and a tennis ball. Am J Phys 70(5):482–489
Walton OR, Braun RL (1986) Viscosity, granular-temperature, and stress calculations for shearing assemblies of inelastic, frictional disks. J Rheol 30:949–980
Kuwabara G, Kono K (1987) Restitution coefficient in a collision between two spheres. Jpn J Appl Phys 26:1230–1233
Brody H (2003) Bounce of a tennis ball. J Sci Med Sport 6(1):113–119
Kagan D, Atkinson D (2004) The coefficient of restitution of baseballs as a function of relative humidity. Phys Teach 42:89–92
Walker J, Halliday D, Resnick R (2013) Principles of physics: extended, 10th edn. Wiley, New York
Alexandrou AN (2001) Principles of fluid mechanics. Prentice-Hall, Upper Saddle River
Cimbala John M, Cengel Yunus A (2008) Essentials of fluid mechanics: fundamentals and applications. McGraw-Hill, New York
Acknowledgments
This work was supported by the Technology Innovation Program (10040097) funded by the Ministry of Trade, Industry and Energy Republic of Korea (MOTIE, Korea), supported by Mid-career Researcher Program through NRF grant funded by the MEST(NRF-2013 R1A2A2A01068814), supported by the Technology Innovation Program (10052980, Development of microrobotic system for surgical treatment of chronic total occlusion) funded By the Ministry of Trade, Industry & Energy(MI, Korea), and supported by the Technology Innovation Program (10049789, Steering and driving mechanism for Cardio-vascular intervention procedure) funded By the Ministry of Trade, Industry & Energy (MI, Korea). This work performed by ICT-based Medical Robotic Systems Team of Hanyang University, Department of Electronic Systems Engineering was supported by the BK21 Plus Program funded by National Research Foundation of Korea (NRF).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Ryu, HT., Yi, BJ. & Kwon, Y.H. Analytical model of variable characteristic of coefficient of restitution and its application to ball trajectory planning. Intel Serv Robotics 10, 13–29 (2017). https://doi.org/10.1007/s11370-016-0206-5
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11370-016-0206-5