Adaptive Beam Deactivation for Coordinated Multi-cell System with Limited Feedback

Abstract

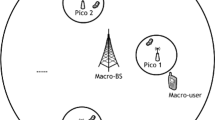
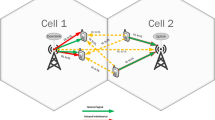
In this paper, we propose an adaptive beam deactivation scheme to mitigate inter-cell interference for a coordinated single-cell beamforming downlink transmission with limited feedback in a multi-cell multiple-input multiple-output wireless network. In the proposed scheme, each user feeds the indices of a desired beam and other undesired beams, and a certain channel quality indicator back to its home base station (BS). Based on the returned information, coordinating BSs assign each user to a serving BS by exploiting the proposed transmitting user set selection algorithm. Both exact and asymptotic sum rate analyses are done on the resulting system. Selected numerical examples are provided to show that the proposed scheme achieves noticeable sum rate gain compared to the conventional zero-forcing based coordinated multi-cell system with reduced feedback load.

Appendices

Appendix 1

It is possible to obtain the joint pdf $f_{{\mathbf{Y}}_{(i)},{\mathbf{V}}_{i+1}^{M(N-1)}}(y_{i},v_2)$ by integrating the joint pdf $f_{{\mathbf{V}}_{1}^{i-1},{\mathbf{Y}}_{(i)},{\mathbf{V}}_{i+1}^{M(N-1)}}(v_1,y_{i},v_2)$ [15, Eq. 28] with regard to $v_1$ as follows:

$$\begin{aligned}&f_{{\mathbf{Y}}_{(i)},{\mathbf{V}}_{i+1}^{M(N-1)}}(y_{i},v_2) \\& \quad=\int _{(i-1)y_i}^{\infty }f_{{\mathbf{V}}_{1}^{i-1},{\mathbf{Y}}_{(i)},{\mathbf{V}}_{i+1}^{M(N-1)}}(v_1,y_{i},v_2){{{\rm d}}}v_1 \\&\quad=\frac{[M(N-1)]!e^{-(iy_i+v_2)}}{[M(N-1)-l]![M(N-1)-i-1]!(i-1)!}\sum _{j=0}^{M(N-1)-i} \left( \begin{array}{c} M(N-1)-i \\ j \\ \end{array} \right) \\&\qquad \times (-1)^{j}(v_2-jy_i)^{M(N-1)-i-1}U(v_2-jy_i),v_2<[M(N-1)-i]y_i. \end{aligned}$$

(51)

From (51), the joint pdf $f_{{\mathbf{Y}}_{(N_d)},{\mathbf{Z}}}(y_{N_d},z)$, where ${\mathbf{Z}}=\frac{N-1}{N_a}{\mathbf{V}}_{N_d+1}^{M(N-1)}$ and $N_a=M(N-1)-N_d$, can be obtained after some modifications as

$$\begin{aligned}&f_{{\mathbf{Y}}_{(N_d)},{\mathbf{Z}}}(y_{N_d},z) \\& \quad=\frac{[M(N-1)]!e^{-\left( N_dy_{N_d}+\frac{N_a}{N-1}z\right) }}{(N_d-1)![(N_a-1)!]^2(N-1)} \sum _{i=0}^{N_a}\left( \begin{array}{c} N_a \\ i \\ \end{array} \right) (-1)^{i} \\&\qquad \times \left( \frac{N_a}{N-1}z-iy_{N_d}\right) ^{N_a-1}U\left( \frac{N_a}{N-1}z-iy_{N_d}\right) ,\,\, y_{N_d}>\frac{z}{N-1}, \end{aligned}$$

(52)

where $U(\cdot )$ is an unit step function. Now, we can obtain the pdf of Z, $f_{\mathbf{Z}}(z)$, from (52) by integrating out $y_{N_d}$, yielding

$$\begin{aligned} f_{\mathbf{Z}}(z)=\int _{\frac{z}{N-1}}^{\infty }f_{{\mathbf{Y}}_{(N_d)},{\mathbf{Z}}}(y_{N_d},z){{{\rm d}}}y_{N_d}. \end{aligned}$$

(53)

Appendix 2

Regarding the joint pdf $f_{{\mathbf{Y}}_{(i)},{\mathbf{Z}}}(y_{N_d},z)$ given in (52), we derive the joint pdf $f_{{\mathbf{Y}}_{(i)},{\mathbf{Z}}}(y_{i},z)$ for the cases of $1\le i<N_d, i=N_d+1, N_d+1<i<M(N-1)$ and $i=M(N-1)$ as follows:

• 1. Case 1: $1\le i<N_d$

  Based on Bayes’ rule, we can obtain the joint pdf $f_{{\mathbf{Y}}_{(i)},{\mathbf{Y}}_{(N_d)},{\mathbf{Z}}}(y_{i},y_{N_d},z)$ from (22) and (52) as

  $$\begin{aligned} f_{{\mathbf{Y}}_{(i)},{\mathbf{Y}}_{(N_d)},{\mathbf{Z}}}(y_{i},y_{N_d},z)=f_{{\mathbf{Y}}_{(i)}|{\mathbf{Y}}_{(N_d)}=y_{N_d}}(y_i)f_{{\mathbf{Y}}_{(N_d)},{\mathbf{Z}}}(y_{N_d},z). \end{aligned}$$

  (54)

  Then we can easily obtain the joint pdf $f_{{\mathbf{Y}}_{(i)},{\mathbf{Z}}}(y_{i},z)$ by integrating out $y_{N_d}$ as

  $$\begin{aligned} f_{{\mathbf{Y}}_{(i)},{\mathbf{Z}}}(y_{i},z)=\int _{\frac{z}{N-1}}^{y_i}f_{{\mathbf{Y}}_{(i)}|{\mathbf{Y}}_{(N_d)}=y_{N_d}}(y_i)f_{{\mathbf{Y}}_{(N_d)},{\mathbf{Z}}}(y_{N_d},z){{{\rm d}}}y_{N_d},\,\,y_i>y_{N_d}. \end{aligned}$$

  (55)

• 2. Case 2: $i=N_d+1$

  We can obtain the joint pdf $f_{{\mathbf{Y}}_{(N_d+1)},{\mathbf{Z}}}(y,z)$ by using (51) as

  $$\begin{aligned} f_{{\mathbf{Y}}_{(N_d+1)},{\mathbf{Z}}}(y,z)=\frac{N_a}{N-1}f_{{\mathbf{Y}}_{(N_d+1)},{\mathbf{V}}_{N_d+2}^{M(N-1)}}\left( y,\frac{N_az}{N-1}-y\right) , \,\, \frac{z}{N-1}<y<\frac{N_az}{N-1}. \end{aligned}$$

  (56)

• 3. Case 3: $N_d+1<i<M(N-1)$

  To derive the joint pdf $f_{{\mathbf{Y}}_{(i)},{\mathbf{Z}}}(y_{i},z)$, we first derive the joint pdf of ${\mathbf{Y}}_{(N_d)}, {\mathbf{V}}_{N_d+1}^{i-1}$ ${\mathbf{Y}}_{(i)}$, and ${\mathbf{V}}_{i+1}^{M(N-1)}$ by applying Bayes’ rule as

  $$\begin{aligned}&f_{{\mathbf{Y}}_{(N_d)},{\mathbf{V}}_{N_d+1}^{i-1},{\mathbf{Y}}_{(i)},{\mathbf{V}}_{i+1}^{M(N-1)}}(y_{N_d},v_1,y_{i},v_2) \\&\quad=f_{{\mathbf{V}}_{N_d+1}^{i-1}|{\mathbf{Y}}_{(N_d)}=y_{N_d},{\mathbf{Y}}_{(i)}=y_i}(v_1)f_{{\mathbf{Y}}_{(N_d)}|{\mathbf{Y}}_{(i)}=y_i}(y_{N_d}) \\&\qquad \times f_{{\mathbf{Y}}_{(i)},{\mathbf{V}}_{i+1}^{M(N-1)}}(y_i,v_2). \end{aligned}$$

  (57)

  The second and third terms in the right side of the expression (57) can be obtained from (22) and (51), respectively, after some modifications. The conditional pdf $f_{{\mathbf{V}}_{N_d+1}^{i-1}|{\mathbf{Y}}_{(N_d)}=y_{N_d},{\mathbf{Y}}_{(i)}=y_i}(v_1)$ can be obtained from [16, Eq. 3.55] as

  $$\begin{aligned}&f_{{\mathbf{V}}_{N_d+1}^{i-1}|{\mathbf{Y}}_{(N_d)}=y_{N_d},{\mathbf{Y}}_{(i)}=y_i}(v_1) \\&\quad=\sum ^{i-N_d-1}_{j=0}\left( \begin{array}{c} i-N_d-1 \\ j \\ \end{array}\right) \frac{(-1)^j[v_1-(i-j-N_d-1)y_i-jy_{N_d}]^{i-N_d-2}}{(i-N_d-2)!(e^{-y_i}-e^{-y_{N_d}})^{i-N_d-1}} \\&\qquad \times e^{-v_1}\cdot U(v_1-(i-j-N_d-1)y_i-jy_{N_d}),(i-N_d-1)y_i<v_1<(i-N_d-1)y_{N_d}. \end{aligned}$$

  (58)

  Therefore, we can write the joint pdf $f_{{\mathbf{Y}}_{(N_d)},{\mathbf{V}}_{N_d+1}^{i-1},{\mathbf{Y}}_{(i)},{\mathbf{V}}_{i+1}^{M(N-1)}}(\cdot ,\cdot ,\cdot ,\cdot )$ as

  $$\begin{aligned}&f_{{\mathbf{Y}}_{(N_d)},{\mathbf{V}}_{N_d+1}^{i-1},{\mathbf{Y}}_{(i)},{\mathbf{V}}_{i+1}^{M(N-1)}}(y_{N_d},v_1,y_{l},v_2)& \\&\quad=\frac{[M(N-1)]!e^{-(N_dy_{N_d}+v_1+y_i+v_2)}}{(M(N-1)-i)!(M(N-1)-i-1)!(N_d-1)!(i-N_d-1)!(i-N_d-2)!} \\&\qquad \times \sum ^{i-N_d-1}_{j=0}\sum _{k=0}^{M(N-1)-i}\left( \begin{array}{c} i-N_d-1 \\ j \\ \end{array}\right) \left( \begin{array}{c} M(N-1)-i \\ k \\ \end{array} \right) (-1)^{j+k} \\&\qquad \times [v_1-(i-j-N_d-1)y_i-jy_{N_d}]^{i-N_d-2}(v_2-ky_i)^{M(N-1)-i-1} \\&\qquad \times U(v_1-(i-j-N_d-1)y_i-jy_{N_d})U(v_2-ky_i), \\&\qquad \quad (i-N_d-1)y_i<v_1<(i-N_d-1)y_{N_d},v_2<(M(N-1)-i)y_i. \end{aligned}$$

  (59)

  After that, we derive the joint pdf $f_{{\mathbf{V}}_{N_d+1}^{i-1},{\mathbf{Y}}_{(i)},{\mathbf{V}}_{i+1}^{M(N-1)}}(v_1,y_{i},v_2)$ by integrating out $y_{N_d}$ as

  $$\begin{aligned}&f_{{\mathbf{V}}_{N_d+1}^{i-1},{\mathbf{Y}}_{(i)},{\mathbf{V}}_{i+1}^{M(N-1)}}(v_1,y_{i},v_2) \\&\quad=\int _{\frac{v_1}{i-N_d-1}}^{\infty }f_{{\mathbf{Y}}_{(N_d)},{\mathbf{V}}_{N_d+1}^{i-1},{\mathbf{Y}}_{(i)},{\mathbf{V}}_{i+1}^{M(N-1)}}(y_{N_d},v_1,y_{i},v_2){{{\rm d}}}y_{N_d}. \end{aligned}$$

  (60)

  Now we can derive the joint pdf $f_{{\mathbf{Y}}_{(i)},{\mathbf{V}}_{N_d+1}^{M(N-1)}}(y_{i},v_3)$ from (60) as

  $$\begin{aligned}&f_{{\mathbf{Y}}_{(i)},{\mathbf{V}}_{N_d+1}^{M(N-1)}}(y_{i},v_3) \\&\quad=\int _{0}^{[M(N-1)-i]y_i}f_{{\mathbf{V}}_{N_d+1}^{i-1},{\mathbf{Y}}_{(i)},{\mathbf{V}}_{i+1}^{M(N-1)}}(v_3\!-\!v_2\!-\!y_i,y_{i},v_2){{{\rm d}}}v_2, \\ \end{aligned}$$

  (61)

  where $v_2<v_3-(l-N_d)y_l$.

  Consequently, we can obtain the joint pdf $f_{{\mathbf{Y}}_{(l)},{\mathbf{Z}}}(y_l,z)$ from (61) as

  $$\begin{aligned} f_{{\mathbf{Y}}_{(i)},{\mathbf{Z}}}(y_{i},z)=\frac{N_a}{N-1}f_{{\mathbf{Y}}_{(i)},{\mathbf{V}}_{N_d+1}^{M(N-1)}}\left( y_{i},\frac{N_az}{N-1}\right) , \,\, y<\frac{N_az}{N-1}. \end{aligned}$$

  (62)

• 4. Case 4: $i=M(N-1)$

  We can obtain the joint pdf $f_{{\mathbf{Y}}_{(N_d+1)},{\mathbf{Z}}}(y,z)$ by using (16) and (58) as

  $$\begin{aligned}&f_{{\mathbf{Y}}_{(M(N-1))},{\mathbf{Z}}}(y_2,z) \\&\quad=\frac{N_a}{N-1}\int _{\frac{\frac{N_az}{N-1}-y_2}{N_a-1}}^{\infty } f_{{\mathbf{V}}_{N_d+1}^{M(N-1)-1}|{\mathbf{Y}}_{(N_d)}=y_{1},{\mathbf{Y}}_{(M(N-1))}=y_2}\left( \frac{N_az}{N-1}-y_2\right) \\&\qquad \times f_{{\mathbf{Y}}_{(N_d)},{\mathbf{Y}}_{(M(N-1))}}(y_1,y_2){{{\rm d}}}y_1,\,\frac{z}{N-1}>y_2. \end{aligned}$$

  (63)

Appendix 3

The feedback SINR of the first selected user is always the largest one among the $NK$ feedback SINRs and $p_{{\varvec{\Gamma }}^{{{\rm out}}}_1}({{{\mathcal {S}}}_{i}^{{}_{{{\rm out}}}}},NK,1)$ can be obtained easily as in (32). On the other hand, if the number of users in the user set ${{\mathcal {U}}}_2$ (or ${{\mathcal {Q}}}$ for the second user selection in Step 3 and Step 4d of the proposed algorithm) is $k(=1,\ldots ,K)$, then the feedback SINR of the second user is the second one to $(NK\!-k\!+1)$-th largest feedback SINRs. The probability that the feedback SINR of the second user is to be $l$-th $(=2,\ldots ,NK\!-k\!+1)$ largest one among $NK$ ones is given as $\frac{{}_{(NK\!-l)}{{\mathcal {C}}}_{(k-1)}}{{}_{(NK\!-\!1)}{{\mathcal {C}}}_{k}}$.

The probability that the number of users in the user set ${{\mathcal {U}}}_2$ in Step 2 of the proposed algorithm is $k$ is ${}_{K}{{\mathcal {C}}}_{k}\left( \left( \frac{M-N_d}{M}\right) ^2\right) ^k\left( 1-\left( \frac{M-N_d}{M}\right) ^2\right) ^{K-k}$. In this case, the interference term in the output SINR of the second selected user is ${\mathbf{Y}}_{j}$ $(N_d<j\le M(N-1))$. However, the probability of the number of users in ${{\mathcal {Q}}}$ for the second user selection of Step 4d to be $k$ can be formulated as follows. Note that each of the K users wants one in the undesired beam set of the first selected user or the desired beam of the first selected user.

• 1. Among the $K$ users, only the desired beams of the $k$ users, each with the desired beam of the first selected user in its undesired beam set, are not included in the undesired beam set of the first selected user. In this case, the probability that the number of the users in ${{\mathcal {Q}}}$ is $k$ is ${}_{K}{{\mathcal {C}}}_{k}\left( \left( \frac{M-N_d}{M}\right) \frac{N_d}{M}\right) ^k\left( \frac{N_d}{M}\right) ^{K-k}$ and the interference term in the output SINR of the second selected user is ${\mathbf{Y}}_j$ $(1\le j\,\le\,N_d)$.

• 2. Among the $K$ users, only the desired beams of $k$ users, each without the desired beam of the first selected user in its undesired beam set, are included in the undesired beam set of the first selected user. In addition, each of the other $K-k$ users desires the one of the beam in the undesired beam set of the first selected user and undesires the desired beam of the first selected user. In this case, the probability that the number of the users in ${{\mathcal {Q}}}$ is $k$ is ${}_{K}{{\mathcal {C}}}_{k}\left( \left( \frac{M-N_d}{M}\right) \frac{N_d}{M}\right) ^k\left( \frac{N_d}{M}\right) ^{2(K-k)}$ and the interference term in the output SINR of the second selected user is ${\mathbf{Y}}_j$ $(N_d<j\,\le\,M(N-1))$.

• 3. Each of all the K users desires the one of the beam in the undesired beam set of the first selected user and undesires the desired beam of the first selected user. In this case, the probability is $\left( \frac{N_d}{M}\right) ^{2K}$ and the interference term in the output SINR of the second selected user is ${\mathbf{Y}}_j$ $(1\le j \le N_d)$.

Consequently, we can obtain $p_{{\varvec{\Gamma }}^{{{\rm out}}}_2}({{{\mathcal {S}}}_{i}^{{}_{{{\rm out}}}}},k,l)$ as in (33).