Abstract
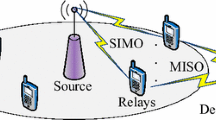
In this paper, we apply the classical Alamouti coding technique in a distributed and cascaded fashion to multihop relay networks where relays perform the decode-and-forward (DF) protocol at each relay stage. The considered system consists of two antennas at the source, two single-antenna relays at each relay stage and one antenna at the destination. The source transmits at each coding step two symbols according to the Alamouti code, to the first relay stage where each relay decodes the two symbols independently. The two relays of each relay stage behave like the two antennas of the source and transmit the two estimated symbols to the next relay stage until the destination using distributed Alamouti code. We obtain a closed form expression for the outage probability of the N-hop relay network and derive a tight analytical upper bound for the bit error probability at the destination, by considering the two-hop case firstly and then generalizing for the N-hop networks. We also show that DF protocol provides better bit error performance compared to the amplify-and-forward protocol previously considered in the literature for N-hop relay networks.




Similar content being viewed by others
References
Laneman, J. N., Tse, D. N. C., & Wornell, G. W. (2004). Cooperative communication in wireless networks: Efficient protocols and outage behaviour. IEEE Transactions on Information Theory, 50(12), 3062–3080.
Laneman, J. N., Tse, D. N. C., & Wornell, G. W. (2003). Distributed space–time coded protocols for exploiting cooperative diversity in wireless networks. IEEE Transactions on Information Theory, 49(10), 2415–2425.
Boyer, J., Falconer, D. D., & Yanikomeroglu, H. (2004). Multihop diversity in wireless relaying channels. IEEE Transactions on Communications, 52(10), 1820–1830.
Farhadi, G., & Beaulieu, N. C. (2007). Selective decode-and-forward relaying scheme for multi-hop diversity transmission systems. IEEE global telecommunications conference (GLOBECOM) (pp. 4385–4390).
Morgado, E., Mora-Jimnez, I., Vinagre, J. J., Ramos, J., & Caamao, A. J. (2010). End-to-end average BER in multihop wireless networks over fading channels. IEEE Transactions on Wireless Communications, 9(8), 2478–2487.
Ikki, S. S., & Assa, S. (2012). Multihop wireless relaying systems in the presence of cochannel interferences: Performance analysis and design optimization. IEEE Transactions on Vehicular Technology, 61(2), 566–573.
Soithong, T., Aalo, V. A., Efthymoglou, G. P., & Chayawan, C. (2011). Performance of multihop relay systems with co-channel interference in Rayleigh fading channels. IEEE Communications Letters, 15(8), 836–838.
Soithong, T., Aalo, V. A., Efthymoglou, G. P., & Chayawan, C. (2012). Outage analysis of multihop relay systems in interference-limited Nakagami-m fading channels. IEEE Transactions on Vehicular Technology, 61(3), 1451–1457.
Tam, N. T. B., Tran-Thien, T., Do-Hong, T., & Bao, V. N. Q. (2010). Performance analysis of decode-and-forward relaying for multi-hop alamouti transmission over Rayleigh fading channels. In Proceedings of international conference on advanced technology for communications (pp. 195–200).
Yindi, J., & Hassibi, B. (2006). Distributed space–time coding in wireless relay networks. IEEE Transactions on Wireless communications, 5(12), 3524–3536.
Vaze, R., & Heath, R. W. (2008). Maximizing reliability in multi-hop wireless networks. In IEEE international symposium on information theory (pp. 11–15).
Vaze, R., & Heath, R. W. (2013). Cascaded orthogonal spacetime block codes for wireless multi-hop relay networks. EURASIP Journal on Wireless Communications and Networking. doi:10.1186/1687-1499-2013-113.
Yuen, C., Chin, W. H., Guan, Y. L., Chen, W., & Tee, T. (2008). Bi-directional multi-antenna relay communications with wireless network coding. In IEEE vehicular technology conference (pp. 1385–1388).
Eslamifar, M., Yuen, C., Chin, W. H., & Guan, Y. L. (2010). Max–min antenna selection for bi-directional multi-antenna relaying. In IEEE vehicular technology conference (pp. 1–5).
Eslamifar, M., Chin, W. H., Yuen, C., & Guan, Y. L. (2012). Performance analysis of two-step bi-directional relaying with multiple antennas. IEEE Transactions on Wireless communications, 11(12), 4237–4242.
Duong, T. Q., Yuen, C., Zepernick, H., & Lei, X. (2010). Average sum-rate of distributed alamouti space–time scheme in two-way amplify-and-forward relay networks. In IEEE global telecommunications conference (GLOBECOM) (pp. 79–83).
Kuek, S. K., Yuen, C., & Chin, W. H. (2008). Four-node relay network with bi-directional traffic employing wireless network coding with pre-cancellation. In IEEE vehicular technology conference (pp. 1201–1205).
Scutari, G., & Barbarossa, S. (2005). Distributed space–time coding for regenerative relay networks. IEEE Transactions on Information Theory, 4(5), 2387–2399.
Anghel, P. A., & Kaveh, M. (2006). Distributed space–time cooperative systems with regenerative relays. IEEE Transactions on Wireless Communications, 5(11), 3130–3141.
Alamouti, S. (1998). A simple transmit diversity technique for wireless communications. IEEE Journal on Selected Areas in Communications, 16(8), 1451–1458.
Simon, M. K., & Alouini, M. S. (2005). Digital communications over fading channels (2nd ed.). London: Wiley.
Lee, H., Andrews, J. G., & Powers, E. J. (2008). Information outage probability and diversity order of symmetric coordinate interleaved orthogonal designs. IEEE Transactions on Wireless Communications, 7(5), 1501–1506.
Author information
Authors and Affiliations
Corresponding author
Appendix
Appendix
The diversity order is obtained by [22]
where the outage probability is given by
After some manipulation, we get
We cannot find the limit directly, so we need to use Taylor series expansion to have a proper expression. We know that the Taylor series expansions are given by
where \(O(\cdot)\) represents omitted higher-order terms in power series. When we use (57) in (56) we have
where \(C_1\) is a constant. Then we have
So it is proved that the diversity order of the system is 2. \(\square\)
Rights and permissions
About this article
Cite this article
Aydın, İ., Aygölü, Ü. Performance analysis of a multihop relay network using distributed Alamouti code. Wireless Netw 21, 217–226 (2015). https://doi.org/10.1007/s11276-014-0781-3
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11276-014-0781-3