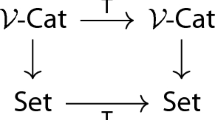Abstract
Looking at the operation of forming neat \(\alpha \)-reducts as a functor, with \(\alpha \) an infinite ordinal, we investigate when such a functor obtained by truncating \(\omega \) dimensions, has a right adjoint. We show that the neat reduct functor for representable cylindric algebras does not have a right adjoint, while that of polyadic algebras is an equivalence. We relate this categorial result to several amalgamation properties for classes of representable algebras. We show that the variety of cylindric algebras of infinite dimension, endowed with the merry go round identities, fails to have the amalgamation property answering a question of Németi’s. We also study two variants of the so-called cylindric polyadic algebras introduced by Ferenczi (all are reducts of polyadic equality algebras, that are also varieties). We show that one is more cylindric than polyadic, and that the other is more polyadic than cylindric. Our classification is determined by results on neat embeddings and amalgamation expressed from the point of view of category theory, thereby witnessing, and, indeed, further emphasizing, the dichotomy between the cylindric and polyadic paradigms. For example, the first class does not have the unique neat embedding property, fails to have the amalgamation property and the neat reduct functor does not have a right adjoint, while the second class has the unique neat emdedding property, the superamalgamation property and the neat reduct functor is strongly invertible. Other results, like first order definability of the class of neat reducts and the class of completely representable algebras, confirming our classification along these lines are presented.

Similar content being viewed by others
References
Andréka, H., Ferenczi, M., & Németi, I. (Eds.). (2012). Cylindric-like algebras and algebraic logic. Berlin: Bolyai Society Mathematical Studies and Springer-Verlag.
Andréka, H., Németi, I., & Sayed-Ahmed, T. (2013). A non-representable quasi-polyadic equality algebra with a representable cylindric reduct. Studia Mathematica Hungarica, 50, 1–16.
Daigneault, A. (1963). Freedom in polyadic algebras and two theorems of Beth and Craig. Michigan Mathematical Journal, 11, 129–135.
Daigneault, A., & Monk, J. D. (1963). Representation theory for polyadic algebras. Fundamenta Mathematicae, 52, 151–176.
Ferenczi, M. (2007a). Finitary polyadic algebras from cylindric algebras. Studia Logica, 87(1), 1–11.
Ferenczi, M. (2007b). On cylindric algebras satisfying the merry-go-round properties. Logic Journal of IGPL, 15(2), 183–199.
Ferenczi, M. (2012a). The polyadic generalization of the Boolean axiomatization of fields of sets. Transactions of the American Mathematical Society, 364(2), 867–886.
Ferenczi, M. (2012b). A new representation theory for cylindric-like algebras. In Cylindric-like algebras and algebraic logic. Berlin: Bolyai Mathematical Society and Springer-Verlag.
Halmos, P. (1962). Algebraic logic. New York: Chelsea Publishing Co.
Henkin, L., Monk, J. D., & Tarski, A. (1971). Cylindric algebras. Part I. Amsterdam: North Holland.
Henkin, L., Monk, J. D., & Tarski, A. (1985). Cylindric algebras. Part II. Amsterdam: North Holland.
Herrlich, H., & Strecker, G. E. (1973). Category theory—An introduction (p. 55). Boston, MA: Allyn and Bacon.
Hirsch, R., & Hodkinson, I. (1997). Complete representations in algebraic logic. Journal of Symbolic Logic, 62, 816–847.
Hirsch, R., & Sayed-Ahmed, T. (2013). The neat embedding problem for other algebras and for infinite dimension. Journal of Symbolic Logic (accepted).
Johnson, J. S. (1970). Amalgamation of polyadic algebras. Transactions of the American Mathematical Society, 149, 627–652.
Keisler, H. J. (1963). A complete first order logic with infinitary predicates. Fundamenta Mathematicae, 52, 177–203.
Madárasz, J., & Sayed-Ahmed, T. (2007). Amalgamation, interpolation and epimorphisms. Algebra Universalis, 56(2), 179–210.
Madarász, J., & Sayed-Ahmed, T. (2012). Amalgamation, interpolation and epimorphisms in algebraic logic. In Cylindric-like algebras and algebraic logic (pp. 91–103). Berlin: Bolyai Mathematical Society and Springer-Verlag.
Marx, I. (1995). Algebraic relativization and arrow logic. Ph.D. Thesis, ILLC Dissertation Series.
Németi, I., & Sági, G. (2000). On the equational theory of representable polyadic algebras. Journal of Symbolic Logic, 65(3), 1143–1167.
Pigozzi, D. (1971). Amalgmation, congruence extension, and interpolation properties in algebras. Algebra Universalis, 1, 269–349.
Sain, I. (2000). Searching for a finitizable algebraization of first order logic. Logic Journal of IGPL, 4, 495–589.
Sági, G. (2011). On non representable \(G\)-polyadic algebras with representable cylindric reduct. Logic Journal of IGPL, 19(1), 105–109.
Sági, G. (2012). Polyadic algebras. In Cylindric-like algebras and algebraic logic pp. (367–392). Berlin: Bolyai Mathematical Society and Springer-Verlag.
Sági, G., & Ferenczi, M. (2006). On some developments in the representation theory of cylindric-like algebras. Algebra Universalis, 55(2–3), 345–353.
Sági, G., & Shelah, S. (2006). Weak and Strong interpolation for algebraic logic. Journal of Symbolic Logic, 71, 104–118.
Sayed-Ahmed, T. (2001). The class of neat reducts is not elementary. Logic Journal of IGPL, 9, 593–628.
Sayed-Ahmed, T. (2004). On amalgamation of reducts of polyadic algebras. Algebra Universalis, 51, 301–359.
Sayed-Ahmed, T. (2005). Algebraic logic, where does it stand today? Bulletin of Symbolic Logic, 11(4), 465–516.
Sayed-Ahmed, T. (2006). The class of infinite dimensional quasi-polyadic algebras is not axiomatizable. Mathematical Logic Quarterly, 52, 106–112.
Sayed-Ahmed, T. (2007). An interpolation theorem for first order logic with infinitary predicates. Logic Journal of IGPL, 15(1), 21–32.
Sayed-Ahmed, T. (2009). On neat embeddings of cylindric algebras. Mathematical Logic Quarterly, 55(6), 666–668.
Sayed-Ahmed, T. (2010a). The class of polyadic algebras has the superamalgmation property. Mathematical Logic Quarterly, 56(1), 83–112.
Sayed-Ahmed, T. (2010). Some results about neat reducts. Algebra Universalis, 1(2010), 17–36.
Sayed-Ahmed, T. (2011). Algebras without the amalgamation property. Logic Journal of IGPL, 19(1), 87–104.
Sayed-Ahmed, T. (2012a). Neat reducts and neat embeddings in cylindric algebras. In Cylindric-like algebras and algebraic logic. Berlin: Bolayi Mathematical Society and Springer-Verlag.
Sayed-Ahmed, T. (2012b). Amalgamation in universal algebraic logic. Studia Mathematica Hungarica, 49(1), 26–43.
Sayed-Ahmed, T. (2013). The class of quasi-polyadic equality algebras is not finitely axiomatizable over its diagonal free reducts. Notre Dame Journal of Formal Logic (revised and re-submitted).
Sayed-Ahmed, T., & Németi, I. (2001). On neat reducts of algebras of logic. Studia Logica, 62(2), 229–262.
Acknowledgments
This paper was presented in the conference ‘Logic and Relativity’ held in Budapest in September 2012 in honour of Istvan Németi turning 70. I dedicate it to my mentor Professor Istvan Németi, and my present is an answer to a question of his. The variety of cylindric algebras of infinite dimensions endowed with the merry go round identities fails to have the amalgamation property. I came to know about this question back in Budapest in 2006, when together with Professor Hajnal Andréka we were discussing the open questions in Pigozzi (1971). Professor Németi had a copy of Pigozzi’s paper, that was obviously excessively used and re-used with question marks scattered all over. Miraculously I was able to obtain a photocopy, which I still have, with Istvan Németi’s questions in his own writing still there, but barely. One of my results here settles one of these scribbled question marks. Many more of Németi’s scribbled question marks were settled in my dissertation under his supervision, and some other question marks were jointly settled with Judit Madarász, witness Madárasz and Sayed-Ahmed (2007).
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Sayed-Ahmed, T. Neat embeddings as adjoint situations. Synthese 192, 2223–2259 (2015). https://doi.org/10.1007/s11229-013-0344-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11229-013-0344-7