Validation of the 3D AMR SIP–CESE Solar Wind Model for Four Carrington Rotations | Solar Physics
Skip to main content
Springer Nature Link
Log in

Validation of the 3D AMR SIP–CESE Solar Wind Model for Four Carrington Rotations

  • Published:
Solar Physics Aims and scope Submit manuscript

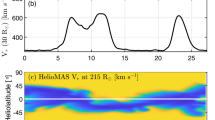
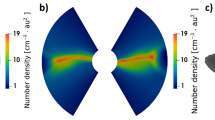
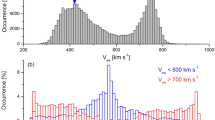
Abstract

We carry out the adaptive mesh refinement (AMR) implementation of our solar–interplanetary space-time conservation element and solution element (CESE) magnetohydrodynamic model (SIP–CESE MHD model) using a six-component grid system (Feng, Zhou, and Wu, Astrophys. J. 655, 1110, 2007; Feng et al., Astrophys. J. 723, 300, 2010). By transforming the governing MHD equations from the physical space (x,y,z) to the computational space (ξ,η,ζ) while retaining the form of conservation (Jiang et al., Solar Phys. 267, 463, 2010), the SIP–AMR–CESE MHD model is implemented in the reference coordinates with the aid of the parallel AMR package PARAMESH available at http://sourceforge.net/projects/paramesh/ . Meanwhile, the volumetric heating source terms derived from the topology of the magnetic-field expansion factor and the minimum angular separation (at the photosphere) between an open-field foot point and its nearest coronal-hole boundary are also included. We show the preliminary results of applying the SIP–AMR–CESE MHD model for simulating the solar-wind background of different solar-activity phases by comparison with SOHO observations and other spacecraft data from OMNI. Our numerical results show overall good agreements in the solar corona and in interplanetary space with these multiple-spacecraft observations.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Figure 1
Figure 2
Figure 3
Figure 4
Figure 5
Figure 6

Similar content being viewed by others

References

  • Abramenko, V., Yurchyshyn, V., Linker, J., Mikić, Z., Luhmann, J., Lee, C.O.: 2010, Low-latitude coronal holes at the minimum of the 23rd solar cycle. Astrophys. J. 712, 813.

    Article  ADS  Google Scholar 

  • Arge, C.N., Pizzo, V.J.: 2000, Improvement in the prediction of solar wind conditions using near-real time solar magnetic field updates. J. Geophys. Res. 105, 10465.

    Article  ADS  Google Scholar 

  • Arge, C.N., Odstrcil, D., Pizzo, V.J., Mayer, L.R.: 2003, Improved method for specifying solar wind speed near the Sun. In: Velli, M., Bruno, R., Malara, F., Bucci, B. (eds.) Solar Wind Ten, AIP Conf. Ser. 679, 190.

    Google Scholar 

  • Aschwanden, M.J., Burlaga, L.F., Kaiser, M.L., Ng, C.K., Reames, D.V., Reiner, M.J., Gombosi, T.I., Lugaz, N., Manchester, W., Roussev, I.I., Zurbuchen, T.H., Farrugia, C.J., Galvin, A.B., Lee, M.A., Linker, J.A., Mikić, Z., Riley, P., Alexander, D., Sandman, A.W., Cook, J.W., Howard, R.A., Odstrčil, D., Pizzo, V.J., Kóta, J., Liewer, P.C., Luhmann, J.G., Inhester, B., Schwenn, R.W., Solanki, S.K., Vasyliunas, V.M., Wiegelmann, T., Blush, L., Bochsler, P., Cairns, I.H., Robinson, P.A., Bothmer, V., Kecskemety, K., Llebaria, A., Maksimovic, M., Scholer, M., Wimmer-Schweingruber, R.F.: 2008, Theoretical modeling for the STEREO mission. Space Sci. Rev. 136, 565.

    Article  ADS  Google Scholar 

  • Baumann, I., Schmitt, D., Schüssler, M.: 2006, A necessary extension of the surface flux transport model. Astron. Astrophys. 446, 307.

    Article  ADS  Google Scholar 

  • Bilenko, I.A.: 2002, Coronal holes and the solar polar field reversal. Astron. Astrophys. 396, 657.

    Article  ADS  Google Scholar 

  • Bridges, T.J.: 2008, Conservation laws in curvilinear coordinates: A short proof of Vinokur’s theorem using differential forms. Appl. Math. Comput. 202, 882.

    Article  MathSciNet  MATH  Google Scholar 

  • Cameron, R.H., Jiang, J., Schmitt, D., Schüssler, M.: 2010, Surface flux transport modeling for solar cycles 15-21: Effects of cycle-dependent tilt angles of sunspot groups. Astrophys. J. 719, 264.

    Article  ADS  Google Scholar 

  • Charbonneau, P.: 2005, Dynamo models of the solar cycle. Living Rev. Solar Phys. 2, 2. http://solarphysics.livingreviews.org/Articles/Irsp-2010-3/ .

    ADS  Google Scholar 

  • Cohen, O., Sokolov, I.V., Roussev, I.I., Gombosi, T.I.: 2008, Validation of a synoptic solar wind model. J. Geophys. Res. 113, A03104.

    Article  Google Scholar 

  • Colella, P., Graves, D.T., Keen, N.D., Ligocki, T.J., Martin, D.F., McCorquodale, P.W., Modiano, D., Schwartz, P.O., Sternberg, T.D., Van Straalen, B.: 2007, Chombo software package for AMR applications design document. Technical report, Applied Numerical Algorithms Group, NERSC Division, Lawrence Berkeley National Laboratory Berkeley.

  • Cranmer, S.R.: 2010, An efficient approximation of the coronal heating rate for use in global Sun-heliosphere simulations. Astrophys. J. 710, 676.

    Article  ADS  Google Scholar 

  • Cranmer, S.R., Matthaeus, W.H., Breech, B.A., Kasper, J.C.: 2009, Empirical constraints on proton and electron heating in the fast solar wind. Astrophys. J. 702, 1604.

    Article  ADS  Google Scholar 

  • De Zeeuw, D.L.: 1993, A quadtree-based adaptively refined Cartesian-grid algorithm for solution of the Euler equations. PhD thesis, Ann Arbor, MI, USA. UMI Order No. GAX94-09674.

  • Dryer, M.: 2007, Space weather simulation in 3D MHD from the Sun to the Earth and beyond to 100 AU: A modeler’s perspective of the present state of the art. Asian J. Phys. 16, 97.

    Google Scholar 

  • Feng, X., Zhou, Y., Wu, S.T.: 2007, A novel numerical implementation for solar wind modeling by the modified conservation element/solution element method. Astrophys. J. 655, 1110.

    Article  ADS  Google Scholar 

  • Feng, X.S., Xiang, C.Q., Zhong, D.K.: 2011, The state-of-art of three-dimensional numerical study for corona-interplanetary process of solar storms. Sci. Sin.-Terr. 41, 1 (in Chinese).

    Google Scholar 

  • Feng, X., Yang, L., Xiang, C., Wu, S.T., Zhou, Y., Zhong, D.: 2010, Three-dimensional solar wind modeling from the Sun to Earth by a SIP–CESE MHD model with a six-component grid. Astrophys. J. 723, 300.

    Article  ADS  Google Scholar 

  • Feng, X., Zhang, S., Xiang, C., Yang, L., Jiang, C., Wu, S.T.: 2011, A hybrid solar wind model of the CESE+HLL method with a Yin-Yang overset grid and an AMR grid. Astrophys. J. 734, 50.

    Article  ADS  Google Scholar 

  • Fisk, L.A., Schwadron, N.A., Zurbuchen, T.H.: 1999, Acceleration of the fast solar wind by the emergence of new magnetic flux. J. Geophys. Res. 104, 19765.

    Article  ADS  Google Scholar 

  • Fryxell, B., Olson, K., Ricker, P., Timmes, F.X., Zingale, M., Lamb, D.Q., MacNeice, P., Rosner, R., Truran, J.W., Tufo, H.: 2000, FLASH: An adaptive mesh hydrodynamics code for modeling astrophysical thermonuclear flashes. Astrophys. J. Suppl. Ser. 131, 273.

    Article  ADS  Google Scholar 

  • Garaizar, X., Hornung, R., Kohn, S.: 1999, Structured adaptive mesh refinement applications infracture. Technical report, Lawrence Livermore National Laboratory.

  • Gombosi, T.I., De Zeeuw, D.L., Powell, K.G., Ridley, A.J., Sokolov, I.V., Stout, Q.F., Tóth, G.: 2003, Adaptive mesh refinement for global magnetohydrodynamic simulation. In: Büchner, J., Dum, C., Scholer, M. (eds.) Space Plasma Simulation, Lecture Notes in Physics 615, Springer, Berlin, 247.

    Chapter  Google Scholar 

  • Harvey, K.L., Recely, F.: 2002, Polar coronal holes during cycles 22 and 23. Solar Phys. 211, 31.

    Article  ADS  Google Scholar 

  • Hayashi, K.: 2005, Magnetohydrodynamic simulations of the solar corona and solar wind using a boundary treatment to limit solar wind mass flux. Astrophys. J. Suppl. Ser. 161, 480.

    Article  ADS  Google Scholar 

  • Hayashi, K., Zhao, X.P., Liu, Y.: 2008, MHD simulations of the global solar corona around the Halloween event in 2003 using the synchronic frame format of the solar photospheric magnetic field. J. Geophys. Res. 113, 7104.

    Article  Google Scholar 

  • Hoeksema, J.T.: 2009, Evolution of the large-scale magnetic field over three solar cycles. In: Kosovichev, A.G., Andrei, A.H., Roelot, J.P. (eds.) IAU Symposium 264, Cambridge University Press, Cambridge, 222.

    Google Scholar 

  • Hoeksema, J.T., Wilcox, J.M., Scherrer, P.H.: 1983, The structure of the heliospheric current sheet: 1978–1982. J. Geophys. Res. 88, 9910.

    Article  ADS  Google Scholar 

  • Jiang, C., Feng, X., Zhang, J., Zhong, D.: 2010, AMR simulations of magnetohydrodynamic problems by the CESE method in curvilinear coordinates. Solar Phys. 267, 463.

    Article  ADS  Google Scholar 

  • Linde, T.: 2002, MHD simulations with the FLASH code. APS Meeting Abstracts, F3005.

  • Lionello, R., Linker, J.A., Mikić, Z.: 2009, Multispectral emission of the Sun during the first whole Sun month: Magnetohydrodynamic simulations. Astrophys. J. 690, 902.

    Article  ADS  Google Scholar 

  • Lugaz, N., Downs, C., Shibata, K., Roussev, I.I., Asai, A., Gombosi, T.I.: 2011, Numerical investigation of a coronal mass ejection from an Anemone active region: Reconnection and deflection of the 2005 August 22 eruption. Astrophys. J. 738, 127.

    Article  ADS  Google Scholar 

  • Luhmann, J.G., Lee, C.O., Li, Y., Arge, C.N., Galvin, A.B., Simunac, K., Russell, C.T., Howard, R.A., Petrie, G.: 2009, Solar wind sources in the late declining phase of cycle 23: Effects of the weak solar polar field on high speed streams. Solar Phys. 256, 285.

    Article  ADS  Google Scholar 

  • MacNeice, P., Olson, K.M., Mobarry, C., de Fainchtein, R., Packer, C.: 2000, Paramesh: A parallel adaptive mesh refinement community toolkit. Comput. Phys. Commun. 126, 330.

    Article  ADS  MATH  Google Scholar 

  • Marder, B.: 1987, A method for incorporating Gauss’ law into electromagnetic PIC codes. J. Comput. Phys. 68, 48.

    Article  ADS  MATH  Google Scholar 

  • McComas, D.J., Elliott, H.A., Gosling, J.T., Reisenfeld, D.B., Skoug, R.M., Goldstein, B.E., Neugebauer, M., Balogh, A.: 2002, Ulysses’ second fast-latitude scan: Complexity near solar maximum and the reformation of polar coronal holes. Geophys. Res. Lett. 29, 1290.

    Article  ADS  Google Scholar 

  • McComas, D.J., Elliott, H.A., Schwadron, N.A., Gosling, J.T., Skoug, R.M., Goldstein, B.E.: 2003, The three-dimensional solar wind around solar maximum. Geophys. Res. Lett. 30, 1517.

    Article  ADS  Google Scholar 

  • McComas, D.J., Elliott, H.A., Gosling, J.T., Skoug, R.M.: 2006, Ulysses observations of very different heliospheric structure during the declining phase of solar activity cycle 23. Geophys. Res. Lett. 33, 9102.

    Article  Google Scholar 

  • Mignone, A., Bodo, G., Massaglia, S., Matsakos, T., Tesileanu, O., Zanni, C., Ferrari, A.: 2007, PLUTO: A numerical code for computational astrophysics. Astrophys. J. Suppl. Ser. 170, 228.

    Article  ADS  Google Scholar 

  • Mikić, Z., Linker, J.A., Schnack, D.D., Lionello, R., Tarditi, A.: 1999, Magnetohydrodynamic modeling of the global solar corona. Phys. Plasmas 6, 2217.

    Article  ADS  Google Scholar 

  • Nakamizo, A., Tanaka, T., Kubo, Y., Kamei, S., Shimazu, H., Shinagawa, H.: 2009, Development of the 3-D MHD model of the solar corona–solar wind combining system. J. Geophys. Res. 114, 7109.

    Article  Google Scholar 

  • Odstrcil, D., Pizzo, V.J.: 1999, Distortion of the interplanetary magnetic field by three-dimensional propagation of coronal mass ejections in a structured solar wind. J. Geophys. Res. 104, 28225.

    Article  ADS  Google Scholar 

  • Olson, K.: 2006, Paramesh: A parallel adaptive grid tool. In: James, M., Gunther, B., Nobuyuki, S., Akin, E., Anil, D. (eds.) Parallel Computational Fluid Dynamics 2005: Theory and Applications: Proceedings of the Parallel CFD Conference, Elsevier, Amsterdam, 341.

    Google Scholar 

  • Owens, M.J., Crooker, N.U.: 2006, Coronal mass ejections and magnetic flux buildup in the heliosphere. J. Geophys. Res. 111, A10104.

    Article  ADS  Google Scholar 

  • Owens, M.J., Spence, H.E., McGregor, S., Hughes, W.J., Quinn, J.M., Arge, C.N., Riley, P., Linker, J., Odstrcil, D.: 2008, Metrics for solar wind prediction models: Comparison of empirical, hybrid, and physics-based schemes with 8 years of l1 observations. Space Weather 6, S08001.

    Article  Google Scholar 

  • Parashar, M.: 2007, Grace grid adaptive computational engine. Technical report, Rutgers University.

  • Pätzold, M., Tsurutani, B.T., Bird, M.K.: 1997, An estimate of large-scale solar wind density and velocity profiles in a coronal hole and the coronal streamer belt. J. Geophys. Res. 102, 24151.

    Article  ADS  Google Scholar 

  • Porfir’eva, G.A., Yakunina, G.V., Delone, A.B., Oreshina, A.V., Oreshina, I.V.: 2009, Coronal streamers on the Sun and their physical properties. J. Phys. Stud. 13, 2901.

    Google Scholar 

  • Powell, K.G., Roe, P.L., Linde, T.J., Gombosi, T.I., de Zeeuw, D.L.: 1999, A solution-adaptive upwind scheme for ideal magnetohydrodynamics. J. Comput. Phys. 154, 284.

    Article  MathSciNet  ADS  MATH  Google Scholar 

  • Rendleman, C.A., Beckner, V.E., Lijewski, M., Crutchfield, W., Bell, J.B.: 2000, Parallelization of structured, hierarchical adaptive mesh refinement algorithms. Comput. Vis. Sci. 3, 147.

    Article  MATH  Google Scholar 

  • Riley, P.: 2007, An alternative interpretation of the relationship between the inferred open solar flux and the interplanetary magnetic field. Astrophys. J. Lett. 667, L97.

    Article  ADS  Google Scholar 

  • Riley, P., Linker, J.A., Mikić, Z., Lionello, R., Ledvina, S.A., Luhmann, J.G.: 2006, A comparison between global solar magnetohydrodynamic and potential field source surface model results. Astrophys. J. 653, 1510.

    Article  ADS  Google Scholar 

  • Riley, P., Lionello, R., Linker, J.A., Mikic, Z., Luhmann, J., Wijaya, J.: 2011, Global MHD modeling of the solar corona and inner heliosphere for the whole heliosphere interval. Solar Phys. 274, 361.

    Article  ADS  Google Scholar 

  • Roussev, I.I., Gombosi, T.I., Sokolov, I.V., Velli, M., Manchester, W. IV, De Zeeuw, D.L., Liewer, P., Tóth, G., Luhmann, J.: 2003, A three-dimensional model of the solar wind incorporating solar magnetogram observations. Astrophys. J. Lett. 595, L57.

    Article  ADS  Google Scholar 

  • Sanderson, T.R., Appourchaux, T., Hoeksema, J.T., Harvey, K.L.: 2003, Observations of the Sun’s magnetic field during the recent solar maximum. J. Geophys. Res. 108, 1035.

    Article  Google Scholar 

  • Smith, E.J.: 2011, Solar cycle evolution of the heliospheric magnetic field: The Ulysses legacy. J. Atmos. Solar-Terr. Phys. 73, 277.

    Article  ADS  Google Scholar 

  • Smith, E.J., Marsden, R.G., Balogh, A., Gloeckler, G., Geiss, J., McComas, D.J., McKibben, R.B., MacDowall, R.J., Lanzerotti, L.J., Krupp, N., Krueger, H., Landgraf, M.: 2003, The Sun and heliosphere at solar maximum. Science 302, 1165.

    Article  ADS  Google Scholar 

  • Stone, J.M., Gardiner, T.A., Teuben, P., Hawley, J.F., Simon, J.B.: 2008, Athena: A new code for astrophysical MHD. Astrophys. J. Suppl. Ser. 178, 137.

    Article  ADS  Google Scholar 

  • Taktakishvili, A., Pulkkinen, A., MacNeice, P., Kuznetsova, M., Hesse, M., Odstrcil, D.: 2011, Modeling of coronal mass ejections that caused particularly large geomagnetic storms using ENLIL heliosphere cone model. Space Weather 90, 6002.

    Article  Google Scholar 

  • Tanaka, T.: 1994, Finite volume tvd scheme on an unstructured grid system for three-dimensional mhd simulation of inhomogeneous systems including strong background potential fields. J. Comput. Phys. 111, 381.

    Article  ADS  MATH  Google Scholar 

  • Tokumaru, M., Kojima, M., Fujiki, K., Hayashi, K.: 2009, Non-dipolar solar wind structure observed in the cycle 23/24 minimum. Geophys. Res. Lett. 36, 9101.

    Article  Google Scholar 

  • Tóth, G., Sokolov, I.V., Gombosi, T.I., Chesney, D.R., Clauer, C.R., De Zeeuw, D.L., Hansen, K.C., Kane, K.J., Manchester, W.B., Oehmke, R.C., Powell, K.G., Ridley, A.J., Roussev, I.I., Stout, Q.F., Volberg, O., Wolf, R.A., Sazykin, S., Chan, A., Yu, B., Kóta, J.: 2005, Space weather modeling framework: A new tool for the space science community. J. Geophys. Res. 110, 12226.

    Article  Google Scholar 

  • Tóth, G., van der Holst, B., Sokolov, I.V., De Zeeuw, D.L., Gombosi, T.I., Fang, F., Manchester, W.B., Meng, X., Najib, D., Powell, K.G., Stout, Q.F., Glocer, A., Ma, Y.-J., Opher, M.: 2012, Adaptive numerical algorithms in space weather modeling. J. Comput. Phys. 231, 870.

    Article  ADS  Google Scholar 

  • Usmanov, A.V.: 1996, A global 3-D MHD solar wind model with Alfvén waves. In: Winterhalter, D., Gosling, J.T., Habbal, S.R., Kurth, W.S., Neugebauer, M. (eds.) AIP Conf. Ser. 382, 141.

    Chapter  Google Scholar 

  • Usmanov, A.V., Goldstein, M.L.: 2006, A three-dimensional MHD solar wind model with pickup protons. J. Geophys. Res. 111, A07101.

    Article  Google Scholar 

  • van der Holst, B., Keppens, R.: 2007, Hybrid block-AMR in Cartesian and curvilinear coordinates: MHD applications. J. Comput. Phys. 226, 925.

    Article  MathSciNet  ADS  MATH  Google Scholar 

  • van der Holst, B., Manchester, W.B., Frazin, R.A., Vásquez, A.M., Tóth, G., Gombosi, T.I.: 2010, A data-driven, two-temperature solar wind model with Alfvén waves. Astrophys. J. 725, 1373.

    Article  ADS  Google Scholar 

  • Vinokur, M.: 1974, Conservation equations of gasdynamics in curvilinear coordinate systems. J. Comput. Phys. 14, 105.

    Article  MathSciNet  ADS  MATH  Google Scholar 

  • Waldmeier, M.: 1981, Cyclic variations of the polar coronal hole. Solar Phys. 70, 251.

    Article  ADS  Google Scholar 

  • Wang, Y.M., Sheeley, N.R. Jr.: 1990, Solar wind speed and coronal flux-tube expansion. Astrophys. J. 355, 726.

    Article  ADS  Google Scholar 

  • Wang, Y.M., Robbrecht, E., Sheeley, N.R. Jr.: 2009, On the weakening of the polar magnetic fields during solar cycle 23. Astrophys. J. 707, 1372.

    Article  ADS  Google Scholar 

  • Wang, Y.M., Sheeley, N.R. Jr., Walters, J.H., Brueckner, G.E., Howard, R.A., Michels, D.J., Lamy, P.L., Schwenn, R., Simnett, G.M.: 1998, Origin of streamer material in the outer corona. Astrophys. J. Lett. 498, L165. doi: 10.1086/311321 .

    Article  ADS  Google Scholar 

  • Wang, A.H., Wu, S.T., Tandberg-Hanssen, E., Hill, F.: 2011, Utilization of multiple measurements for global three-dimensional magnetohydrodynamic simulations. Astrophys. J. 732, 19.

    Article  ADS  Google Scholar 

  • Watermann, J., Wintoft, P., Sanahuja, B., Saiz, E., Poedts, S., Palmroth, M., Milillo, A., Metallinou, F.-A., Jacobs, C., Ganushkina, N.Y., Daglis, I.A., Cid, C., Cerrato, Y., Balasis, G., Aylward, A.D., Aran, A.: 2009, Models of solar wind structures and their interaction with the Earth’s space environment. Space Sci. Rev. 147, 233.

    Article  ADS  Google Scholar 

  • Wei, F., Feng, X., Cai, H., Zhou, Q.: 2003, Global distribution of coronal mass outputs and its relation to solar magnetic field structures. J. Geophys. Res. 108, 1238.

    Article  Google Scholar 

  • Wu, S.T., Wang, A.H., Liu, Y., Hoeksema, J.T.: 2006, Data-driven magnetohydrodynamic model for active region evolution. Astrophys. J. 652, 800.

    Article  ADS  Google Scholar 

  • Yang, L., Feng, X., Xiang, C., Zhang, S., Wu, S.T.: 2011, Simulation of the unusual solar minimum with 3D SIP–CESE MHD model by comparison with multi-satellite observations. Solar Phys. 271, 91.

    Article  ADS  Google Scholar 

  • Yeates, A.R., Mackay, D.H., van Ballegooijen, A.A., Constable, J.A.: 2010a, A nonpotential model for the Sun’s open magnetic flux. J. Geophys. Res. 115, A09112.

    Article  ADS  Google Scholar 

  • Yeates, A.R., Attrill, G.D.R., Nandy, D., Mackay, D.H., Martens, P.C.H., van Ballegooijen, A.A.: 2010b, Comparison of a global magnetic evolution model with observations of coronal mass ejections. Astrophys. J. 709, 1238.

    Article  ADS  Google Scholar 

  • Ziegler, U.: 2008, The NIRVANA code: Parallel computational MHD with adaptive mesh refinement. Comput. Phys. Commun. 179, 227.

    Article  ADS  MATH  Google Scholar 

Download references

Acknowledgements

The work is jointly supported by the National Natural Science Foundation of China (41031066, 40921063, 40874091, 40890162, 40904050, 40874077, 41074121, and 41074122), 973 program 2012CB825601 and the Specialized Research Fund for State Key Laboratories. S.T. Wu is supported by AFOSR (grant FA9550-07-1-0468), AURA Sub-Award C10569A of NSO’s Cooperative Agreement AST 0132798, and NSF (grant ATM-0754378). The numerical calculation has been completed on our SIGMA Cluster computing system. The PARAMESH software used in this work was developed at the NASA Goddard Space Flight Center and Drexel University under NASA’s HPCC and ESTO/CT projects and under grant NNG04GP79G from the NASA/AISR project. Wilcox Solar Observatory data used in this study were obtained via http://wso.stanford.edu . The Wilcox Solar Observatory is currently supported by NASA. SOHO is a project of international cooperation between ESA and NASA. The OMNI data is obtained from the GSFC/SPDF OMNIWeb interface http://omniweb.gsfc.nasa.gov . Special thanks go to our reviewer for the invaluable comments on the improvement of the article.

Author information

Authors and Affiliations

  1. SIGMA Weather Group, State Key Laboratory for Space Weather, Center for Space Science and Applied Research, Chinese Academy of Sciences, Beijing, 100190, China

    Xueshang Feng, Liping Yang, Changqing Xiang, Caowei Jiang, Xiaopeng Ma, DingKun Zhong & Yufen Zhou

  2. College of Earth Sciences, Graduate University of Chinese Academy of Sciences, Beijing, 100049, China

    Liping Yang, Caowei Jiang & Xiaopeng Ma

  3. Center for Space Plasma and Aeronomic Research, The University of Alabama in Huntsville, Huntsville, AL, 35899, USA

    S. T. Wu

Authors
  1. Xueshang Feng
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Liping Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Changqing Xiang
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Caowei Jiang
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Xiaopeng Ma
    View author publications

    You can also search for this author in PubMed Google Scholar

  6. S. T. Wu
    View author publications

    You can also search for this author in PubMed Google Scholar

  7. DingKun Zhong
    View author publications

    You can also search for this author in PubMed Google Scholar

  8. Yufen Zhou
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Xueshang Feng.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Feng, X., Yang, L., Xiang, C. et al. Validation of the 3D AMR SIP–CESE Solar Wind Model for Four Carrington Rotations. Sol Phys 279, 207–229 (2012). https://doi.org/10.1007/s11207-012-9969-9

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11207-012-9969-9

Keywords

Search

Navigation