Abstract
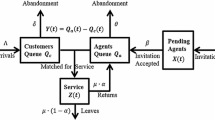
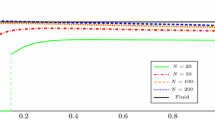
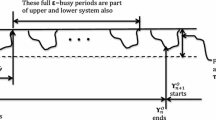
Motivated by applications in telephone call centers, we consider a service system model with m customer classes and r server pools. The model is one with doubly stochastic arrivals, which means that the m-vector λ of instantaneous arrival rates is allowed to vary both temporally and stochastically. Two levels of dynamic control are considered: customers may be either blocked or accepted at the time of their arrival, and then accepted customers of each class must be routed, either immediately upon acceptance or after some period of waiting, to a server pool that is qualified to handle that class. Customers who are made to wait before commencement of their service are liable to defect. The objective is to minimize the expected sum of blocking costs, waiting costs and defection costs over a fixed and finite planning horizon. We consider an asymptotic parameter regime in which (i) the arrival rates, service rates and defection rates are uniformly accelerated by a large factor κ, then (ii) arrival rates are increased by an additional factor g(κ), and the number of servers in each pool is increased by g(κ) as well. This produces a separation of time scales, justifying a pointwise stationary stochastic fluid approximation for our original system model. In the stochastic fluid approximation, optimal admission control and routing decisions are determined by a simple linear program that uses the current arrival rate vector λ as data. We explain how to implement the fluid model's optimal control policy in our original service system context, and prove that the proposed implementation is asymptotically optimal in the first-order sense.
Similar content being viewed by others
References
A. Bassamboo, J.M. Harrison and A. Zeevi, Design and control of a large call center: Asymptotic analysis of an LP-based method, Operations Research (To appear 2005).
P. Bremaud, Point Processes and Queues: Martingale Dynamics., (Springer Verlag, New York, 1981).
N. Gans, G. Koole and A. Mandelbaum, Telephone call centers: Tutorial, review, and research prospects, {Manufacturing & Service Operations Management} 5 (2003) 79–141.
L. Green and P. Kolesar, The pointwise stationary approximation for queues with nonstationary arrivals, {Management Science} {37} (1991) 84–97.
S. Halfin and W. Whitt, Heavy-traffic limits for queues with many exponential servers, {Operations Research} {29} (1981) 567–588.
J.M. Harrison and M. Lopez, Heavy traffic resource pooling in parallel-server systems, {Queueing Systems} {33} (1999) 339–368.
J.M. Harrison and A. Zeevi, Dynamic scheduling of a multi-class queue in the Halfin-Whitt heavy traffic regime, {Operations Research} {52} (2004) 243–257.
J.M. Harrison and A. Zeevi, A method for staffing large call centers based on stochastic fluid models, {Manufacturing & Service Operations Management} 7 (2005) 20–36.
J. Komlós, P. Major and G. Tusnady, An approximation of partial sums of independent random variables and the sample distribution I, {Z. Wahr. und Verw. Gebiete} {32} (1975) 111–131.
T. Kurtz, Strong approximation theorems for density dependent Markov chains, {Stochastic Processes and Their Applications} {6} (1978) 223–240.
C. Maglaras and J. Van Mieghem, Admission and sequencing control under delay constraints with applications to GPS and GLQ. To appear in the European Journal of Operations Research (2004).
A. Mandelbaum, W. Massey and M. Reiman, Strong approximations for Markovian service networks, {Queueing Systems, Theory and Applications (QUESTA)} 30 (1998) 149–201 .
W.A. Massey and W. Whitt, Uniform acceleration expansions for Markov chains with time-varying rates, {Annals of Applied Probabability} 8(4) (1998) 1130–1155.
E. Plambeck, S. Kumar and J.M. Harrison, A multiclass queue in heavy traffic with throughput time constraints: Asymptotically optimal dynamic controls, {Queueing Systems} {39} (2001) 23–54.
W. Whitt, Comparing counting processes and queues, {Advances in Applied Probability} {13} (1981) 207–220.
W. Whitt, The pointwise stationary approximation for M. t./M. t./s. queues is asymptotically correct as the rates increase, {Management Science} {37} (1991) 307–314.
W. Whitt, Stochastic-Process Limits., (Springer-Verlag, New York, 2002).
W. Whitt, Fluid models for many-server queues with abandonments, Working paper (2004). To appear in Operations Research.
Author information
Authors and Affiliations
Corresponding author
Additional information
AMS subject classification: 60K30, 90B15, 90B36
Rights and permissions
About this article
Cite this article
Bassamboo, A., Harrison, J.M. & Zeevi, A. Dynamic Routing and Admission Control in High-Volume Service Systems: Asymptotic Analysis via Multi-Scale Fluid Limits. Queueing Syst 51, 249–285 (2005). https://doi.org/10.1007/s11134-005-2897-8
Received:
Revised:
Issue Date:
DOI: https://doi.org/10.1007/s11134-005-2897-8