Complete analysis to minimum-error discrimination of four mixed qubit states with arbitrary prior probabilities | Quantum Information Processing Skip to main content
Springer Nature Link
Log in

Complete analysis to minimum-error discrimination of four mixed qubit states with arbitrary prior probabilities

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

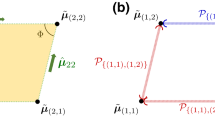
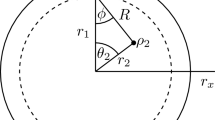
Abstract

In this work, we provide a complete analysis to minimum-error discrimination of mixed four qubit states with arbitrary prior probabilities. For the complete analysis, the most important work to do is to find the necessary and sufficient conditions for the existence of null measurement operator. From the geometric structure of qubit states, we obtain the analytic condition for deciding the existence of a null operator in minimum-error measurement for four mixed qubit states, which also gives the necessary and sufficient conditions for every optimal POVM to have nonzero elements. Using the condition, we completely analyze minimum-error discrimination of four mixed qubit states with arbitrary prior probabilities.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

Data availability

All data generated or used during the study appear in the submitted article.

References

  1. Chefles, A.: Quantum state discrimination. Contemp. Phys. 41, 401 (2000)

    Article  ADS  MATH  Google Scholar 

  2. Barnett, S.M., Croke, S.: Quantum state discrimination. Adv. Opt. Photon. 1, 238 (2009)

    Article  Google Scholar 

  3. Bergou, J.A.: Discrimination of quantum states. J. Mod. Opt. 57, 160 (2010)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  4. Bae, J., Kwek, L.C.: Quantum state discrimination and its applications. J. Phys. A Math. Theor. 48, 083001 (2015)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  5. Helstrom, C.W.: Quantum Detection and Estimation Theory. Academic Press, New York (1976)

    MATH  Google Scholar 

  6. Holevo, A.S.: Probabilistic and Statistical Aspects of Quantum Theory. North-Holland, Amsterdam (1979)

    Google Scholar 

  7. Yuen, H.P., Kennedy, R.S., Lax, M.: Optimum testing of multiple hypotheses in quantum detection theory. IEEE Trans. Inf. Theory 21, 125 (1975)

    Article  MathSciNet  MATH  Google Scholar 

  8. Barnett, S.M., Croke, S.: On the conditions for discrimination between quantum states with minimum error. J. Phys. A Math. Theor. 42, 062001 (2009)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  9. Herzog, U.: Minimum-error discrimination between a pure and a mixed two-qubit state. J. Opt. B Quantum Semiclass. Opt. 6, S24 (2004)

    Article  ADS  Google Scholar 

  10. Ban, M., Kurokawa, K., Momose, R., Hirota, O.: Optimum measurements for discrimination among symmetric quantum states and parameter estimation. Int. J. Theor. Phys. 36, 1269 (1997)

    Article  MathSciNet  MATH  Google Scholar 

  11. Eldar, Y.C., Forney, G.D.: On quantum detection and the square-root measurement. IEEE Trans. Inf. Theory 47, 858 (2001)

    Article  MathSciNet  MATH  Google Scholar 

  12. Chou, C.L., Hsu, L.Y.: Minimum-error discrimination between symmetric mixed quantum states. Phys. Rev. A 68, 042305 (2003)

    Article  ADS  Google Scholar 

  13. Mochon, C.: Family of generalized “pretty good’’ measurements and the minimal-error pure-state discrimination problems for which they are optimal. Phys. Rev. A 73, 032328 (2006)

    Article  ADS  Google Scholar 

  14. Samsonov, B.F.: Minimum error discrimination problem for pure qubit states. Phys. Rev. A 80, 052305 (2009)

    Article  ADS  MathSciNet  Google Scholar 

  15. Bae, J., Hwang, W.Y.: Minimum-error discrimination of qubit states: methods, solutions, and properties. Phys. Rev. A 87, 012334 (2013)

    Article  ADS  Google Scholar 

  16. Bae, J.: Structure of minimum-error quantum state discrimination. New. J. Phys. 15, 073037 (2013)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  17. Ha, D., Kwon, Y.: Complete analysis for three-qubit mixed-state discrimination. Phys. Rev. A 87, 062302 (2013)

    Article  ADS  Google Scholar 

  18. Ha, D., Kwon, Y.: Discriminating \(N\)-qudit states using geometric structure. Phys. Rev. A 90, 022330 (2014)

    Article  ADS  Google Scholar 

  19. Ha, D., Kwon, Y.: Quantum nonlocality without entanglement: explicit dependence on prior probabilities of nonorthogonal mirror-symmetric states. NPJ Quantum Inf. 7(1), 1–6 (2021)

    Google Scholar 

  20. Deconinck, M.E., Terhal, B.M.: Qubit state discrimination. Phys. Rev. A 81, 062304 (2010)

    Article  ADS  Google Scholar 

  21. Eldar, Y.C., Megretski, A., Verghese, G.C.: Designing optimal quantum detectors via semidefinite programming. IEEE Trans. Inf. Theory 49, 1007 (2003)

    Article  MathSciNet  MATH  Google Scholar 

  22. Boyd, S., Vandenberghe, L.: Convex Optimization. Cambridge University Press, UK (2004)

    Book  MATH  Google Scholar 

  23. Nakahira, K., Kato, K., Usuda, T.S.: Generalized quantum state discrimination problems. Phys. Rev. A 91, 052304 (2015)

    Article  ADS  MATH  Google Scholar 

  24. Berkovitz, L.D.: Convexity and Optimization in \({\mathbb{R} }^{n}\). Wiley, New York (2002)

    Google Scholar 

  25. Davies, E.B.: Information and quantum measurement. IEEE Trans. Inf. Theory 24, 596 (1978)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  26. Hunter, K.: Results in optimal discrimination. AIP Conf. Proc. 734, 83 (2004)

    Article  ADS  MathSciNet  Google Scholar 

Download references

Acknowledgements

This work is supported by the Basic Science Research Program through the National Research Foundation of Korea (NRF) funded by the Ministry of Education, Science and Technology (NRF2018R1D1A1B07049420 and NRF2022R1F1A1064459) and Institute of Information and Communications Technology Planning and Evaluation (IITP) grant funded by the Korean government (MSIT) (No. 2020001343, Artificial Intelligence Convergence Research Center (Hanyang University ERICA)

Author information

Authors and Affiliations

  1. Department of Applied Mathematics and Institute of Natural Sciences, Kyung Hee University, Yongin, 17104, Republic of Korea

    Donghoon Ha

  2. Department of Applied Physics, Hanyang University, Ansan, 15588, Republic of Korea

    Younghun Kwon

Authors
  1. Donghoon Ha
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Younghun Kwon
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Younghun Kwon.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Springer Nature or its licensor (e.g. a society or other partner) holds exclusive rights to this article under a publishing agreement with the author(s) or other rightsholder(s); author self-archiving of the accepted manuscript version of this article is solely governed by the terms of such publishing agreement and applicable law.

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Ha, D., Kwon, Y. Complete analysis to minimum-error discrimination of four mixed qubit states with arbitrary prior probabilities. Quantum Inf Process 22, 67 (2023). https://doi.org/10.1007/s11128-022-03814-0

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11128-022-03814-0

Keywords

Search

Navigation