Quantitative wave–particle duality relations from the density matrix properties | Quantum Information Processing Skip to main content

Advertisement

Springer Nature Link
Log in

Quantitative wave–particle duality relations from the density matrix properties

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

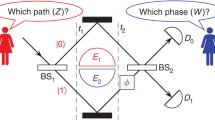
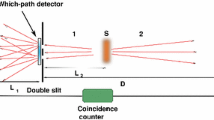
Abstract

We derive upper bounds for Hilbert–Schmidt’s quantum coherence of general states of a d-level quantum system, a qudit, in terms of its incoherent uncertainty, with the latter quantified using the linear and von Neumann’s entropies of the corresponding closest incoherent state. Similar bounds are obtained for Wigner–Yanase’s coherence. The reported inequalities are also given as coherence–populations trade-off relations. As an application example of these inequalities, we derive quantitative wave–particle duality relations for multi-slit interferometry. Our framework leads to the identification of predictability measures complementary to Hilbert–Schmidt’s, Wigner–Yanase’s, and \(l_{1}\)-norm quantum coherences. The quantifiers reported here for the wave and particle aspects of a quanton follow directly from the defining properties of the quantum density matrix (i.e., semi-positivity and unit trace), contrasting thus with most related results from the literature.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Fallani, L., Kastberg, A.: Cold atoms: a field enabled by light. EPL 110, 53001 (2015)

    ADS  Google Scholar 

  2. Simmons, M.: A new horizon for quantum information. npj Quantum Inf. 1, 15013 (2015)

    ADS  Google Scholar 

  3. Pirandola, S., Braunstein, S.L.: Physics: unite to build a quantum Internet. Nat. News 532, 169 (2016)

    Google Scholar 

  4. Schleier-Smith, M.: Editorial: hybridizing quantum physics and engineering. Phys. Rev. Lett. 117, 100001 (2016)

    ADS  Google Scholar 

  5. Institute of Physics Publications, The age of the qubit, (2011)

  6. Fitzsimons, J., Rieffel, F.E.G., Scarani, V.: The quantum Frontier. arXiv:1206.0785

  7. Adesso, G., Franco, R.L., Parigi, V.: Foundations of quantum mechanics and their impact on contemporary society. Philos. Trans. R. Soc. A 376, 20180112 (2018)

    ADS  Google Scholar 

  8. Stuhler, J.: Quantum optics route to market. Nat. Phys. 11, 293 (2015)

    Google Scholar 

  9. Preskill, J.: Quantum information and physics: some future directions. J. Mod. Opt. 47, 127 (2000)

    ADS  MathSciNet  Google Scholar 

  10. Cowen, R.: The quantum source of space-time. Nature 527, 290 (2015)

    ADS  Google Scholar 

  11. Biamonte, J., Wittek, P., Pancotti, N., Rebentrost, P., Wiebe, N., Lloyd, S.: Quantum machine learning. Nature 549, 195 (2017)

    ADS  Google Scholar 

  12. Popescu, S.: Bell’s inequalities versus teleportation: What is nonlocality? Phys. Rev. Lett. 72, 797 (1994)

    ADS  MathSciNet  MATH  Google Scholar 

  13. Cavalcanti, P.S.D., Šupić, I.: All entangled states can demonstrate nonclassical teleportation. Phys. Rev. Lett. 119, 110501 (2017)

    ADS  Google Scholar 

  14. Brunner, N., Cavalcanti, D., Pironio, S., Scarani, V., Wehner, S.: Bell nonlocality. Rev. Mod. Phys. 86, 419 (2014)

    ADS  Google Scholar 

  15. Branciard, C., Cavalcanti, E.G., Walborn, S.P., Scarani, V., Wiseman, H.M.: One-sided device-independent quantum key distribution: security, feasibility, and the connection with steering. Phys. Rev. A 85, 010301(R) (2012)

    ADS  Google Scholar 

  16. Schnabel, R.: Squeezed states of light and their applications in laser interferometers. Phys. Rep. 684, 1 (2017)

    ADS  MathSciNet  MATH  Google Scholar 

  17. Girolami, D., Souza, A.M., Giovannetti, V., Tufarelli, T., Filgueiras, J.G., Sarthour, R.S., Soares-Pinto, D.O., Oliveira, I.S., Adesso, G.: Quantum discord determines the interferometric power of quantum states. Phys. Rev. Lett. 112, 210401 (2014)

    ADS  Google Scholar 

  18. Howard, M., Wallman, J.J., Veitch, V., Emerson, J.: Contextuality supplies the magic for quantum computation. Nature 510, 351 (2014)

    ADS  Google Scholar 

  19. Shi, H.-L., Liu, S.-Y., Wang, X.-H., Yang, W.-L., Yang, Z.-Y., Fan, H.: Coherence depletion in the Grover quantum search algorithm. Phys. Rev. A 95, 032307 (2017)

    ADS  MathSciNet  Google Scholar 

  20. Theurer, T., Killoran, N., Egloff, D., Plenio, M.B.: Resource theory of superposition. Phys. Rev. Lett. 119, 230401 (2017)

    ADS  Google Scholar 

  21. Åberg, J.: Catalytic coherence. Phys. Rev. Lett. 113, 150402 (2014)

    ADS  Google Scholar 

  22. von Prillwitz, K., Rudnicki, L., Mintert, F.: Contrast in multipath interference and quantum coherence. Phys. Rev. A 92, 052114 (2015)

    ADS  Google Scholar 

  23. Streltsov, A., Adesso, G., Plenio, M.B.: Quantum coherence as a resource. Rev. Mod. Phys. 89, 041003 (2017)

    ADS  MathSciNet  Google Scholar 

  24. Chitambar, E., Ma, X., Streltsov, A.: Preface: quantum coherence. J. Phys. A Math. Theor. 51, 410301 (2018)

    MathSciNet  MATH  Google Scholar 

  25. Messiah, A.: Quantum Mechanics. Dover, New York (2014)

    MATH  Google Scholar 

  26. Fano, U.: Description of states in quantum mechanics by density matrix and operator techniques. Rev. Mod. Phys. 29, 74 (1957)

    ADS  MathSciNet  MATH  Google Scholar 

  27. Wilde, M.: Quantum Information Theory. Cambridge University Press, New York (2013)

    MATH  Google Scholar 

  28. Maziero, J.: Hilbert–Schmidt quantum coherence in multi-qudit systems. Quantum Inf. Process. 16, 274 (2017)

    ADS  MathSciNet  MATH  Google Scholar 

  29. Baumgratz, T., Cramer, M., Plenio, M.B.: Quantifying coherence. Phys. Rev. Lett. 113, 140401 (2014)

    ADS  Google Scholar 

  30. Yu, C.-S.: Quantum coherence via skew information and its polygamy. Phys. Rev. A 95, 042337 (2017)

    ADS  Google Scholar 

  31. Puchała, Z., Rudnicki, Ł., Chabuda, K., Paraniak, M., Życzkowski, K.: Certainty relations, mutual entanglement and non-displacable manifolds. Phys. Rev. A 92, 032109 (2015)

    ADS  Google Scholar 

  32. Korzekwa, K., Lostaglio, M., Jennings, D., Rudolph, T.: Quantum and classical entropic uncertainty relations. Phys. Rev. A 89, 042122 (2014)

    ADS  Google Scholar 

  33. Bagan, E., Bergou, J.A., Cottrell, S.S., Hillery, M.: Relations between coherence and path information. Phys. Rev. Lett. 116, 160406 (2016)

    ADS  Google Scholar 

  34. Singh, U., Bera, M.N., Dhar, H.S., Pati, A.K.: Maximally coherent mixed states: complementarity between maximal coherence and mixedness. Phys. Rev. A 91, 052115 (2015)

    ADS  Google Scholar 

  35. Cheng, S., Hall, M.J.W.: Complementarity relations for quantum coherence. Phys. Rev. A 92, 042101 (2015)

    ADS  Google Scholar 

  36. Liu, F., Li, F., Chen, J., Xing, W.: Uncertainty-like relations of the relative entropy of coherence. Quantum Inf. Process. 15, 3459 (2016)

    ADS  MathSciNet  MATH  Google Scholar 

  37. Pessoa Jr., O.: Conceitos de Física Quântica. Editora Livraria da Física, São Paulo (2006)

    Google Scholar 

  38. Qureshi, T.: Coherence, interference and visibility. Quanta 8, 24 (2019)

    MathSciNet  Google Scholar 

  39. Dürr, S.: Quantitative wave-particle duality in multibeam interferometers. Phys. Rev. A 64, 042113 (2001)

    ADS  Google Scholar 

  40. Englert, B.-G., Kaszlikowski, D., Kwek, L.C., Chee, W.H.: Wave-particle duality in multi-path interferometers: general concepts and three-path interferometers. Int. J. Quantum Inf. 6, 129 (2008)

    MATH  Google Scholar 

  41. Mishra, S., Venugopalan, A., Qureshi, T.: Decoherence and visibility enhancement in multi-path interference. Phys. Rev. A 100, 042122 (2019)

    ADS  Google Scholar 

  42. Bertlmann, R.A., Krammer, P.: Bloch vectors for qudits. J. Phys. A Math. Theor. 41, 235303 (2008)

    ADS  MathSciNet  MATH  Google Scholar 

  43. Kuttler, K.: Elementary Linear Algebra. Saylor Foundation, Washington, DC (2012)

    Google Scholar 

  44. Avendaño, M.: Descartes’ rule of signs is exact!. J. Algebra 324, 2884 (2010)

    MathSciNet  MATH  Google Scholar 

  45. Nielsen, M.A., Chuang, I.L.: Quantum Computation and Quantum Information. Cambridge University Press, Cambridge (2000)

    MATH  Google Scholar 

  46. Ledoux, M.: The Concentration of Measure Phenomenon. American Mathematical Society, Providence (2001)

    MATH  Google Scholar 

  47. Maziero, J.: Random sampling of quantum states: a survey of methods. Braz. J. Phys. 45, 575 (2015)

    ADS  Google Scholar 

  48. Maziero, J.: Fortran code for generating random probability vectors, unitaries, and quantum states. Front. ICT 3, 4 (2016)

    Google Scholar 

  49. Horn, R.A., Johnson, C.R.: Matrix Analysis. Cambridge University Press, Cambridge (2013)

    MATH  Google Scholar 

  50. Wehrl, A.: General properties of entropy. Rev. Mod. Phys. 50, 221 (1978)

    ADS  MathSciNet  MATH  Google Scholar 

  51. Hiroshima, T., Ishizaka, S.: Local and nonlocal properties of Werner states. Phys. Rev. A 62, 044302 (2000)

    ADS  MathSciNet  Google Scholar 

  52. Wigner, E.P., Yanase, M.M.: Information contents of distributions. Proc. Nat. Acad. Sci. USA 49, 910 (1963)

    ADS  MathSciNet  MATH  Google Scholar 

  53. Hillery, M.: Coherence as a resource in decision problems: the Deutsch-Jozsa algorithm and a variation. Phys. Rev. A 93, 012111 (2016)

    ADS  Google Scholar 

  54. Kammerlander, P., Anders, J.: Coherence and measurement in quantum thermodynamics. Sci. Rep. 6, 22174 (2016)

    ADS  Google Scholar 

  55. Streltsov, A., Chitambar, E., Rana, S., Bera, M.N., Winter, A., Lewenstein, M.: Entanglement and coherence in quantum state merging. Phys. Rev. Lett. 116, 240405 (2016)

    ADS  Google Scholar 

  56. Yu, C., Yang, S., Guo, B.: Total quantum coherence and its applications. Quantum Inf. Process. 15, 3773 (2016)

    ADS  MathSciNet  MATH  Google Scholar 

  57. Yuan, X., Liu, K., Xu, Y., Wang, W., Ma, Y., Zhang, F., Yan, Z., Vijay, R., Sun, L., Ma, X.: Experimental quantum randomness processing. Phys. Rev. Lett. 117, 010502 (2016)

    ADS  Google Scholar 

  58. Giorda, P., Allegra, M.: Coherence in quantum estimation. J. Phys. A Math. Theor. 51, 025302 (2018)

    ADS  MathSciNet  MATH  Google Scholar 

  59. Zhang, F.-L., Wang, T.: Intrinsic coherence in assisted sub-state discrimination. EPL 117, 10013 (2017)

    ADS  Google Scholar 

  60. Pozzobom, M.B., Maziero, J.: Environment-induced quantum coherence spreading of a qubit. Ann. Phys. 377, 243 (2017)

    ADS  MATH  Google Scholar 

  61. Buruaga, D.N.S., Sabín, C.: Quantum coherence in the dynamical Casimir effect. Phys. Rev. A 95, 022307 (2017)

    ADS  Google Scholar 

  62. Li, L., Zou, J., Li, H., Li, J.-G., Wang, Y.-M., Shao, B.: Controlling energy flux into a spatially correlated environment via quantum coherence. Eur. Phys. J. D 71, 62 (2017)

    ADS  Google Scholar 

  63. Brandner, K., Bauer, M., Seifert, U.: Universal coherence-induced power losses of quantum heat engines in linear response. Phys. Rev. Lett. 119, 170602 (2017)

    ADS  Google Scholar 

  64. Scholes, G.D., Fleming, G.R., Chen, L.X., Aspuru-Guzik, A., Buchleitner, A., Coker, D.F., Engel, G.S., van Grondelle, R., Ishizaki, A., Jonas, D.M., Lundeen, J.S., McCusker, J.K., Mukamel, S., Ogilvie, J.P., Olaya-Castro, A., Ratner, M.A., Spano, F.C., Whaley, K.B., Zhu, X.: Using coherence to enhance function in chemical and biophysical systems. Nature 543, 647 (2017)

    ADS  Google Scholar 

  65. Bengtson, C., Sjöqvist, E.: The role of quantum coherence in dimer and trimer excitation energy transfer. New J. Phys. 19, 113015 (2017)

    ADS  Google Scholar 

  66. Pinto, D.F., Maziero, J.: Entanglement production by the magnetic dipolar interaction dynamics. Quantum Inf. Proc. 17, 253 (2018)

    ADS  MATH  Google Scholar 

  67. Southwell, K.: Quantum coherence. Nature 453, 1003 (2008)

    ADS  Google Scholar 

  68. Li, F., Bao, W.-S., Zhang, S., Huang, H., Li, T., Wang, X., Fu, X.: Role of coherence in adiabatic search algorithms. Phys. Lett. A 382, 2709 (2018)

    ADS  MathSciNet  MATH  Google Scholar 

  69. Byrd, M.S., Khaneja, N.: Characterization of the positivity of the density matrix in terms of the coherence vector representation. Phys. Rev. A 68, 062322 (2003)

    ADS  MathSciNet  Google Scholar 

  70. Kimura, G.: The Bloch vector for N-level systems. Phys. Lett. A 314, 339 (2003)

    ADS  MathSciNet  MATH  Google Scholar 

  71. Englert, B.-G.: Fringe visibility and which-way information: an inequality. Phys. Rev. Lett. 77, 2154 (1996)

    ADS  Google Scholar 

  72. Brandão, F.G.S.L., Gour, G.: The general structure of quantum resource theories. Phys. Rev. Lett. 115, 070503 (2015)

    ADS  MathSciNet  Google Scholar 

  73. Chitambar, E., Gour, G.: Quantum resource theories. Rev. Mod. Phys. 91, 025001 (2019)

    ADS  MathSciNet  Google Scholar 

  74. Huber, M., Perarnau-Llobet, M., Hovhannisyan, K.V., Skrzypczyk, P., Klöckl, C., Brunner, N., Acín, A.: Thermodynamic cost of creating correlations. New J. Phys. 17, 065008 (2015)

    ADS  MATH  Google Scholar 

  75. Goold, J., Huber, M., Riera, A., del Rio, L., Skrzypczyk, P.: The role of quantum information in thermodynamics—a topical review. J. Phys. A Math. Theor. 49, 143001 (2016)

    ADS  MathSciNet  MATH  Google Scholar 

  76. Anders, J., Esposito, M.: Focus on quantum thermodynamics. New J. Phys. 19, 010201 (2017)

    ADS  Google Scholar 

  77. Lostaglio, M.: An introductory review of the resource theory approach to thermodynamics. Rep. Prog. Phys. 82, 114001 (2019)

    ADS  MathSciNet  Google Scholar 

Download references

Acknowledgements

This work was supported by the Coordenação de Aperfeiçoamento de Pessoal de Nível Superior (CAPES), process 88882.427924/2019-01, by the Fundação de Amparo à Pesquisa do Estado do Rio Grande do Sul (FAPERGS), and by the Instituto Nacional de Ciência e Tecnologia de Informação Quântica (INCT-IQ), process 465469/2014-0.

Author information

Authors and Affiliations

  1. Departamento de Física, Centro de Ciências Naturais e Exatas, Universidade Federal de Santa Maria, Avenida Roraima 1000, Santa Maria, RS, 97105-900, Brazil

    Marcos L. W. Basso, Diego S. S. Chrysosthemos & Jonas Maziero

Authors
  1. Marcos L. W. Basso
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Diego S. S. Chrysosthemos
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Jonas Maziero
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Jonas Maziero.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Basso, M.L.W., Chrysosthemos, D.S.S. & Maziero, J. Quantitative wave–particle duality relations from the density matrix properties. Quantum Inf Process 19, 254 (2020). https://doi.org/10.1007/s11128-020-02753-y

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11128-020-02753-y

Keywords

Search

Navigation