Enhancing non-Markovianity by quantum feedback control | Quantum Information Processing Skip to main content
Springer Nature Link
Log in

Enhancing non-Markovianity by quantum feedback control

  • Published:
Quantum Information Processing Aims and scope Submit manuscript

Abstract

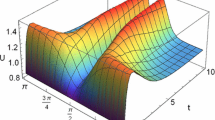
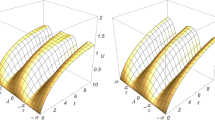
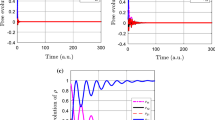
Manipulation of non-Markovian dynamics of open quantum systems has become an important topic in the field of quantum information since quantum non-Markovianity has been proved to be a potential quantum resource. Here, focusing on a simple yet well-known model of a two-level system coupled to bosonic fields, we study how to control the non-Markovian dynamics of an open quantum system using quantum-jump-based feedback control. Numerical simulations show that the quantum-jump-based feedback control can be used to enhance the measure of non-Markovianity. We find that the non-Markovianity can further be optimized by choosing appropriate feedback amplitude. These results may trigger potential applications in exploring non-Markovian effect for future quantum technology and quantum memory.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Breuer, H.P., Petruccione, F.: The Theory of Open Quantum Systems. Oxford University Press, Oxford (2001)

    MATH  Google Scholar 

  2. Zhang, D.J., Huang, H.L., Tong, D.M.: Non-Markovian quantum dissipative processes with the same positive features as Markovian dissipative processes. Phys. Rev. A 93(1–5), 012117 (2016)

    Article  ADS  Google Scholar 

  3. Zhang, D.J., Yu, X.-D., Huang, H.-L., Tong, D.M.: General approach to find steady-state manifolds in Markovian and non-Markovian systems. Phys. Rev. A 94(1–8), 052132 (2016)

    Article  ADS  Google Scholar 

  4. Chin, A.W., Huelga, S.F., Plenio, M.B.: Quantum metrology in non-Markovian environments. Phys. Rev. Lett. 109(1–5), 233601 (2012)

    Article  ADS  Google Scholar 

  5. Bai, K., Peng, Z., Luo, H.-G., An, J.-H.: Retrieving ideal precision in noisy quantum optical metrology. Phys. Rev. Lett. 123(1–6), 040402 (2019)

    Article  ADS  Google Scholar 

  6. Vasile, R., Olivares, S., Paris, M.G.A., Maniscalco, S.: Continuous-variable quantum key distribution in non-Markovian channels. Phys. Rev. A 83(1–6), 042321 (2011)

    Article  ADS  Google Scholar 

  7. Davies, E.B.: Quantum Theory of Open Systems. Academic Press, Cambridge (1976)

    MATH  Google Scholar 

  8. Xu, K., Han, W., Zhang, Y.-J., Xia, Y.-J., Fan, H.: Hierarchical-environment-assisted non-Markovian speedup dynamics control. Phys. Rev. A 98(1–7), 022114 (2018)

    Article  ADS  MATH  Google Scholar 

  9. Aolita, L., De Melo, F., Davidovich, L.: Open-system dynamics of entanglement: a key issues review. Rep. Prog. Phys 78(1–79), 042001 (2015)

    Article  ADS  Google Scholar 

  10. Franco, R.L., Bellomo, B., Maniscalco, S., Compagno, G.: Dynamics of quantum correlations in two-qubit systems within non-Markovian environments. Int. J. Mod. Phys. B 27(1–20), 1345053 (2013)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  11. Xu, J.-S., Sun, K., Li, C.-F., Xu, X.-Y., Guo, G.-C., Andersson, E., Franco, R.L., Compagno, G.: Experimental recovery of quantum correlations in absence of system-environment back-action. Nat. Commun. 4(1–7), 2851 (2013)

    Article  ADS  Google Scholar 

  12. Orieux, A., D’Arrigo, A., Ferranti, G., Franco, R.L., Benenti, G., Paladino, E., Falci, G., Sciarrino, F., Mataloni, P.: Experimental on-demand recovery of quantum entanglement by local operations within non-Markovian dynamics. Sci. Rep. 5(1–8), 8575 (2015)

    Article  ADS  Google Scholar 

  13. D’Arrigo, A., Franco, R.L., Benenti, G., Paladino, E., Falci, G.: Recovering entanglement by local operations. Ann. Phys. 350, 211–224 (2014)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  14. Man, Z.-X., Xia, Y.-J., Franco, R.L.: Cavity-based architecture to preserve quantum coherence and entanglement. Sci. Rep. 5(1–13), 13843 (2015)

    Article  ADS  Google Scholar 

  15. Mortezapour, A., Naeimi, G., Franco, R.L.: Coherence and entanglement dynamics of vibrating qubits. Optics. Commun. 424, 26–31 (2018)

    Article  ADS  Google Scholar 

  16. Mirkin, N., Poggi, P., Wisniacki, D.: Entangling protocols due to non-Markovian dynamics. Phys. Rev. A 99(1–5), 020301 (2019)

    Article  ADS  Google Scholar 

  17. Mortezapour, A., Borji, M.A., Franco, R.L.: Protecting entanglement by adjusting the velocities of moving qubits inside non-Markovian environments. Laser. Phys. Lett. 14(1–9), 055201 (2017)

    Article  ADS  Google Scholar 

  18. Franco, R.L., D’Arrigo, A., Falci, G., Compagno, G., Paladino, E.: Preserving entanglement and nonlocality in solid-state qubits by dynamical decoupling. Phys. Rev. B 90(1–11), 054304 (2014)

    Article  ADS  Google Scholar 

  19. Dijkstra, A.G., Tanimura, Y.: Non-Markovian entanglement dynamics in the presence of system-bath coherence. Phys. Rev. Lett. 104(1–4), 250401 (2010)

    Article  ADS  Google Scholar 

  20. Mortezapour, A., Franco, R.L.: Protecting quantum resources via frequency modulation of qubits in leaky cavities. Sci. Rep. 8(1–17), 14304 (2018)

    Article  ADS  Google Scholar 

  21. Man, Z.-X., An, N.B., Xia, Y.-J.: Non-Markovian dynamics of a two-level system in the presence of hierarchical environments. Optics. Exp. 23(1–14), 5763 (2015)

    Article  ADS  Google Scholar 

  22. Breuer, H.P., Laine, E.M., Piilo, J.: Measure for the Degree of Non-Markovian Behavior of Quantum Processes in Open Systems. Phys. Rev. Lett. 103(1–4), 210401 (2009)

    Article  ADS  MathSciNet  Google Scholar 

  23. Rivas, A., Huelga, S.F., Plenio, M.B.: Entanglement and non-Markovianity of quantum evolutions. Phys. Rev. Lett. 105(1–4), 050403 (2010)

    Article  ADS  MathSciNet  Google Scholar 

  24. Wolf, M.M., Cirac, J.I.: Dividing quantum channels. Commun. Math. Phys. 279, 147–168 (2008)

    Article  ADS  MathSciNet  MATH  Google Scholar 

  25. Breuer, H.P., Laine, E.M., Piilo, J., Vacchini, B.: Colloquium: non-markovian dynamics in open quantum systems. Rev. Mod. Phys. 88(1–24), 021002 (2016)

    Article  ADS  Google Scholar 

  26. Lu, X.-M., Wang, X., Sun, C.P.: Quantum Fisher information flow and non-Markovian processes of open systems. Phys. Rev. A 82(1–4), 042103 (2010)

    Article  ADS  Google Scholar 

  27. Luo, S., Fu, S., Song, H.: Quantifying non-Markovianity via correlations. Phys. Rev. A 86(1–4), 044101 (2012)

    Article  ADS  Google Scholar 

  28. Bylicka, B., Chruscinski, D., Maniscalco, S.: Non-Markovianity and reservoir memory of quantum channels: a quantum information theory perspective. Sci. Rep. 4(1–7), 5720 (2014)

    ADS  Google Scholar 

  29. Fanchini, F.F., Karpat, G., Cakmak, B., Castelano, L.K., Aguilar, G.H., Farias, O.J., Walborn, S.P., Ribeiro, P.H.S., de Oliveira, M.C.: Non-Markovianity through Accessible Information. Phys. Rev. Lett 112(1–6), 210402 (2014)

    Article  ADS  Google Scholar 

  30. He, Z., Yao, C., Wang, Q., Zou, J.: Measuring non-Markovianity based on local quantum uncertainty. Phys. Rev. A 90(1–7), 042101 (2014)

    Article  ADS  Google Scholar 

  31. Dhar, H.S., Bera, M.N., Adesso, G.: Characterizing non-Markovianity via quantum interferometric power. Phys. Rev. A 91(1–9), 032115 (2015)

    Article  ADS  Google Scholar 

  32. Liu, B.-H., Li, L., Huang, Y.-F., Li, C.-F., Guo, G.-C., Laine, E.M., Breuer, H.P., Piilo, J.: Experimental control of the transition from Markovian to non-Markovian dynamics of open quantum systems. Nature Physics. 7, 931–934 (2011)

    Article  ADS  Google Scholar 

  33. Apollaro, T.J.G., Franco, C.D., Plastina, F., Paternostro, M.: Memory-keeping effects and forgetfulness in the dynamics of a qubit coupled to a spin chain. Phys. Rev. A 83(1–10), 032103 (2011)

    Article  ADS  Google Scholar 

  34. Brito, F., Werlang, T.: A knob for Markovianity. New. J. Phys. 17(1–9), 072001 (2015)

    Article  ADS  Google Scholar 

  35. Lorenzo, S., Plastina, F., Paternostro, M.: Tuning non-Markovianity by spin-dynamics control. Phys. Rev. A 87(1–7), 022317 (2013)

    Article  ADS  Google Scholar 

  36. Addis, C., Ciccarello, F., Cascio, M., Palma, G.M., Maniscalco, S.: Dynamical decoupling efficiency versus quantum non-Markovianity. New. J. Phys. 17(1–11), 123004 (2015)

    Article  ADS  Google Scholar 

  37. Pang, S., Brun, T.A., Jordan, A.N.: Abrupt transitions between Markovian and non-Markovian dynamics in open quantum systems. arXiv:1712.10109 (2017)

  38. Wang, J., Wiseman, H.M., Milburn, G.J.: Dynamical creation of entanglement by homodyne-mediated feedback. Phys. Rev. A 71(1–9), 042309 (2005)

    Article  ADS  Google Scholar 

  39. Carvalho, A.R.R., Hope, J.J.: Stabilizing entanglement by quantum-jump-based feedback. Phys. Rev. A 76(1–4), 010301 (2007)

    Article  ADS  MathSciNet  Google Scholar 

  40. Hou, S.C., Huang, X.L., Yi, X.X.: Suppressing decoherence and improving entanglement by quantum-jump-based feedback control in two-level systems. Phys. Rev. A 82(1–6), 012336 (2010)

    Article  ADS  Google Scholar 

  41. Wiseman, H.M.: Quantum theory of continuous feedback. Phys. Rev. A 49, 2133–2150 (1994)

    Article  ADS  Google Scholar 

  42. Breuer, H.P.: Foundations and measures of quantum non-Markovianity. J. Phys. B. 45(1–12), 154001 (2012)

    Article  ADS  Google Scholar 

  43. Laine, E.M., Piilo, J., Breuer, H.P.: Measure for the non-Markovianity of quantum processes. Phys. Rev. A 81(1–8), 062115 (2010)

    Article  ADS  Google Scholar 

  44. Wißmann, S., Karlsson, A., Laine, E.M., Piilo, J., Breuer, H.P.: Optimal state pairs for non-Markovian quantum dynamics. Phys. Rev. A 86(1–6), 062108 (2012)

    Article  ADS  Google Scholar 

  45. Streltsov, A., Adesso, G., Plenio, M.B.: Colloquium: quantum coherence as a resource. Rev. Mod. Phys 89(1–34), 041003 (2017)

    Article  ADS  MathSciNet  Google Scholar 

  46. Hu, M.-L., Hu, X., Wang, J.-C., Peng, Y., Zhang, Y.-R., Fan, H.: Quantum coherence and geometric quantum discord. Phys. Rep. 762–764, 1–100 (2018)

    ADS  MathSciNet  MATH  Google Scholar 

  47. Zhang, D.J., Liu, C.L., Yu, X.D., Tong, D.M.: Estimating coherence measures from limited experimental data available. Phys. Rev. Lett. 120(1–6), 170501 (2018)

    Article  ADS  Google Scholar 

  48. Myatt, C.J., King, B.E., Turchette, Q.A., Sackett, C.A., Kielpinski, D., Itano, W.M., Monroe, C., Wineland, D.J.: Decoherence of quantum superpositions through coupling to engineered reservoirs. Nature 403, 269–273 (2000)

    Article  ADS  Google Scholar 

  49. Piilo, J., Maniscalco, S.: Driven harmonic oscillator as a quantum simulator for open system. Phys. Rev. A 74(1–11), 032303 (2006)

    Article  ADS  Google Scholar 

Download references

Acknowledgements

This work was supported by NSF-China under Grant Nos. 11904071, 11374085, the Key Program of the Education Department of Anhui Province under Grant Nos. KJ2017A922, KJ2019A0725, the Anhui Provincial Natural Science Foundation under Grant Nos. 1908085QA40, 1708085MA12, 1708085MA10, the discipline top-notch talents Foundation of Anhui Provincial Universities under Grants Nos.gxbjZD2017024, gxbjZD2016078, the Anhui Provincial Candidates for academic and technical leaders Foundation under Grant No. 2019H208.

Author information

Authors and Affiliations

  1. Institute for Quantum Control and Quantum Information, School of Physics and Materials Engineering, Hefei Normal University, Hefei, 230601, China

    Xiao-Lan Zong, Wei Song & Zhuo-Liang Cao

  2. School of Physics and Material Science, Anhui University, Hefei, 230601, China

    Ming Yang

Authors
  1. Xiao-Lan Zong
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Wei Song
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Ming Yang
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Zhuo-Liang Cao
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Wei Song.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Zong, XL., Song, W., Yang, M. et al. Enhancing non-Markovianity by quantum feedback control. Quantum Inf Process 19, 131 (2020). https://doi.org/10.1007/s11128-020-02629-1

Download citation

  • Received:

  • Accepted:

  • Published:

  • DOI: https://doi.org/10.1007/s11128-020-02629-1

Keywords

Search

Navigation