Abstract
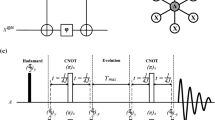
Based on a quantum interferometric circuit, we implement a NMR quantum thermometer, in which a probe qubit measures the temperature of a nuclear spin at thermal equilibrium with a bath. The whole procedure lasts 5.5 ms, a much shorter time than the probe’s spin-lattice relaxation time, which is \(T_{1}=7.0\,\hbox {s}\). The fidelity of the probe final quantum state, in respect to the ideal theoretical prediction, is above 99 %. We show that quantum coherence is essential for the high fidelity of temperature measurement. We discuss the source of errors on the temperature measurement and some possible applications of the thermometer.
This is a preview of subscription content, log in via an institution to check access.
Similar content being viewed by others
Explore related subjects
Discover the latest articles, news and stories from top researchers in related subjects.References
Stace, T.M.: Quantum limits of thermometry. Phys. Rev. A 82, 011611 (2010)
Brunelli, M., Olivares, S., Paternostro, M., Paris, M.G.A.: Qubit-assisted thermometry of a quantum harmonic oscillator. Phys. Rev. A 86, 012125 (2012)
Martín-Martínez, E., Dragan, A., Mann, R.B., Fuentes, I.: Berry phase quantum thermometer. New J. Phys. 15, 053036 (2013)
Higgins, K.D.B., Lovett, B.W., Gauger, E.M.: Quantum thermometry using the ac Stark shift within the Rabi model. Phys. Rev. B 88, 155409 (2013)
Neumann, P., Jakobi, I., Dolde, F., Burk, C., Reuter, R., Waldherr, G., Honert, J., Wolf, T., Brunner, A., Shim, J.H., Suter, D., Sumiya, H., Isoya, J., Wrachtrup, J.: High-precision nanoscale temperature sensing using single defects in diamond. Nano Letters 13, 2738 (2013)
Toyli, D.M., de las Casas, C.F., Christle, D.J., Dobrovitskib, V.V., Awschalom, D.D.: Fluorescence thermometry enhanced by the quantum coherence of single spins in diamond. PNAS 110, 8417 (2013)
Souza, A.M., Oliveira, I.S., Sarthour, R.S.: A scattering quantum circuit for measuring Bell’s time inequality: a nuclear magnetic resonance demonstration using maximally mixed states. New J. Phys. 13, 053023 (2011)
Negrevergne, C., Somma, R., Ortiz, G., Knill, E., Laflamme, R.: Liquid-state NMR simulations of quantum many-body problems. Phys. Rev. A 71, 032344 (2005)
Leonhardt, U.: Quantum-state tomography and discrete Wigner function. Phys. Rev. Lett. 74, 4101 (1995)
Miquel, C., Paz, J.P., Saraceno, M., Knill, E., Laflamme, R., Negrevergne, C.: Interpretation of tomography and spectroscopy as dual forms of quantum computation. Nature 418, 59–62 (2002)
Batalhão, T., Souza, A.M., Mazzola, L., Auccaise, R., Oliveira, I.S., Goold, J., Chiara, G.D., Paternostro, M., Serra, R.M.: Experimental reconstruction of work distribution and verification of fluctuation relations at the full quantum level. Phys. Rev. Lett. 113, 140601 (2014)
Oliveira, I.S., Bonagamba, T.J., Sarthour, R.S., Freitas, J.C.C., de Azevedo, E.R.: NMR Quantum Information Processing. Elsevier, Amsterdam (2007)
Fukushima, E., Roeder, S.B.W.: Experimental Pulse NMR. Addison-Wesley Publishing Company, London (1993)
Lee, J.-S.: The quantum state tomography on an NMR system. Phys. Lett. A 305, 349–353 (2002)
Raiford, D.S., Fisk, C.L., Becker, E.D.: Calibration of methanol and ethylene glycol nuclear magnetic resonance thermometers. Anal. Chem. 51, 2050 (1979)
Acknowledgments
We acknowledge suggestions from E. R. de Azevedo and S. O. Soares-Pinto from USP/São Carlos, and from L. C. Céleri from UFG, and financial support from CAPES, FAPERJ, and INCT of Quantum Information.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Raitz, C., Souza, A.M., Auccaise, R. et al. Experimental implementation of a nonthermalizing quantum thermometer. Quantum Inf Process 14, 37–46 (2015). https://doi.org/10.1007/s11128-014-0858-z
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s11128-014-0858-z