Pseudo random sequences based on elliptic curve subgroups and mathematical model for its application to digital image security | Multimedia Tools and Applications Skip to main content
Springer Nature Link
Log in

Pseudo random sequences based on elliptic curve subgroups and mathematical model for its application to digital image security

  • Published:
Multimedia Tools and Applications Aims and scope Submit manuscript

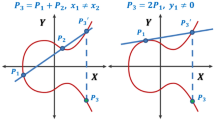
Abstract

The security strength of the elliptic curve cryptosystems (ECC) is due to its core operations-based group law. This aspect of the elliptic curve provides key service to ensure security against modern cryptanalysis. However, the excess use of group law in EC based algorithms make it computationally hard for real time applications. In this context, this paper presented a smart-like algorithm based on subgroup co-set operations. The suggested scheme uses all co-sets that generates multiple sequences that can smoothly be adopted in most promising communication architectures of the future such as internet of things (IoT). Besides, the subgroup structure on a small prime with possible embedding is managed to construct efficient substitution box (S-box). Whereas, the performance of the proposed S-box is examined via standardized tests thus found significant for multimedia data security applications. Moreover, a small prime based EC subgroup coset model is designed, that generates a set of experimentally verified independent pseudo random streams. The atypical mathematical model for its application to image data encryption is established, by combining the S-box module (SM) and subgroup coset module (ES-PM). Several statistical tests revealed that the proposed technique is suitable for various cryptographic applications.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9

Similar content being viewed by others

References

  1. Abd el-Latif AA, Abd-el-Atty B, Amin M, Iliyasu AM (2020) Quantum-inspired cascaded discrete-time quantum walks with induced chaotic dynamics and cryptographic applications. Sci Rep 10(1):1–16

    Article  Google Scholar 

  2. Abd El-Latif AA, Niu X (2013) A hybrid chaotic system and cyclic elliptic curve for image encryption. AEU-Int J Electr Commun 67(2):136–143

    Article  Google Scholar 

  3. Adams C, Tavares S (1990) The structured Design of Cryptographically Good S-boxes. J Cryptol 3:27–41

    Article  MathSciNet  Google Scholar 

  4. Akhshani A, Akhavan A, Mobaraki A, Lim SC, Hassan Z (2014) Pseudo random number generator based on quantum chaotic map. Commun Nonlinear Sci Numer Simul 19(1):101–111

    Article  Google Scholar 

  5. Azimi Z, Ahadpour S (2020) Color image encryption based on DNA encoding and pair coupled chaotic maps. Multimed Tools Appl 79(3):1727–1744

    Article  Google Scholar 

  6. Bansal M, Kumar M, Kumar M, Kumar K (2021) An efficient technique for object recognition using Shi-Tomasi corner detection algorithm. Soft Comput 25(6):4423–4432

    Article  Google Scholar 

  7. Brown J, Zhang JF, Zhou B, Mehedi M, Freitas P, Marsland J, Ji Z (2020) Random-telegraph-noise-enabled true random number generator for hardware security. Sci Rep 10(1):1–13

    Article  Google Scholar 

  8. Çavuşoğlu Ü, Zengin A, Pehlivan I, Kaçar S (2017) A novel approach for strong S-box generation algorithm design based on chaotic scaled Zhongtang system. Nonlinear Dyn 87(2):1081–1094

    Article  Google Scholar 

  9. Chhabra P, Garg NK, Kumar M (2020) Content-based image retrieval system using ORB and SIFT features. Neural Comput & Applic 32(7):2725–2733

    Article  Google Scholar 

  10. Farwa S, Bibi N, Muhammad N (2020) An efficient image encryption scheme using Fresnelet transform and elliptic curve based scrambling. Multimed Tools Appl 79(37):28225–28238

    Article  Google Scholar 

  11. Gallian J (2010) Contemporary Abstract Algebra , Cengage Learning, Exercise 43 (p. 84). ISBN 978-0-547-16509-7

  12. Garg D, Garg NK, Kumar M (2018) Underwater image enhancement using blending of CLAHE and percentile methodologies. Multimed Tools Appl 77(20):26545–26561

    Article  Google Scholar 

  13. Haider MI, Ali A, Shah D, Shah T (2020) Block cipher’s nonlinear component design by elliptic curves: an image encryption application. Multimed Tools Appl 1–26

  14. Hussain I, Shah T, Mahmood H, Gondal MA (2013) A projective general linear group based algorithm for the construction of substitution box for block ciphers. Neural Comput & Applic 22(6):1085–1093

    Article  Google Scholar 

  15. Ibrahim S, Alharbi A (2020) Efficient image encryption scheme using Henon map, dynamic S-boxes and elliptic curve cryptography. IEEE Access 8:194289–194302

    Article  Google Scholar 

  16. Jia N, Liu S, Ding Q, Wu S, Pan X (2016) A new method of encryption algorithm based on Chaos and ECC. J Inf Hiding Multimed Signal Process 7(3):637–643

    Google Scholar 

  17. Jiang H, Belkin D, Savel’ev SE, Lin S, Wang Z, Li Y, … Barnell M (2017) A novel true random number generator based on a stochastic diffusive memristor. Nat Commun 8(1):1–9

    Article  Google Scholar 

  18. Koblitz N (1987) Elliptic curve cryptosystems. Math Comput 48(177):203–209

    Article  MathSciNet  Google Scholar 

  19. Kumar M, Bansal M, Kumar M (2020) 2D object recognition techniques: state-of-the-art work. Arch Comput Methods Eng 2

  20. Kumar M, Chhabra P, Garg NK (2018) An efficient content based image retrieval system using BayesNet and K-NN. Multimed Tools Appl 77(16):21557–21570

    Article  Google Scholar 

  21. Lai X, Massey JL (1990). A proposal for a new block encryption standard. In workshop on the theory and application of of cryptographic techniques (pp 389-404). Springer, Berlin, Heidelberg

  22. Li W, Chang X, Yan A, Zhang H (2021) Asymmetric multiple image elliptic curve cryptography. Opt Lasers Eng 136:106319

    Article  Google Scholar 

  23. Liu Q, Liu L (2020) Color image encryption algorithm based on DNA coding and double Chaos system. IEEE Access 8:83596–83610

    Article  Google Scholar 

  24. Liu G, Yang W, Liu W, Dai Y (2015) Designing S-boxes based on 3-D four-wing autonomous chaotic system. Nonlinear Dyn 82(4):1867–1877

    Article  MathSciNet  Google Scholar 

  25. Matsui M (1993) Linear cryptanalysis method of DES cipher. In advances in cryptology, proceedings of the workshop on the theory and application of of cryptographic techniques (EURO-CRYPT-93), Lofthus, Norway, 23–27; Springer: Berlin/Heidelberg, German

  26. Miller VS (1985) Use of elliptic curves in cryptography. In conference on the theory and application of cryptographic techniques (pp. 417-426). Springer, Berlin, Heidelberg

  27. Monika MK, Kumar M (2020) XGBoost: 2D-object recognition using shape descriptors and extreme gradient boosting classifier. Computational methods and data engineering: proceedings of ICMDE 2020, volume 1, 1227, 207

  28. Nian-Sheng L (2011) Pseudo-randomness and complexity of binary sequences generated by the chaotic system. Commun Nonlinear Sci Numer Simul 16(2):761–768

    Article  MathSciNet  Google Scholar 

  29. Özkaynak F (2019) Construction of robust substitution boxes based on chaotic systems. Neural Comput & Applic 31(8):3317–3326

    Article  Google Scholar 

  30. Özkaynak F, Çelik V, Özer AB (2017) A new S-box construction method based on the fractional-order chaotic Chen system. SIViP 11(4):659–664

    Article  Google Scholar 

  31. Ramesh A, Jain A (2015) Hybrid image encryption using Pseudo random number generators, and transposition and substitution techniques. In 2015 international conference on trends in automation, communications and computing technology (I-TAC

  32. Ran Q, Wang L, Ma J, Tan L, Yu S (2018) A quantum color image encryption scheme based on coupled hyper-chaotic Lorenz system with three impulse injections. Quantum Inf Process 17(8):188

    Article  MathSciNet  Google Scholar 

  33. Reyad O, Kotulski Z (2015) Image encryption using koblitz’s encoding and new mapping method based on elliptic curve random number generator. In international conference on multimedia communications, services and security (pp. 34-45). Spri

  34. Reyad O, Kotulski Z, Abd-Elhafiez WM (2016) Image encryption using chaos-driven elliptic curve pseudo-random number generators. Appl Math Inf Sci 10(4):1283–1292

    Article  Google Scholar 

  35. Shah T, Ali A, Khan M, Farooq G, de Andrade AA (2020) Galois ring $$ GR\left ({2^{3}, 8}\right) $$ GR23, 8 dependent $ $24\times 24$ $24× 24 S-box design: an RGB image encryption application. Wirel Pers Commun 113(2):1201–1201

  36. Shah D, Shah T (2020) A novel discrete image encryption algorithm based on finite algebraic structures. Multimed Tools Applic 1–20

  37. Shah D, Shah T (2020) Binary Galois field extensions dependent multimedia data security scheme. Microprocess Microsyst 77:103181

    Article  Google Scholar 

  38. Shah D, Shah T, Jamal SS (2019) A novel efficient image encryption algorithm based on affine transformation combine with linear fractional transformation. Multidimensional Syst Signal Process 1–21

  39. Shannon CE (1949) Communication theory of secrecy systems. Bell Syst Tech J 28(4):656–715

    Article  MathSciNet  Google Scholar 

  40. Te Chiang Y, Wang HS, Wang YN (2013) A chaotic-based Pseudo-random bit generator for navigation applications. Appl Mech Mater 311:99–104

    Article  Google Scholar 

  41. Toughi S, Fathi MH, Sekhavat YA (2017) An image encryption scheme based on elliptic curve pseudo random and advanced encryption system. Signal Process 141:217–227

    Article  Google Scholar 

  42. ul Haq T, Shah T (2020) 12× 12 S-box design and its application to RGB image encryption. Optik 164922:164922. https://doi.org/10.1016/j.ijleo.2020.164922

    Article  Google Scholar 

  43. ul Haq T, Shah T (2020) Algebra-chaos amalgam and DNA transform based multiple digital image encryption. J Inf Secur Appl 54:102592

    Google Scholar 

  44. USC-SIPI. Image database. Available at http://sipi.usc.edu/database/database.php

  45. Wang X, Çavuşoğlu Ü, Kacar S, Akgul A, Pham VT, Jafari S, Alsaadi F, Nguyen XQ (2019) S-box based image encryption application using a chaotic system without equilibrium. Appl Sci 9(4):781

    Article  Google Scholar 

  46. Wang X, Guan N, Zhao H, Wang S, Zhang Y (2020) A new image encryption scheme based on coupling map lattices with mixed multi-chaos. Sci Rep 10(1):1–15

    Article  Google Scholar 

  47. Wang Y, Liu Z, Ma J, He H (2016) A pseudorandom number generator based on piecewise logistic map. Nonlinear Dyn 83(4):2373–2391

    Article  MathSciNet  Google Scholar 

  48. Washington LC (2008) Elliptic curves: number theory and cryptography. CRC Press

  49. Webster A, Tavares SE (1985) On the design of S-boxes. In Conference on the Theory and Application of Cryptographic Techniques; Springer: Berlin/Heidelberg, Germany; pp 523–534

  50. Xuejing K, Zihui G (2020) A new color image encryption scheme based on DNA encoding and spatiotemporal chaotic system. Signal Process Image Commun 80:115670

    Article  Google Scholar 

  51. Ye G, Jiao K, Huang X, Goi BM, Yap WS (2020) An image encryption scheme based on public key cryptosystem and quantum logistic map. Sci Rep 10(1):1–19

    Article  Google Scholar 

  52. Ye T, Zhimao L (2018) Chaotic S-box: six-dimensional fractional Lorenz–duffing chaotic system and O-shaped path scrambling. Nonlinear Dyn 94(3):2115–2126

    Article  Google Scholar 

  53. Yu SS, Zhou NR, Gong LH, Nie Z (2020) Optical image encryption algorithm based on phase-truncated short-time fractional Fourier transform and hyper-chaotic system. Opt Lasers Eng 124:105816

    Article  Google Scholar 

  54. Zahid AH, Arshad MJ (2019) An innovative design of substitution-boxes using cubic polynomial mapping. Symmetry 11(3):437

    Article  Google Scholar 

  55. Zhang YQ, He Y, Li P, Wang XY (2020) A new color image encryption scheme based on 2DNLCML system and genetic operations. Opt Lasers Eng 128:106040

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Mathematics, Quaid-i-Azam University, Islamabad, Pakistan

    Muhammad Imran Haider, Tariq Shah, Asif Ali, Dawood Shah & Ijaz Khalid

Authors
  1. Muhammad Imran Haider
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Tariq Shah
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Asif Ali
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Dawood Shah
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Ijaz Khalid
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Muhammad Imran Haider.

Ethics declarations

Conflict of interest

The authors declare no conflict of interest

Additional information

Publisher’s note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Haider, M.I., Shah, T., Ali, A. et al. Pseudo random sequences based on elliptic curve subgroups and mathematical model for its application to digital image security. Multimed Tools Appl 81, 23709–23734 (2022). https://doi.org/10.1007/s11042-022-12358-5

Download citation

  • Received:

  • Revised:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s11042-022-12358-5

Keywords

Search

Navigation