The role of linear and voltage-dependent ionic currents in the generation of slow wave oscillations

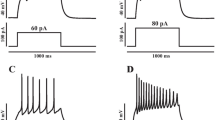
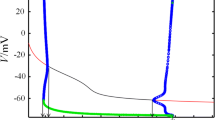
Abstract

Neuronal oscillatory activity is generated by a combination of ionic currents, including at least one inward regenerative current that brings the cell towards depolarized voltages and one outward current that repolarizes the cell. Such currents have traditionally been assumed to require voltage-dependence. Here we test the hypothesis that the voltage dependence of the regenerative inward current is not necessary for generating oscillations. Instead, a current I _NL that is linear in the biological voltage range and has negative conductance is sufficient to produce regenerative activity. The current I _NL can be considered a linear approximation to the negative-conductance region of the current–voltage relationship of a regenerative inward current. Using a simple conductance-based model, we show that I _NL , in conjunction with a voltage-gated, non-inactivating outward current, can generate oscillatory activity. We use phase-plane and bifurcation analyses to uncover a rich variety of behaviors as the conductance of I _NL is varied, and show that oscillations emerge as a result of destabilization of the resting state of the model neuron. The model shows the need for well-defined relationships between the inward and outward current conductances, as well as their reversal potentials, in order to produce stable oscillatory activity. Our analysis predicts that a hyperpolarization-activated inward current can play a role in stabilizing oscillatory activity by preventing swings to very negative voltages, which is consistent with what is recorded in biological neurons in general. We confirm this prediction of the model experimentally in neurons from the crab stomatogastric ganglion.

Appendix

We used the following set of equations for simulations associated with Figs. 4, 5, 6, 7, and 8.

$$ \begin{array}{l}v^{\prime }=\left[{I}_{ext}-{I}_{NL}^{*}(v)-{I}_K\left(v,w\right)-{I}_h\left(v,h\right)\right]/{C}_m\hfill \\ {}w^{\prime }=\frac{w_{\infty }(v)-w}{\tau_K(v)}\hfill \\ {}h^{\prime }=\frac{h_{\infty }(v)-h}{\tau_h(v)}\hfill \end{array} $$

where

$$ \begin{array}{l}{I}_K\left(v,w\right)={g}_Kw\left[v-{E}_K\right]\hfill \\ {}{I}_{NL}^{*}=\left\{\begin{array}{l}{g}_{NL}\left[v-{E}_{NL}\right] Heav\left(v-{E}_{NL}\right),\mathrm{for}\ \mathrm{Fig}\mathrm{s}.4,5,6,7\ \mathrm{and}\ 8\\ {}{g}_{NL}\left[v-{E}_{NL}\right],\mathrm{for}\ \mathrm{Fig}.8\end{array}\right.\hfill \\ {}{I}_h={g}_hh\left[v-{E}_h\right]\hfill \end{array} $$

The gating functions are

$$ \begin{array}{l}{w}_{\infty }(v)=1/\left[1+ \exp \left(-\left[v-{w}_{mid}\right]/{k}_1\right)\right]\hfill \\ {}{h}_{\infty }(v)=1/\left[1+ \exp \left(\left[v-{h}_{mid}\right]/{h}_1\right)\right]\hfill \end{array} $$

and the time constants are given by τ _K (v) = τ ₁/[1 + exp(v/k _s )] and τ _h (v) = τ _h1 ms. The following parameter values were used in all simulations E _K  = − 80 mV, g _K  = 0.5, I _ext  = 0, and C _m  = 1.0 nF

In Figs. 4 and 5 there was no h current, which we modeled simply by setting g _h  = 0 μS. We let E _NL  = − 79 mV, w _mid  = − 60 mV, k _s  = 2 mV. For Fig. 4, we used k ₁ = 2 mV and τ ₁ = 60 ms. For Fig. 5a, we used k ₁ = 4 mV and τ ₁ = 80 ms. For Fig. 5b, we used k ₁ = 4 mV and τ ₁ = 60 ms.

In Fig. 6, an instantaneous version of the h current was used, i.e., the equation for h ′ was replaced with h ≡ h _∞ (v). For this figure, parameter values from Fig. 5a were used. In addition, E _h  = − 30 mV, h _mid  = − 85 mV, h ₁ = 2 mV, E _NL  = −75 mV and g _NL  = − 0.15 μS. Figure 6b was obtained by varying h _mid while g _h  = 1 μS was fixed.

In Fig. 7, we used parameter values from Fig. 5a with g _NL  = − 0.45 μS, E _NL  = −75 mV, g _h  = 1. The perturbation was achieved be setting I _ext  = −1 for 50 mS.

In Fig. 8, we used C _m  = 10.0 nF, τ _h1 = 200 ms, τ ₁ = 600 ms to match the biological timescale. Additionally, we set τ _K (v) = τ ₁/cosh((v − w _mid ))/k _s ) with k _s  = 5.6 mV, g _NL  = − 0.45 μS, E _NL  = − 65 mV, w _mid  = − 40 mV, g _h  = 0.8 μS and h ₁ = 6 mV.