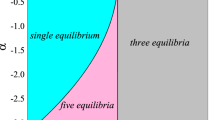
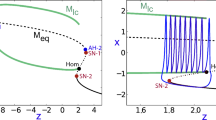
Abstract
The leech neuron model studied here has a remarkable dynamical plasticity. It exhibits a wide range of activities including various types of tonic spiking and bursting. In this study we apply methods of the qualitative theory of dynamical systems and the bifurcation theory to analyze the dynamics of the leech neuron model with emphasis on tonic spiking regimes. We show that the model can demonstrate bi-stability, such that two modes of tonic spiking coexist. Under a certain parameter regime, both tonic spiking modes are represented by the periodic attractors. As a bifurcation parameter is varied, one of the attractors becomes chaotic through a cascade of period-doubling bifurcations, while the other remains periodic. Thus, the system can demonstrate co-existence of a periodic tonic spiking with either periodic or chaotic tonic spiking. Pontryagin’s averaging technique is used to locate the periodic orbits in the phase space.
Similar content being viewed by others
References
Arnold VI, Afrajmovich VS, Ilyashenko YuS, Shilnikov LP (1994) Bifurcation Theory, Dynamical Systems V. Encyclopaedia of Mathematical Sciences. Springer-Verlag.
Bazhenov M, Timofeev I, Steriade M, Sejnowski TJ (2000) Spiking-bursting activity in the thalamic reticular nucleus initiates sequences of spindle oscillations in thalamic networks. J. Neurophysiology 84: 1076–1087.
Bertram R (1993) A computational study of the effectsof serotonin on a Molluscan Burster Neuron. Biol. Cybern. 69: 257–267.
Borisyuk RM, Kazanovich YB (2004) Oscillatory model of attention-guided object selection and novelty detection. Neural Netw. 17: 899–915.
Canavier CC, Baxter DA, Clark L, Byrne J (1993) Nonlinear dynamics in a model neuron provide a novel mechanism for transient synaptic inputs to produce long-term alterations of postsynaptic activity. J. Neurophysiol. 69: 2252.
Canavier CC, Baxter DA, Clark JW, Byrne JH (1994) Multiple modes of activity in a model neuron suggest a novel mechanism for the effects of neuromodulators. Multiple modes of activity in a model neuron suggest a novel mechanism for the effects of neuromodulators. J. Neurophysiol. 72: 872–882.
Cymbalyuk GS, Calabrese RL (2001) A model of slow plateau-like oscillations based upon the fast Na+ current in a window mode. Neurocomputing 38–40: 159–166.
Cymbalyuk GS, Gaudry Q, Masino MA, Calabrese RL (2002) A model of a segmental oscillator in the leech heartbeat neuronal network. J. Neuroscience 22: 10580.
Feudel U, Neiman A, Pei X, Wojtenek W, Braun H, Huber M, Moss F (2000) Homoclinic bifurcation in a Hodgkin-Huxley model of thermally sensitive neurons. Chaos 10(1): 231–239.
Gray CM, Singer W (1989) Stimulus-specific neuronal oscillations in orientation columns of cat visual cortex. Proc. Natl. Acad. Sci. USA. 86: 1698–1702.
Guckenheimer J, Hofman K, Weckesserand W (2000) Numerical computations of canards. Int. J. Bifurcation and Chaos 2: 2669–2689.
Hill A, Lu J, Masino M, Olsen O, Calabrese RL (2001) A model of a segmental oscillator in the leech heartbeat neuronal network. J. Comput. Neuroscience 10, 281.
Hodgkin AL, Huxley AF (1952) A quantitative description of membrane current and its application to conduction and excitation in nerve. J. Physiol. 117: 500.
Hopfield JJ, Brody CD (2001) What is a moment? Transient synchrony as a collective mechanism for spatiotemporal integration. Proc. Natl. Acad. Sci. USA. 98: 1282–1287.
Hoppensteadt FC, Izhikevich EM (1998) Thalamo-cortical interactions modeled by weakly connected oscillators: Could the brain use FM radio principles? Biosystems. 48: 85–94.
Izhikevich EM (2000) Neural Excitability, spiking, and bursting. international. journalof bifurcation and Chaos. 10: 1171–1266.
Hounsgaard J, Kiehn O (1989) Serotonin-induced bistability of turtle motoneurones caused by a nifedipine-sensitive calcium plateau potential. J. Physiol. 414: 265–282.
CONTENT is available at ftp://ftp.cwi.nl/pub/CONTENT.
Lechner H, Baxter D, Clark C, Byrne J (1996) Bistability and its regulation by serotonin in the endogenously bursting neuron R15 in Aplysia. J. Neurophysiol. 75: 957.
Marder E, Calabrese RL (1996) Principles of rhythmic motor pattern generation. Physiol. Rev. 76: 687.
Marder E, Abbott L, Turrigiano G, Liu Z, Golowasch J (1996) Memory from the dynamics of intrinsic membrane currents. Proc. Natl. Acad. Sci. USA 26–93(24), 13481.
Opdyke CA, Calabrese RL (1994) A persistent sodium current contributes to oscillatory activity in heart interneurons of the medicinal leech. J. Comp. Physiol. 175: 781–789.
Pontryagin LS, Rodygin LV (1960) Periodic solution of a system of ordinary differential equations with a small parameter in the terms containing derivatives. Sov. Math. Dokl. 1: 611–614.
Rinzel J, Ermentrout B (1989) Analysis of neural excitability and oscillations. In: C. Koch and I. Segev, eds., Methods of Neural Modeling: From Synapses to Networks. MIT Press, pp. 135– 169.
Rowat PF, Elson RC (2004) State-dependent effects of Na channel noise on meuronal burst generation, J. of Computational Neuroscience 16: 87–112.
Schwarz C, Thier P (1999) Binding of signals relevant for action: Towards a hypothesis of the functional role of the pontine nuclei. Trends Neurosci. 22: 443–451.
Shilnikov AL, Cymbalyuk G (2005) Transition between tonic spiking and bursting in a neuron model via the blue-sky catastrophe. Phys Rev Letters 94: 048101.
Shilnikov AL, Calabrese RL, and Cymbalyuk GS (2004) Mechanism of bi-stability: Tonic spiking and bursting in a neuron model. Phys. Rev. E. (submitted).
Shilnikov AL, Calabrese RL, Cymbaluyk G (2005) How a neuron model can demonstrate coexistence of tonic spiking and bursting? Neurocomputing (accepted for publication).
Shilnikov LP, Shilnikov AL, Turaev DV, Chua LO (1998, 2001) Methods Qualitative Theory in Nonlinear Dynamics, Volumes I and II. World Sci. Publ.
Shilnikov AL, Rulkov NF (2003) Origin of chaos in a two-dimensional map modelling spiking-bursting neural activity. Bifurcations and Chaos 13(11): 3325–3340.
Shilnikov AL, Rulkov NF (2004) Subthreshold oscillations in a map-based neuron model. Physics Letters A328: 177–184.
Shilnikov AL, Shilnikov LP, Turaev DV (2004) Mathematical aspects of classical synchronization theory, Tutorial. Bifurcations and Chaos 14(7): 2143–2160.
Terman D (1992) The transition from bursting to continuous spiking in an excitable membrane model. J. Nonlinear Science 2, 135– 182.
Turrigiano G, Marder E, Abbott L (1996) Cellular short-term memory from a slow potassium conductance. J. Neurophysiol. 75: 963.
Vinogradova OS. (2001) Hippocampus as comparator: Role of the two input and two output systems of the hippocampus in selection and registration of information. Hippocampus. 11(5): 578– 598.
Wang XJ (1993) Genesis of bursting oscillations in the Hindmarsh-Rose model and homoclinicity to a chaotic saddle. Physica D 62: 263–274.
Author information
Authors and Affiliations
Corresponding author
Rights and permissions
About this article
Cite this article
Cymbalyuk, G., Shilnikov, A. Coexistence of Tonic Spiking Oscillations in a Leech Neuron Model. J Comput Neurosci 18, 255–263 (2005). https://doi.org/10.1007/s10827-005-0354-7
Received:
Revised:
Accepted:
Issue Date:
DOI: https://doi.org/10.1007/s10827-005-0354-7