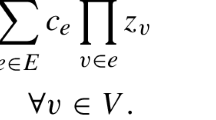Weighted stability number of graphs and weighted satisfiability: The two facets of pseudo-Boolean optimization | Annals of Operations Research Skip to main content
Springer Nature Link
Log in

Weighted stability number of graphs and weighted satisfiability: The two facets of pseudo-Boolean optimization

  • Published:
Annals of Operations Research Aims and scope Submit manuscript

Abstract

We exhibit links between pseudo-Boolean optimization, graph theory and logic. We show the equivalence of maximizing a pseudo-Boolean function and finding a maximum weight stable set; symmetrically minimizing a pseudo-Boolean function is shown to be equivalent to solving a weighted satisfiability problem.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  • Alexe, A., P.L. Hammer, V.V. Lozin, and D. de Werra. (2004). “Struction Revisited.” Discrete Applied Mathematics, 132, 27–46.

    Article  Google Scholar 

  • Berge, C. (1973). Graphs and Hypergraphs. North-Holland: Amsterdam.

    Google Scholar 

  • Ebenegger, C., P.L. Hammer, and D. de Werra. (1984). “Pseudoboolean Functions and Stability of Graphs.” Annals of Discrete Mathematics, 19, 83–98.

    Google Scholar 

  • Hamor, A. (1980). “Stories of the One-Zero-One Nights: Abou Boul in Graphistan.” In P. Hansen and D. de Werra (Eds.), Regards sur la théorie des graphes. Lausanne, Presses Polytechniques Romandes, pp. 29–38.

  • Hertz, A. (1995). “Polynomially Solvable Cases for the Maximum Stable Set Problem.” Discrete Applied Mathematics, 60, 195–210.

    Article  Google Scholar 

  • Hertz, A. (1985). “On the Use of Boolean Methods for the Computation of the Stability Number.” Discrete Applied Mathematics, 76, 183–203.

    Article  Google Scholar 

  • Garey, M.R. and D.S. Johnson. (1979). Computers and Intractability: A Guide to the Theory of NP-Completeness. New York, Freeman.

    Google Scholar 

  • Hammer, P.L., N.V.R. Mahadev, and D. de Werra. (1985). “Stability in CAN-Free Graphs.” J. Combin. Theory, B38, 23–30.

    Google Scholar 

  • Hammer, P.L., N.V.R. Mahadev, and D. de Werra. (1985). “The Struction of a Graph: Applications to CN-Free Graphs.” Combinatorica, 5, 141–147.

    Google Scholar 

Download references

Author information

Authors and Affiliations

  1. IMA - EPFL, Lausanne, Suisse, Switzerland

    D. de Werra

  2. RUTCOR, Rutgers University, 640 Bartholomew Rd, Piscataway, NJ, 088254-8003, USA

    P. L. Hammer

Authors
  1. D. de Werra
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. P. L. Hammer
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to D. de Werra.

Rights and permissions

Reprints and permissions

About this article

Cite this article

de Werra, D., Hammer, P.L. Weighted stability number of graphs and weighted satisfiability: The two facets of pseudo-Boolean optimization. Ann Oper Res 149, 67–73 (2007). https://doi.org/10.1007/s10479-006-0101-0

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s10479-006-0101-0

Keywords

Search

Navigation