A family of C¹ quadrilateral finite elements

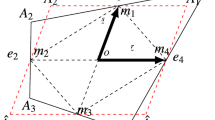
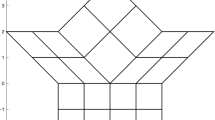
Abstract

We present a novel family of C¹ quadrilateral finite elements, which define global C¹ spaces over a general quadrilateral mesh with vertices of arbitrary valency. The elements extend the construction by Brenner and Sung (J. Sci. Comput. 22(1-3), 83-118, 2005), which is based on polynomial elements of tensor-product degree p ≥ 6, to all degrees p ≥ 3. The proposed C¹ quadrilateral is based upon the construction of multi-patch C¹ isogeometric spaces developed in Kapl et al. (Comput. Aided Geometr. Des. 69, 55–75 2019). The quadrilateral elements possess similar degrees of freedom as the classical Argyris triangles, developed in Argyris et al. (Aeronaut. J. 72(692), 701–709 1968). Just as for the Argyris triangle, we additionally impose C² continuity at the vertices. In contrast to Kapl et al. (Comput. Aided Geometr. Des. 69, 55–75 2019), in this paper, we concentrate on quadrilateral finite elements, which significantly simplifies the construction. We present macro-element constructions, extending the elements in Brenner and Sung (J. Sci. Comput. 22(1–3), 83–118 2005), for polynomial degrees p = 3 and p = 4 by employing a splitting into 3 × 3 or 2 × 2 polynomial pieces, respectively. We moreover provide approximation error bounds in $L^{\infty }$, L², H¹ and H² for the piecewise-polynomial macro-element constructions of degree p ∈{3,4} and polynomial elements of degree p ≥ 5. Since the elements locally reproduce polynomials of total degree p, the approximation orders are optimal with respect to the mesh size. Note that the proposed construction combines the possibility for spline refinement (equivalent to a regular splitting of quadrilateral finite elements) as in Kapl et al. (Comput. Aided Geometr. Des. 69, 55–75 30) with the purely local description of the finite element space and basis as in Brenner and Sung (J. Sci. Comput. 22(1–3), 83–118 2005). In addition, we describe the construction of a simple, local basis and give for p ∈{3,4,5} explicit formulas for the Bézier or B-spline coefficients of the basis functions. Numerical experiments by solving the biharmonic equation demonstrate the potential of the proposed C¹ quadrilateral finite element for the numerical analysis of fourth order problems, also indicating that (for p = 5) the proposed element performs comparable or in general even better than the Argyris triangle with respect to the number of degrees of freedom.

Appendices

Appendix A: Pull-backs of derivatives

In the following, we derive the pull-backs of the partial derivatives ∂₁φ, ∂₂φ, $\partial _{1}^{2}\varphi$, ∂₁∂₂φ and $\partial _{2}^{2}\varphi$, i.e., their representations in parameters (ξ₁,ξ₂). We have that

$$ \varphi \circ \mathbf{F}_{Q} = \widehat{\varphi} $$

and, after applying the chain rule, obtain

$$ \left( \begin{array}{cc} \partial_{1}\varphi\circ\mathbf{F}_{Q} & \partial_{2}\varphi\circ\mathbf{F}_{Q} \end{array}\right) \left( \begin{array}{cc} \partial_{1}\mathrm{F}_{Q,1} & \partial_{2}\mathrm{F}_{Q,1} \\ \partial_{1}\mathrm{F}_{Q,2} & \partial_{2}\mathrm{F}_{Q,2} \end{array}\right) = \left( \begin{array}{cc} \partial_{1}\widehat{\varphi} & \partial_{2}\widehat{\varphi} \end{array}\right) $$

Consequently, we obtain

$$ \partial_{1}\varphi \circ \mathbf{F}_{Q} = \frac{\partial_{1} \widehat{\varphi} \partial_{2} \mathrm{F}_{Q,2}-\partial_{2} \widehat{\varphi} \partial_{1} \mathrm{F}_{Q,2}}{\mathit{J}} \quad\text{ and }\quad \partial_{2}\varphi \circ \mathbf{F}_{Q} = \frac{-\partial_{1} \widehat{\varphi} \partial_{2} \mathrm{F}_{Q,1}+\partial_{2} \widehat{\varphi} \partial_{1} \mathrm{F}_{Q,1}}{\mathit{J}}. $$

For second derivatives, the chain rule yields

$$ \begin{array}{rcl} \left( \begin{array}{c} {\partial_{1}^{2}}\widehat{\varphi} \\ \partial_{1}\partial_{2}\widehat{\varphi} \\ {\partial_{2}^{2}}\widehat{\varphi} \end{array} \right) &=& \left( \begin{array}{ccc} (\partial_{1}\mathrm{F}_{Q,1})^{2} & 2\partial_{1}\mathrm{F}_{Q,1} \partial_{1}\mathrm{F}_{Q,2} & (\partial_{1}\mathrm{F}_{Q,2})^{2} \\ \partial_{1}\mathrm{F}_{Q,1} \partial_{2}\mathrm{F}_{Q,1} & \partial_{1}\mathrm{F}_{Q,1} \partial_{2}\mathrm{F}_{Q,2} + \partial_{2}\mathrm{F}_{Q,1} \partial_{1}\mathrm{F}_{Q,2} & \partial_{1}\mathrm{F}_{Q,2} \partial_{2}\mathrm{F}_{Q,2} \\ (\partial_{2}\mathrm{F}_{Q,1})^{2} & 2\partial_{2}\mathrm{F}_{Q,1} \partial_{2}\mathrm{F}_{Q,2} & (\partial_{2}\mathrm{F}_{Q,2})^{2} \end{array} \right) \left( \begin{array}{c} \partial_{1}^{2}\varphi\circ\mathbf{F}_{Q} \\ \partial_{1}\partial_{2}\varphi\circ\mathbf{F}_{Q} \\ \partial_{2}^{2}\varphi\circ\mathbf{F}_{Q} \end{array} \right) \\ &&+ \left( \begin{array}{cc} {\partial_{1}^{2}}\mathrm{F}_{Q,1} & {\partial_{1}^{2}}\mathrm{F}_{Q,2} \\ \partial_{1}\partial_{2}\mathrm{F}_{Q,1} & \partial_{1}\partial_{2}\mathrm{F}_{Q,2} \\ {\partial_{2}^{2}}\mathrm{F}_{Q,1} & {\partial_{2}^{2}}\mathrm{F}_{Q,2} \end{array} \right) \left( \begin{array}{c} \partial_{1}\varphi\circ\mathbf{F}_{Q} \\ \partial_{2}\varphi\circ\mathbf{F}_{Q} \end{array} \right). \end{array} $$

Thus, having ${\partial _{i}^{2}}\mathrm {F}_{Q,j}=0$ for all i,j ∈{1, 2}, we obtain

$$ \left( \begin{array}{c} \partial_{1}^{2}\varphi\circ\mathbf{F}_{Q} \\ \partial_{1}\partial_{2}\varphi\circ\mathbf{F}_{Q} \\ \partial_{2}^{2}\varphi\circ\mathbf{F}_{Q} \end{array} \right) = \frac{1}{\mathit{J}^{2}}\left( \begin{array}{ccc} (\partial_{2}\mathrm{F}_{Q,2})^{2} & -2\partial_{1}\mathrm{F}_{Q,2} \partial_{2}\mathrm{F}_{Q,2} & (\partial_{1}\mathrm{F}_{Q,2})^{2} \\ -\partial_{2}\mathrm{F}_{Q,1} \partial_{2}\mathrm{F}_{Q,2} & \partial_{1}\mathrm{F}_{Q,1} \partial_{2}\mathrm{F}_{Q,2} + \partial_{2}\mathrm{F}_{Q,1} \partial_{1}\mathrm{F}_{Q,2} & -\partial_{1}\mathrm{F}_{Q,1} \partial_{1}\mathrm{F}_{Q,2} \\ (\partial_{2}\mathrm{F}_{Q,1})^{2} & -2\partial_{1}\mathrm{F}_{Q,1} \partial_{2}\mathrm{F}_{Q,1} & (\partial_{1}\mathrm{F}_{Q,1})^{2} \end{array} \right) \left( \begin{array}{c} {\partial_{1}^{2}}\widehat{\varphi} \\ \partial_{1}\partial_{2}\widehat{\varphi} - X \\ {\partial_{2}^{2}}\widehat{\varphi} \end{array} \right), $$

where

$$ X = \frac{\partial_{1} \widehat{\varphi} \partial_{2} \mathrm{F}_{Q,2}-\partial_{2} \widehat{\varphi} \partial_{1} \mathrm{F}_{Q,2}}{\mathit{J}} \partial_{1}\partial_{2}\mathrm{F}_{Q,1} + \frac{-\partial_{1} \widehat{\varphi} \partial_{2} \mathrm{F}_{Q,1}+\partial_{2} \widehat{\varphi} \partial_{1} \mathrm{F}_{Q,1}}{\mathit{J}} \partial_{1}\partial_{2}\mathrm{F}_{Q,2}. $$

Appendix B: Precomputations for basis functions

We recall the notation presented in Section ?? and introduce additional precomputable terms. Let

$$ \mathbf{t}^{(k)} = (t_{1}^{(k)},t_{2}^{(k)})^{T} = {\mathbf{v}}^{(k+1)} - {\mathbf{v}}^{(k)} $$

be the vector corresponding to the edge ε^(k) and let

$$ (s^{(k)}_{1},s^{(k)}_{2})^{T} = (-t_{2}^{(k)},t_{1}^{(k)})^{T}, $$

then we have

$$ \mathbf{n}_{\varepsilon^{(k)}} = (n^{(k)}_{1},n^{(k)}_{2})^{T} = \frac{1}{\|\mathbf{t}^{(k)}\|}(s^{(k)}_{1},s^{(k)}_{2})^{T}. $$

Let moreover

$$\renewcommand{\arraystretch}{1.5} \begin{array}{lll} a^{(k)}&=&\det (\mathbf{t}^{(k-1)}, \mathbf{t}^{(k)} ), \\ b^{(k)} &=& \mathbf{t}^{(k-1)} \cdot \mathbf{t}^{(k)}, \\ \mathbf{q}^{(k)} &=& (q_{1}^{(k)},q_{2}^{(k)})^{T} = \mathbf{t}^{(k-1)} + \mathbf{t}^{(k+1)}, \\ T^{(k)}_{i,j} &=& t^{(k)}_{i} t^{(k)}_{j}, \\ Q^{(k)}_{i,j} &=& t^{(k-1)}_{i} t^{(k)}_{j} + t^{(k-1)}_{j} t^{(k)}_{i}, \\ S^{(k)}_{i,j} &=& s^{(k)}_{i} t^{(k)}_{j} + s^{(k)}_{j} t^{(k)}_{i}, \end{array} $$

for i,j ∈{1, 2} and k ∈{1, 2, 3, 4}. Here k is considered modulo 4.

2.1 B.1 Precomputations for degree p = 5

For p = 5 we define

and

We moreover define

and

$$ \begin{array}{lcl} \mathbf{E}_{T}^{(k)} &=& -\frac{8 a^{(k+1)}}{5\| \mathbf{t}^{(k)} \|} \mathbf{K}_{1} - \frac{8a^{(k)}}{5\| \mathbf{t}^{(k)} \|} \mathbf{K}_{2}, \\ \mathbf{E}_{L}^{(k)} &=& \frac{8 a^{(k)}}{5\| \mathbf{t}^{(k)} \|} \mathbf{K}_{1}^{T} + \frac{8 a^{(k+1)}}{5\| \mathbf{t}^{(k)} \|} \mathbf{K}_{2}^{T}, \end{array} $$

$$ \begin{array}{lcl} \mathbf{X}_{T}^{(k)} &=& \frac{3 b^{(k+1)}}{\| \mathbf{t}^{(k)} \|^{2}} \mathbf{K}_{1} - \frac{3 b^{(k)}}{\| \mathbf{t}^{(k)} \|^{2}} \mathbf{K}_{2}, \\ \mathbf{X}_{L}^{(k)} &=& \frac{3 b^{(k)}}{\| \mathbf{t}^{(k)} \|^{2}} \mathbf{K}_{1}^{T} - \frac{3 b^{(k+1)}}{\| \mathbf{t}^{(k)} \|^{2}} \mathbf{K}_{2}^{T}. \end{array} $$

In addition, we define the correction terms

$$ \begin{array}{lcl} \mathbf{Y}_{T,i}^{(k)} &=& \left( \frac{-12 t^{(k)}_{i} b^{(k+1)}-5 s^{(k)}_{i} a^{(k+1)}}{10\| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{1} + \left( \frac{12 t^{(k)}_{i} b^{(k)}-5 s^{(k)}_{i} a^{(k)}}{10\| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{2}, \\ \mathbf{Y}_{L,i}^{(k)} &=& \left( \frac{12 t^{(k)}_{i} b^{(k)}-5 s^{(k)}_{i} a^{(k)}}{10\| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{1}^{T} + \left( \frac{-12 t^{(k)}_{i} b^{(k+1)}-5 s^{(k)}_{i} a^{(k+1)}}{10\| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{2}^{T}, \\ \mathbf{Z}_{T,(i,j)}^{(k)} &=& \left( \frac{3 T^{(k)}_{i,j} b^{(k+1)} + S^{(k)}_{i,j} a^{(k+1)}}{20\| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{1} + \left( \frac{-3 T^{(k)}_{i,j} b^{(k)} + S^{(k)}_{i,j} a^{(k)}}{20\| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{2}, \\ \mathbf{Z}_{L,(i,j)}^{(k)} &=& \left( \frac{3 T^{(k)}_{i,j} b^{(k)} - S^{(k)}_{i,j} a^{(k)}}{20\| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{1}^{T} + \left( \frac{-3 T^{(k)}_{i,j} b^{(k+1)} - S^{(k)}_{i,j} a^{(k+1)}}{20\| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{2}^{T}. \end{array} $$

(B.1)

We then have

$$\begin{array}{lcll} \mathbf{V}_{0}^{(k)} & = & \mathbf{X} + \mathbf{X}_{T}^{(k-1)} + \mathbf{X}_{L}^{(k)}, & \\ \mathbf{V}_{i}^{(k)} & = & \mathbf{Y}_{i}^{(k)} + \mathbf{Y}_{T,i}^{(k-1)} + \mathbf{Y}_{L,i}^{(k)}, \quad&\text{for }i\in\{1,2\},\\ \mathbf{V}_{i+j+1}^{(k)} & = & (2-{\delta_{i}^{j}})\left( \mathbf{Z}_{(i,j)}^{(k)} + \mathbf{Z}_{T,(i,j)}^{(k-1)} + \mathbf{Z}_{L,(i,j)}^{(k)}\right), \quad&\text{for }(i,j)\!\in\!\{(1,1),(1,2),(2,2)\}. \end{array} $$

(B.2)

The functions derived from the coefficient matrices X, $\mathbf {Y}_{i}^{(k)}$ and $\mathbf {Z}_{(i,j)}^{(k)}$, i.e., $\hat {\mathbf {b}}^{p}(\xi _{1})^{T} R^{(k)}\left (\mathbf {X}\right )\hat {\mathbf {b}}^{p}(\xi _{2})$, are constructed in such a way that they satisfy the C² interpolation conditions at the vertex v^k and have vanishing derivatives up to second order at all others. Moreover, they have vanishing function values and gradients along the edges ε^k+ 1 and ε^k+ 2. However, their normal derivatives along the edges ε^k and ε^k− 1 are (in general) not polynomials of degree four, i.e., they do not satisfy the condition $(\partial _{\mathbf {n}_{\varepsilon ^{(\ell )}}} \varphi \circ \mathbf {F}_{Q})|_{\hat {\varepsilon }^{(\ell )}} \in \mathbb {P}_{4}$ in Eq. ?? for ℓ ∈{k − 1,k}, and their normal derivatives do not vanish at the edge midpoints, i.e., $\partial _{\mathbf {n}_{\varepsilon ^{(\ell )}}} \varphi (\mathbf {m}_{\varepsilon ^{(\ell )}}) \neq 0$ for ℓ ∈{k − 1,k}. The correction terms in Eq. B.1 are defined in such a way that both conditions are satisfied for the functions ${\beta }_{\mathbf {v},i}^{(k)}$, with i ∈{0, 1, 2, 3, 4, 5}, defined in Eq. ??. The functions for degrees p = 4 and p = 3 are constructed analogously.

2.2 B.2 Precomputations for degree p = 4

For macro-element constructions of degree p = 3 and p = 4 the involved matrices are different from those for degree p = 5. For p = 4 we have

and

as well as

$$ \begin{array}{lcllcl} \mathbf{E}_{T}^{(k)} &=& -\frac{a^{(k+1)}}{2 \| \mathbf{t}^{(k)} \|} \mathbf{K}_{1} -\frac{a^{(k)}}{2 \| \mathbf{t}^{(k)} \|} \mathbf{K}_{2}, \qquad& \mathbf{X}_{T}^{(k)} &=& \frac{b^{(k+1)}}{ \| \mathbf{t}^{(k)} \|^{2}} \mathbf{K}_{1} - \frac{b^{(k)}}{ \| \mathbf{t}^{(k)} \|^{2}} \mathbf{K}_{2}, \\ \mathbf{E}_{L}^{(k)} &=& \frac{a^{(k)}}{ 2 \| \mathbf{t}^{(k)} \|} \mathbf{K}_{1}^{T} + \frac{a^{(k+1)}}{2 \| \mathbf{t}^{(k)} \|} \mathbf{K}_{2}^{T}, & \mathbf{X}_{L}^{(k)} &=& \frac{b^{(k)}}{ \| \mathbf{t}^{(k)} \|^{2}} \mathbf{K}_{1}^{T} - \frac{b^{(k+1)}}{ \| \mathbf{t}^{(k)} \|^{2}} \mathbf{K}_{2}^{T}, \end{array} $$

and the correction terms

$$ \begin{array}{lcl} \mathbf{Y}_{T,i}^{(k)} &=& \left( \frac{-3 t^{(k)}_{i} b^{(k+1)}- s^{(k)}_{i} a^{(k+1)}}{8\| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{1} + \left( \frac{3 t^{(k)}_{i} b^{(k)}- s^{(k)}_{i} a^{(k)}}{8\| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{2}, \\ \mathbf{Y}_{L,i}^{(k)} &=& \left( \frac{3 t^{(k)}_{i} b^{(k)}- s^{(k)}_{i} a^{(k)}}{8\| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{1}^{T} + \left( \frac{-3 t^{(k)}_{i} b^{(k+1)}- s^{(k)}_{i} a^{(k+1)}}{8\| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{2}^{T}, \\ \mathbf{Z}_{T,(i,j)}^{(k)} &=& \left( \frac{4 T^{(k)}_{i,j} b^{(k+1)} + S^{(k)}_{i,j} a^{(k+1)}}{96 \| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{1} + \left( \frac{-4 T^{(k)}_{i,j} b^{(k)} + S^{(k)}_{i,j} a^{(k)}}{96 \| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{2}, \\ \mathbf{Z}_{L,(i,j)}^{(k)} &=& \left( \frac{4 T^{(k)}_{i,j} b^{(k)} - S^{(k)}_{i,j} a^{(k)}}{96 \| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{1}^{T} + \left( \frac{-4 T^{(k)}_{i,j} b^{(k+1)} - S^{(k)}_{i,j} a^{(k+1)}}{96 \| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{2}^{T}. \end{array} $$

The matrices $\mathbf {V}_{i}^{(k)}$, for 0 ≤ i ≤ 5, are then defined as in Eq. B.2.

2.3 B.3 Precomputations for degree p = 3

For p = 3 we have

and

where all matrices are of size 8 × 8 and the dots signify that the matrices are completed with zeros. We moreover have

$$ \begin{array}{lcllcl} \mathbf{E}_{T}^{(k)} &=& -\frac{2a^{(k+1)}}{9 \| \mathbf{t}^{(k)} \|} \mathbf{K}_{1} -\frac{2a^{(k)}}{9 \| \mathbf{t}^{(k)} \|} \mathbf{K}_{2}, \qquad& \mathbf{X}_{T}^{(k)} &=& \frac{b^{(k+1)}}{2 \| \mathbf{t}^{(k)} \|^{2}} \mathbf{K}_{1} - \frac{b^{(k)}}{2 \| \mathbf{t}^{(k)} \|^{2}} \mathbf{K}_{2}, \\ \mathbf{E}_{L}^{(k)} &=& \frac{2a^{(k)}}{9 \| \mathbf{t}^{(k)} \|} {\mathbf{K}_{\text{1}}^{\text{T}}} + \frac{2a^{(k+1)}}{9 \| \mathbf{t}^{(k)} \|} {\mathbf{K}_{\text{2}}^{\text{T}}}, & \mathbf{X}_{L}^{(k)} &=& \frac{b^{(k)}}{2 \| \mathbf{t}^{(k)} \|^{2}} {\mathbf{K}_{\text{1}}^{\text{T}}} - \frac{b^{(k+1)}}{2 \| \mathbf{t}^{(k)} \|^{2}} {\mathbf{K}_{\text{2}}^{\text{T}}}, \end{array} $$

and the correction terms

$$ \begin{array}{lcl} \mathbf{Y}_{T,i}^{(k)} &=& \left( \frac{-6 t^{(k)}_{i} b^{(k+1)}- s^{(k)}_{i} a^{(k+1)}}{36 \| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{1} + \left( \frac{6 t^{(k)}_{i} b^{(k)}- s^{(k)}_{i} a^{(k)}}{36 \| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{2}, \\ \mathbf{Y}_{L,i}^{(k)} &=& \left( \frac{6 t^{(k)}_{i} b^{(k)}- s^{(k)}_{i} a^{(k)}}{36 \| \mathbf{t}^{(k)} \|^{2}} \right) {\mathbf{K}_{\text{1}}^{\text{T}}} + \left( \frac{-6 t^{(k)}_{i} b^{(k+1)}- s^{(k)}_{i} a^{(k+1)}}{36 \| \mathbf{t}^{(k)} \|^{2}} \right) {\mathbf{K}_{\text{2}}^{\text{T}}}, \\ \mathbf{Z}_{T,(i,j)}^{(k)} &=& \left( \frac{8 T^{(k)}_{i,j} b^{(k+1)} + S^{(k)}_{i,j} a^{(k+1)}}{432 \| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{1} + \left( \frac{-8 T^{(k)}_{i,j} b^{(k)} + S^{(k)}_{i,j} a^{(k)}}{432 \| \mathbf{t}^{(k)} \|^{2}} \right) \mathbf{K}_{2}, \\ \mathbf{Z}_{L,(i,j)}^{(k)} &=& \left( \frac{8 T^{(k)}_{i,j} b^{(k)} - S^{(k)}_{i,j} a^{(k)}}{432 \| \mathbf{t}^{(k)} \|^{2}} \right) {\mathbf{K}_{\text{1}}^{\text{T}}} + \left( \frac{-8 T^{(k)}_{i,j} b^{(k+1)} - S^{(k)}_{i,j} a^{(k+1)}}{432 \| \mathbf{t}^{(k)} \|^{2}} \right) {\mathbf{K}_{\text{2}}^{\text{T}}}. \end{array} $$

The matrices $\mathbf {V}_{i}^{(k)}$, for 0 ≤ i ≤ 5, are again defined as in Eq. B.2.