Synchronization of unified chaotic system via adaptive wavelet cerebellar model articulation controller | Neural Computing and Applications Skip to main content
Springer Nature Link
Log in

Synchronization of unified chaotic system via adaptive wavelet cerebellar model articulation controller

  • Published:
Neural Computing and Applications Aims and scope Submit manuscript

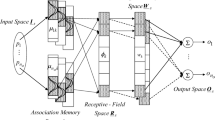
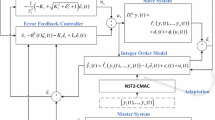
Abstract

This study aims to propose a more efficient control algorithm for the chaotic system synchronization. In this study, a novel wavelet cerebellar model articulation controller (WCMAC) is proposed, which incorporates the wavelet decomposition property with a cerebellar model articulation controller (CMAC). This WCMAC is a generalization network; in some special cases, it can be reduced to a wavelet neural network, a neural network and a conventional CMAC. Then, an adaptive wavelet cerebellar model articulation control system (AWCCS) is proposed to synchronize a unified chaotic system. In this AWCCS, WCMAC is the main controller utilized to mimic a perfect controller and the parameters of WCMAC are online adjusted by the derived adaptive laws; and a compensation controller is designed to dispel the residual of the approximation error for achieving \( H^{\infty } \) robust performance. The derived AWCCS is then applied to the chaotic system synchronization control. Finally, the effectiveness of the proposed control system is demonstrated through simulation results.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  1. Ott E, Grebogi C, Yorke JA (1990) Controlling chaos. Phys Rev Lett 64:1196–1199

    Article  MathSciNet  MATH  Google Scholar 

  2. Chen G (1999) Controlling chaos and bifurcations in engineering systems. CRC Press, Boca Raton

    Google Scholar 

  3. Jiang ZP (2002) Advanced feedback control of the chaotic Duffing equation. IEEE Trans Circuits Syst I 49:244–249

    Article  Google Scholar 

  4. Wai RJ, Lin YW, Yang HC (2011) Experimental verification of total sliding-mode control for Chua’s chaotic circuit. IET Circuits Devices Syst 5:451–461

    Article  Google Scholar 

  5. Hugues-Salas O, Shore KA, Banks SP (2008) Stabilisation of chaotic dynamics in semiconductor lasers with optical feedback using optimal control. IET Optoelectron 2:231–240

    Article  Google Scholar 

  6. Andreyev YV, Dmitriev AS, Efremova EV, Anagnostopoulos AN (2003) Chaotic signal processing: information aspects. Chaos Solitons Fractals 17:531–544

    Article  MATH  Google Scholar 

  7. Terry JR, VanWiggeren GD (2001) Chaotic communication using generalized synchronization. Chaos Solitons Fractals 12:145–152

    Article  Google Scholar 

  8. Li ZG, Xu DL (2004) A secure communication scheme using projective chaos synchronization. Chaos Solitons Fractals 22:477–481

    Article  MATH  Google Scholar 

  9. Jovic B, Unsworth CP (2010) Fast synchronisation of chaotic maps for secure chaotic communications. Electron Lett 46:49–50

    Article  Google Scholar 

  10. Sheikhan M, Shahnazi R, Garoucy S (2012) Synchronization of general chaotic systems using neural controllers with application to secure communication. Neural Comput Appl. doi:10.1007/s00521-011-0697-0

  11. Loria A, Zavala-Rio A (2007) Adaptive tracking control of chaotic systems with applications to synchronization. IEEE Trans Circuits Syst I 54:2019–2029

    Article  MathSciNet  Google Scholar 

  12. Park JH (2005) Stability criterion for synchronization of linearly coupled unified chaotic systems. Chaos Solitons Fractals 23:1319–1325

    MATH  Google Scholar 

  13. Lin CM, Hsu CF (2003) Neural network hybrid control for antilock braking systems. IEEE Trans Neural Netw 14:351–359

    Article  Google Scholar 

  14. Chen CJ, Liao TL, Yan JJ, Hwang CC (2005) Synchronization of neural networks by decentralized feedback control. Phys Lett A 338:28–35

    Article  Google Scholar 

  15. Hou YY, Liao TL, Yan JJ (2003) Global asymptotic stability for a class of nonlinear neural networks with multiple delays. Nonlinear Anal 53:319–333

    Article  MathSciNet  Google Scholar 

  16. Wai RJ, Duan RY, Lee JD, Chang HH (2003) Wavelet neural network control for induction motor drive using sliding-mode design technique. IEEE Trans Neural Netw 50:733–748

    Google Scholar 

  17. Hsu CF, Lin CM, Lee TT (2006) Wavelet adaptive backstepping control for a class of nonlinear systems. IEEE Trans Neural Netw 17:1175–1183

    Article  Google Scholar 

  18. Albus JS (1975) A new approach to manipulator control: the cerebellar model articulation controller (CMAC). J Dyn Syst Meas Contr 97:220–227

    Article  MATH  Google Scholar 

  19. Chiang CT, Lin CS (1996) CMAC with general basis functions. Neural Netw 9:1199–1211

    Article  Google Scholar 

  20. Lin CM, Peng YF (2004) Adaptive CMAC-based supervisory control for uncertain nonlinear systems. IEEE Trans Syst Man Cybern B Cybern 34:1248–1260

    Article  Google Scholar 

  21. Peng YF, Lin CM (2007) Intelligent motion control of linear ultrasonic motor with H∞ tracking performance. IET Proc Control Theory Appl 1:9–17

    Article  MathSciNet  Google Scholar 

  22. Almedia PEM, Simoes MG (2003) Parametric CMAC networks fundamentals and applications of a fast convergence neural structure. IEEE Trans Ind Appl 39:1551–1557

    Article  Google Scholar 

  23. Lin CM, Chen LY, Chen CH (2007) RCMAC hybrid control for MIMO uncertain nonlinear systems using sliding-mode technology. IEEE Trans Neural Netw 18:708–720

    Article  Google Scholar 

  24. Lorenz E (1963) Deterministic nonperiodic flows. J Atmos Sci 20:130–141

    Article  Google Scholar 

  25. Chen GR, Ueta T (1999) Yet another chaotic attractor. Int J Bifurcat Chaos 9:146–156

    MathSciNet  Google Scholar 

  26. Lu J, Chen G, Cheng D (2002) Bridge the gap between the Lorenz system and the Chen system. Int J Bifurcat Chaos 12:2917–2926

    Article  MathSciNet  Google Scholar 

  27. Park JH (2005) On synchronization of unified chaotic systems via nonlinear control. Chaos Solitons Fractals 25:699–704

    Article  MATH  Google Scholar 

  28. Ucar A, Lonngren KE, Bai EW (2006) Synchronization of the unified chaotic systems via active control. Chaos Solitons Fractals 27:1292–1297

    Article  MATH  Google Scholar 

  29. Chen BS, Lee CH (1996) H∞ tracking design of uncertain nonlinear SISO systems: adaptive fuzzy approach. IEEE Trans Fuzzy Syst 4:32–43

    Article  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Department of Electrical Engineering, Yuan Ze University, No. 135, Far-East Rd., Chung-Li, Tao-Yuan, Taiwan, 320, Republic of China

    Chih-Min Lin & Rong-Guan Yeh

  2. Chung Shan Institute of Science and Technology, Taoyuan, Taiwan, Republic of China

    Ming-Hung Lin

Authors
  1. Chih-Min Lin
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Ming-Hung Lin
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Rong-Guan Yeh
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Chih-Min Lin.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Lin, CM., Lin, MH. & Yeh, RG. Synchronization of unified chaotic system via adaptive wavelet cerebellar model articulation controller. Neural Comput & Applic 23, 965–973 (2013). https://doi.org/10.1007/s00521-012-1021-3

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00521-012-1021-3

Keywords

Search

Navigation