The relationship among soft sets, soft rough sets and topologies | Soft Computing Skip to main content
Springer Nature Link
Log in

The relationship among soft sets, soft rough sets and topologies

  • Foundations
  • Published:
Soft Computing Aims and scope Submit manuscript

Abstract

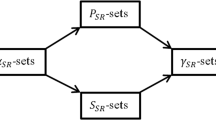
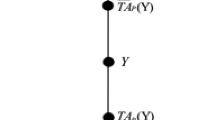
Molodtsov’s soft set theory is a newly emerging tool to deal with uncertain problems. Based on the novel granulation structures called soft approximation spaces, Feng et al. initiated soft rough approximations and soft rough sets. Feng’s soft rough sets can be seen as a generalized rough set model based on soft sets, which could provide better approximations than Pawlak’s rough sets in some cases. This paper is devoted to establishing the relationship among soft sets, soft rough sets and topologies. We introduce the concept of topological soft sets by combining soft sets with topologies and give their properties. New types of soft sets such as keeping intersection soft sets and keeping union soft sets are defined and supported by some illustrative examples. We describe the relationship between rough sets and soft rough sets. We obtain the structure of soft rough sets and the topological structure of soft sets, and reveal that every topological space on the initial universe is a soft approximating space.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

Explore related subjects

Discover the latest articles, news and stories from top researchers in related subjects.

References

  • Aktas H N. Cağman, (2007) soft sets and soft groups. Inf Sci 177:2726–2735

  • Császár A (2002) Generalized topology, generalized continuity. Acta Mathematica Hungarica 96:351–357

    Article  MATH  MathSciNet  Google Scholar 

  • Császár A (1997) Generalized open sets. Acta Mathematica Hungarica 75:65–87

    Article  MATH  MathSciNet  Google Scholar 

  • Feng F, Li C, Davvaz B (2010) Soft sets combined with fuzzy sets and rough sets: a tentative approach. Soft Comput 14:899–911

    Article  MATH  Google Scholar 

  • Feng F, Liu X, Leoreanu-Fotea V, Jun Y (2011) Soft sets and soft rough sets. Inf Sci 181:1125–1137

    Article  MATH  MathSciNet  Google Scholar 

  • Grzymala-Busse JW (2011) Mining incomplete data—a rough set approach, rough sets and knowledge technology. Lect Notes Comput Sci 6954:1–7

    Article  Google Scholar 

  • Ge X, Li Z, Ge Y (2011) Topological spaces and soft sets. J Comput Anal Appl 13:881–885

    MATH  MathSciNet  Google Scholar 

  • Jiang Y, Tang Y, Chen Q, Wang J, Tang S (2010) Extending soft sets with description logics. Comput Math Appl 59:2087–2096

    Google Scholar 

  • Kong Z, Gao L, Wang L, Li S (2008) The normal parameter reduction of soft sets and its algorithm. Comput Math Appl 56:3029–3037

    Google Scholar 

  • Maji PK, Biswas R, Roy AR (2003) Soft set theory. Comput Math Appl 45:555–562

    Google Scholar 

  • Maji PK, Biswas R, Roy AR (2001) Fuzzy soft sets. J Fuzzy Math 9:589–602

    MATH  MathSciNet  Google Scholar 

  • Majumdar P, Samanta SK (2008) Similarity measure of soft sets. New Math Nat Comput 4(1):1–12

    Article  MATH  MathSciNet  Google Scholar 

  • Maji PK, Roy AR, Biswas R (2002) An application of soft sets in a decision making problem. Comput Math Appl 44:1077–1083

    Article  MATH  MathSciNet  Google Scholar 

  • Molodtsov D (1999) Soft set theory-first result. Comput Math Appl 37:19–31

    Article  MATH  MathSciNet  Google Scholar 

  • Pawlak Z (1991) Rough sets: theoretical aspects of reasoning about data. Kluwer Academic Publishers, Boston

    Book  MATH  Google Scholar 

  • Pei D, Miao D (2005) From soft sets to information systems. In: Hu X, Liu Q, Skowron A, Lin TY, Yager RR, Zhang B (eds) Proceedings of Granular Computing, IEEE, vol 2, pp 617–621

  • Roy AR, Maji PK (2007) A fuzzy soft set theoretic approach to decision making problems. J Comput Appl Math 203:412–418

    Article  MATH  Google Scholar 

  • Shabir M, Naz M (2011) On soft topological spaces. Comput Math Appl 561:1786–1799

    Article  MathSciNet  Google Scholar 

  • Skowron A, Stepaniuk J (1996) Tolerance approximation spaces. Fundamenta Informaticae 27:245–253

    MATH  MathSciNet  Google Scholar 

  • Slowinski R, Vanderpooten D (1995) Similarity relation as a basis for rough approximations. ICS Research Report 53:249–250

  • Slowinski R, Vanderpooten D (2000) A generalized definition of rough approximations based on similarity. IEEE Trans Data Knowl Eng 12(2):331–336

    Article  Google Scholar 

  • Yao YY (1996) Two views of the theory of rough sets in finite universes. Int J Approx Reason 15:291–317

    Article  MATH  Google Scholar 

  • Yao YY, Lin TY (1996) Generalization of rough sets using modal logics. Intell Autom Soft Comput 2(2):103–120

    Article  Google Scholar 

  • Zadeh LA (1965) Fuzzy sets. Inf Control 8:338–353

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. College of Science, Guangxi University for Nationalities, Nanning, Guangxi, 530006, People’s Republic of China

    Zhaowen Li

  2. College of Mathematics and Information Science, Guangxi University, Nanning, Guangxi, 530004, People’s Republic of China

    Tusheng Xie

Authors
  1. Zhaowen Li
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Tusheng Xie
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Zhaowen Li.

Additional information

Communicated by L. G. Lacasa.

This work is supported by the National Natural Science Foundation of China (No. 11061004, 71140004), the Natural Science Foundation of Guangxi (No. 2013GXNSFBA019016) and Guangxi University Science and Technology Research Project (No. 2013ZD020, 2013ZD061).

Rights and permissions

Reprints and permissions

About this article

Cite this article

Li, Z., Xie, T. The relationship among soft sets, soft rough sets and topologies. Soft Comput 18, 717–728 (2014). https://doi.org/10.1007/s00500-013-1108-5

Download citation

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00500-013-1108-5

Keywords

Search

Navigation