Equilibrium unzipping at finite temperature | Archive of Applied Mechanics Skip to main content
Springer Nature Link
Log in

Equilibrium unzipping at finite temperature

  • SPECIAL
  • Published:
Archive of Applied Mechanics Aims and scope Submit manuscript

Abstract

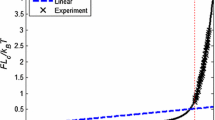
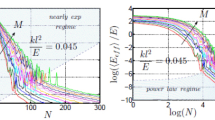
We study thermally activated unzipping, which is modeled as a debonding process. The system is modeled as a parallel bundle of elastically interacting breakable units loaded through a series spring. Using equilibrium statistical mechanics, we compute the reversible response of this mechanical system under quasi-static driving. Depending on the stiffness of the series spring, the system exhibits either ductile behavior, characterized by noncooperative debonding, or brittle behavior, with a highly correlated detachment of the whole bundle. We show that the ductile to brittle transition is of the second order and that it can also be controlled by temperature.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Fig. 1
Fig. 2
Fig. 3
Fig. 4
Fig. 5
Fig. 6
Fig. 7
Fig. 8
Fig. 9
Fig. 10
Fig. 11

Similar content being viewed by others

References

  1. Kanninen, M., Popelar, C.: Advanced Fracture Mechanics. Oxford University Press, Oxford (1985)

    MATH  Google Scholar 

  2. Brenner, S.S.: Mechanical behavior of sapphire whiskers at elevated temperatures. J. Appl. Phys. 33, 33 (1962)

    Article  Google Scholar 

  3. Selinger, R.L.B., Wang, Z.-G., Gelbart, W.M.: Statistical-thermodynamic approach to fracture. J. Chem. Phys. 95, 9128 (1991)

    Article  Google Scholar 

  4. Cook, R.F., Liniger, E.G.: Kinetics of indentation cracking in glass. J. Am. Ceram. Soc. 76, 1096 (1993)

    Article  Google Scholar 

  5. Petrov, V.A., Orlov, A.N.: Statistical kinetics of thermally activated fracture. Int. J. Fract. 12, 231 (1976)

    Article  Google Scholar 

  6. Xing, X.-S.: Nonequilibrium statistical theory of thermally activated delayed fracture. Eng. Fract. Mech. 38, 1 (1991)

    Article  Google Scholar 

  7. Berdichevsky, V., Le, K.C.: On the microcrack nucleation in brittle solids. Int. J. Fract. 133, L47 (2005)

    Article  MATH  Google Scholar 

  8. K. K. a. A. S. Krausz, Fracture Kinetics of Crack Growth, 1st ed., Mechanical Behavior of Materials 1 (Springer Netherlands, 1988)

  9. Pomeau, Y.: Brisure spontanée de cristaux bidimensionnels courbés. C. R. Acac. Sci. Paris Serie II , 553 (1992)

  10. Ciliberto, S., Guarino, A., Scorretti, R.: The effect of disorder on the fracture nucleation process. Phys. D: Nonlinear Phenom. 158, 83 (2001)

    Article  MATH  Google Scholar 

  11. Politi, A., Ciliberto, S., Scorretti, R.: Failure time in the fiber-bundle model with thermal noise and disorder. Phys. Rev. E 66, 026107 (2002)

    Article  Google Scholar 

  12. Bell, G.: Models for the specific adhesion of cells to cells. Science 200, 618 (1978)

    Article  Google Scholar 

  13. Schwarz, U.S., Safran, S.A.: Physics of adherent cells. Rev. Mod. Phys. 85, 1327 (2013)

    Article  Google Scholar 

  14. Evans, E., Ritchie, K.: Dynamic strength of molecular adhesion bonds. Biophys. J. 72, 1541 (1997)

    Article  Google Scholar 

  15. Chakrabarti, B., Nelson, D.R.: Shear unzipping of DNA. J. Phys. Chem. B 113, 3831 (2009)

    Article  Google Scholar 

  16. Hyeon, C., Thirumalai, D.D.: Minimal models for the structure and dynamics of nucleic acids. Mol. Model. At. Scale: Methods Appl. Quant. Biol. 141 (2014)

  17. Mishra, R.K., Modi, T., Giri, D., Kumar, S.: On the rupture of DNA molecule. J. Chem. Phys. 142, 174910 (2015)

    Article  Google Scholar 

  18. Bergues-Pupo, A.E., Bergues, J.M., Falo, F., Fiasconaro, A.: Thermal and inertial resonances in DNA unzipping. Eur. Phys. J. E 38, 41 (2015)

    Article  Google Scholar 

  19. Strunz, T., Oroszlan, K., Schäfer, R., Güntherodt, H.-J.: Dynamic force spectroscopy of single DNA molecules. Proc. Natl. Acad. Sci. 96, 11277 (1999)

    Article  Google Scholar 

  20. Helenius, J., Heisenberg, C.-P., Gaub, H.E., Muller, D.J.: Single-cell force spectroscopy. J. Cell Sci. 121, 1785 (2008)

    Article  Google Scholar 

  21. Friedrichs, J., Helenius, J., Muller, D.J.: Quantifying cellular adhesion to extracellular matrix components by single-cell force spectroscopy. Nat. Protoc. 5, 1353 (2010)

    Article  Google Scholar 

  22. Gonzalez-Rodriguez, D., Bonnemay, L., Elgeti, J., Dufour, S., Cuvelier, D., Brochard- Wyart, F.: Detachment and fracture of cellular aggregates. Soft Matter 9, 2282 (2013)

    Article  Google Scholar 

  23. Hogan, B., Babataheri, A., Hwang, Y., Barakat, A.I., Husson, J.: Characterizing cell adhesion by using micropipette aspiration. Biophys. J. 109, 209 (2015)

    Article  Google Scholar 

  24. Selinger, R.L.B., Wang, Z.-G., Gelbart, W.M., Ben-Shaul, A.: Statistical-thermodynamic approach to fracture. Phys. Rev. A 43, 4396 (1991)

    Article  Google Scholar 

  25. Roux, S.: Thermally activated breakdown in the fiber-bundle model. Phys. Rev. E 62, 6164 (2000)

    Article  Google Scholar 

  26. Scorretti, R., Ciliberto, S., Guarino, A.: Disorder enhances the effects of thermal noise in the fiber bundle model. EPL Europhys. Lett. 55, 626 (2001)

    Article  Google Scholar 

  27. Alava, M.J., Nukala, P.K.V.V., Zapperi, S.: Statistical models of fracture. Adv. Phys. 55, 349 (2006)

    Article  Google Scholar 

  28. Virgilii, A., Petri, A., Salinas, S.R.: A thermodynamical fibre bundle model for the fracture of disordered materials. J. Stat. Mech. Theory Exp. 2007, P04009 (2007)

    Article  MathSciNet  Google Scholar 

  29. Yoshioka, N., Kun, F., Ito, N.: Kinetic Monte Carlo algorithm for thermally induced breakdown of fiber bundles. Phys. Rev. E 91, 033305 (2015)

    Article  Google Scholar 

  30. Erdmann, T., Schwarz, U.S.: Stability of adhesion clusters under constant force. Phys. Rev. Lett. 92, 108102 (2004)

    Article  Google Scholar 

  31. Peyrard, M., Bishop, A.R.: Statistical mechanics of a nonlinear model for DNA denaturation. Phys. Rev. Lett. 62, 2755 (1989)

    Article  Google Scholar 

  32. Manghi, M., Destainville, N.: Physics of base-pairing dynamics in DNA. Phys. Rep. 631, 1 (2016)

    Article  MathSciNet  Google Scholar 

  33. Vologodskii, A., Frank-Kamenetskii, M.D.: DNA melting and energetics of the double helix. Phys. Life Rev. (2017)

  34. Erdmann, T., Albert, P.J., Schwarz, U.S.: Stochastic dynamics of small ensembles of non-processive molecular motors: the parallel cluster model. J. Chem. Phys. 139, 175104 (2013)

    Article  Google Scholar 

  35. Caruel, M., Truskinovsky, L.: Physics of muscle contraction. Rep. Prog. Phys. 81, 036602 (2018)

    Article  MathSciNet  Google Scholar 

  36. Seifert, U.: Rupture of multiple parallel molecular bonds under dynamic loading. Phys. Rev. Lett. 84, 2750 (2000)

    Article  Google Scholar 

  37. Erdmann, T., Schwarz, U.: Impact of receptor-ligand distance on adhesion cluster stability. Eur. Phys. J. E 22, 123 (2007)

    Article  Google Scholar 

  38. Erdmann, T., Schwarz, U.S.: Bistability of cell-matrix adhesions resulting from nonlinear receptor-ligand dynamics. Biophys. J. 91, L60 (2006)

    Article  Google Scholar 

  39. Huxley, A.F., Simmons, R.M.: Proposed mechanism of force generation in striated muscle. Nature 233, 533 (1971)

    Article  Google Scholar 

  40. Seifert, U.: Dynamic strength of adhesion molecules: role of rebinding and self-consistent rates. EPL Europhys. Lett. 58, 792 (2002)

    Article  Google Scholar 

  41. Kramers, H.: Brownian motion in a field of force and the diffusion model of chemical reactions. Physica 7, 284 (1940)

    Article  MathSciNet  MATH  Google Scholar 

  42. Hänggi, P., Talkner, P., Borkovec, M.: Reaction-rate theory: fifty years after Kramers. Rev. Mod. Phys. 62, 251 (1990)

    Article  MathSciNet  Google Scholar 

  43. Pradhan, S., Hansen, A., Chakrabarti, B.K.: Failure processes in elastic fiber bundles. Rev. Mod. Phys. 82, 499 (2010)

    Article  Google Scholar 

  44. Hansen, A., Hemmer, P., Pradhan, S.: The Fiber Bundle Model: Modeling Failure in Materials, Statistical Physics of Fracture and Breakdown. Wiley, New Jersey (2015)

    Book  Google Scholar 

  45. Batra, R.: Elements of Continuum Mechanics, AIAA education series (American Institute of Aeronautics & Astronautics, 2006)

  46. Kreuzer, H.J., Payne, S.H.: Stretching a macromolecule in an atomic force microscope: statistical mechanical analysis. Phys. Rev. E 63, 021906 (2001)

    Article  Google Scholar 

  47. Caruel, M., Truskinovsky, L.: Bi-stability resistant to fluctuations. J. Mech. Phys. Solids 109, 117 (2017)

    Article  MathSciNet  Google Scholar 

  48. Fuhrmann, A., Engler, A.J.: The cytoskeleton regulates cell attachment strength. Biophys. J. 109, 57 (2015)

    Article  Google Scholar 

  49. Discher, D.E., Janmey, P., Wang, Y.-L.: Tissue cells feel and respond to the stiffness of their substrate. Science 310, 1139 (2005)

    Article  Google Scholar 

  50. Yeung, T., Georges, P.C., Flanagan, L.A., Marg, B., Ortiz, M., Funaki, M., Zahir, N., Ming, W., Weaver, V., Janmey, P.A.: Effects of substrate stiffness on cell morphology, cytoskeletal structure, and adhesion. Cell Motil. 60, 24 (2004)

    Article  Google Scholar 

  51. Jülicher, F., Prost, J.: Cooperative molecular motors. Phys. Rev. Lett. 75, 2618 (1995)

    Article  Google Scholar 

  52. Delaplace, A., Roux, S., Jaudier Cabot, G.P.: Damage cascade in a softening interface. Int. J. Solids Struct. 36, 1403 (1999)

    Article  MathSciNet  MATH  Google Scholar 

  53. Campa, A., Dauxois, T., Ruffo, S.: Statistical mechanics and dynamics of solvable models with long-range interactions. Phys. Rep. 480, 57 (2009)

    Article  MathSciNet  Google Scholar 

  54. Caruel, M., Truskinovsky, L.: Statistical mechanics of the Huxley-Simmons model. Phys. Rev. E 93, 062407 (2016)

    Article  MathSciNet  Google Scholar 

  55. Balian, R.: From microphysics to macrophysics: methods and applications of statistical physics, theoretical and mathematical physics. Springer, Berlin (2007)

    MATH  Google Scholar 

  56. Puglisi, G., Truskinovsky, L.: Cohesion-decohesion asymmetry in geckos. Phys. Rev. E 87, 032714 (2013)

    Article  Google Scholar 

  57. Sheshka, R., Recho, P., Truskinovsky, L.: Rigidity generation by nonthermal fluctuations. Phys. Rev. E 93, 052604 (2016)

    Article  Google Scholar 

Download references

Acknowledgements

The authors thank R. Garcia-Garcia for helpful discussions. H.B.R. was supported by a Ph.D. fellowship from Ecole Polytechnique; L. T. was supported by the French Government under the Grants ANR-10-IDEX-0 0 01-02 PSL and ANR-17-CE08-0 047-02.

Author information

Authors and Affiliations

  1. LMS, CNRS-UMR 7649, Ecole Polytechnique, Université Paris-Saclay, 91128, Palaiseau, France

    H. Borja da Rocha

  2. PMMH, CNRS - UMR 7636 PSL-ESPCI, 10 Rue Vauquelin, 75005, Paris, France

    H. Borja da Rocha & L. Truskinovsky

Authors
  1. H. Borja da Rocha
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. L. Truskinovsky
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to H. Borja da Rocha.

Additional information

Publisher's Note

Springer Nature remains neutral with regard to jurisdictional claims in published maps and institutional affiliations.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

da Rocha, H.B., Truskinovsky, L. Equilibrium unzipping at finite temperature. Arch Appl Mech 89, 535–544 (2019). https://doi.org/10.1007/s00419-018-1485-4

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00419-018-1485-4

Keywords

Search

Navigation