The Cycle Spectrum of Claw-free Hamiltonian Graphs | Graphs and Combinatorics Skip to main content
Springer Nature Link
Log in

The Cycle Spectrum of Claw-free Hamiltonian Graphs

  • Original Paper
  • Published:
Graphs and Combinatorics Aims and scope Submit manuscript

Abstract

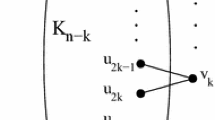
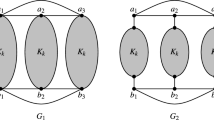
If \(G\) is a claw-free Hamiltonian graph of order \(n\) and maximum degree \(\Delta \) with \(\Delta \ge 24\), then \(G\) has cycles of at least \(\min \left\{ n,\left\lceil \frac{3}{2}\Delta \right\rceil \right\} -2\) many different lengths.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Broersma, H.J., Ryjáček, Z., Schiermeyer, I.: Dirac’s minimum degree condition restricted to claws. Discret. Math. 167–168, 155–166 (1997)

    Article  Google Scholar 

  2. Chen, B., Zhang, S., Qiao, S.: Hamilton cycles in claw-heavy graphs. Discret. Math. 309, 2015–2019 (2009)

    Article  MATH  MathSciNet  Google Scholar 

  3. Chvátal, V., Erdős, P.: A note on Hamiltonian circuits. Discret. Math. 2, 111–113 (1972)

    Article  MATH  Google Scholar 

  4. Faudree, R., Flandrin, E., Ryjáček, Z.: Claw-free graphs—a survey. Discret. Math. 164, 87–147 (1997)

    Article  MATH  Google Scholar 

  5. Ferrara, M., Jacobson, M.S., Harris, A.: Cycle lengths in Hamiltonian graphs with a pair of vertices having large degree sum. Graphs Comb. 26, 215–223 (2010)

    Article  MATH  MathSciNet  Google Scholar 

  6. Gould, R., Pfender, F.: Pancyclicity in claw-free graphs. Discret. Math. 256, 151–160 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  7. Hakimi, S.L., Schmeichel, E.F.: A cycle structure theorem for Hamiltonian graphs. J. Comb. Theory Ser. B 45, 99–107 (1988)

    Article  MATH  MathSciNet  Google Scholar 

  8. Hao, L.: Generalizations of Dirac’s theorem in Hamiltonian graph theory—a survey. Discret. Math. 313, 2034–2053 (2013)

    Article  MATH  Google Scholar 

  9. Kouider, M., Marczyk, A.: On pancyclism in Hamiltonian graphs. Discret. Math. 251, 119–127 (2002)

    Article  MATH  MathSciNet  Google Scholar 

  10. Marczyk, A., Woźniak, M.: Cycles in Hamiltonian graphs of prescribed maximum degree. Discret. Math. 266, 321–326 (2003)

    Article  MATH  Google Scholar 

  11. Marczyk, A.: On the set of cycle lengths in a Hamiltonian graph with a given maximum degree. Graphs Comb. 20, 517–529 (2004)

    Article  MATH  MathSciNet  Google Scholar 

  12. Müttel, J., Rautenbach, D., Regen, F., Sasse, T.: On the cycle spectrum of cubic Hamiltonian graphs. Graphs Comb. 29, 1067–1076 (2013)

    Article  MATH  Google Scholar 

  13. Shi, R.: \(2\)-Neighborhoods and Hamiltonian conditions. J. Graph Theory 16, 267–271 (1992)

    Article  MATH  MathSciNet  Google Scholar 

  14. Trommel, H., Veldman, H.J., Verschut, A.: Pancyclicity of claw-free Hamiltonian graphs. Discret. Math. 197–198, 781–789 (1999)

    Article  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Institut für Optimierung und Operations Research, Universität Ulm, Ulm, Germany

    Jonas Eckert, Felix Joos & Dieter Rautenbach

Authors
  1. Jonas Eckert
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. Felix Joos
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Dieter Rautenbach
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Dieter Rautenbach.

Rights and permissions

Reprints and permissions

About this article

Check for updates. Verify currency and authenticity via CrossMark

Cite this article

Eckert, J., Joos, F. & Rautenbach, D. The Cycle Spectrum of Claw-free Hamiltonian Graphs. Graphs and Combinatorics 32, 93–101 (2016). https://doi.org/10.1007/s00373-015-1530-9

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00373-015-1530-9

Keywords

Mathematics Subject Classification

Search

Navigation