Uniquely C 4-Saturated Graphs | Graphs and Combinatorics Skip to main content
Springer Nature Link
Log in

Uniquely C 4-Saturated Graphs

  • Original Paper
  • Published:
Graphs and Combinatorics Aims and scope Submit manuscript

Abstract

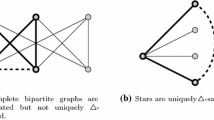
For a fixed graph H, a graph G is uniquely H-saturated if G does not contain H, but the addition of any edge from \({\overline{G}}\) to G completes exactly one copy of H. Using a combination of algebraic methods and counting arguments, we determine all the uniquely C 4-saturated graphs; there are only ten of them.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  1. Erdős P., Hajnal A., Moon J.W.: A problem in graph theory. Am. Math. Mon 71, 1107–1110 (1964)

    Article  Google Scholar 

  2. Hoffman A.J., Singleton R.R.: On Moore graphs with diameters 2 and 3. IBM J. Res. Develop. 4, 497–504 (1960)

    Article  MathSciNet  MATH  Google Scholar 

  3. Mantel W.: Problem 28, soln. by H. Gouwentak, W. Mantel, J. Teixeira de Mattes, F. Schuh and W.A. Wythoff. Wiskundige Opgaven 10, 60–61 (1907)

    Google Scholar 

  4. Ollmann, L.T.: K 2,2-saturated graphs with a minimal number of edges. Proceedings of Third SE Conference on Combinatorics, Graph Theory, and Computing, pp. 367–392. Florida Atlantic University, Boca Raton (1972)

  5. Pikhurko O.: Results and open problems on minimum saturated hypergraphs. Ars Combin. 72, 111–127 (2004)

    MathSciNet  MATH  Google Scholar 

  6. Turán P.: Eine Extremalaufgabe aus der Graphentheorie. Mat. Fiz. Lapok 48, 436–452 (1941)

    MathSciNet  MATH  Google Scholar 

  7. Wenger, P.S.: Uniquely C k -saturated graphs (in preparation)

  8. Wilf, H.S.: The friendship theorem. In: Combinatorial Mathematics and its Applications. Proceedings of International Conference, Oxford, 1969, pp. 307–309. Academic Press, London (1971)

Download references

Author information

Authors and Affiliations

  1. Mathematics Department, University of South Carolina, Columbia, SC, USA

    Joshua Cooper

  2. Mathematics Department, University of Illinois, Urbana, IL, USA

    John Lenz, Timothy D. LeSaulnier & Douglas B. West

  3. Department of Mathematical and Statistical Sciences, University of Colorado Denver, Denver, CO, USA

    Paul S. Wenger

Authors
  1. Joshua Cooper
    View author publications

    You can also search for this author in PubMed Google Scholar

  2. John Lenz
    View author publications

    You can also search for this author in PubMed Google Scholar

  3. Timothy D. LeSaulnier
    View author publications

    You can also search for this author in PubMed Google Scholar

  4. Paul S. Wenger
    View author publications

    You can also search for this author in PubMed Google Scholar

  5. Douglas B. West
    View author publications

    You can also search for this author in PubMed Google Scholar

Corresponding author

Correspondence to Paul S. Wenger.

Additional information

Research of all authors partially supported by NSF grant DMS 08-38434 “EMSW21-MCTP: Research Experience for Graduate Students.”

Douglas B. West’s Research supported by NSA grant H98230-09-1-0363.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Cooper, J., Lenz, J., LeSaulnier, T.D. et al. Uniquely C 4-Saturated Graphs. Graphs and Combinatorics 28, 189–197 (2012). https://doi.org/10.1007/s00373-011-1038-x

Download citation

  • Received:

  • Revised:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00373-011-1038-x

Keywords

Search

Navigation