Abstract
When subjected to magnetic or electric fields, nematic liquid crystals confined between two parallel glass plates and initially uniformly oriented may undergo homogeneous one-dimensional spatial distortions (Fréedericksz and Zolina, Trans. Faraday Soc. 29:919, 1933) or periodic distortions (Lonberg and Meyer, Phys. Rev. Lett. 55(7):718–721, 1985; and Srajer et al., Phys. Rev. Lett. 67(9):1102–1105, 1991). According to the experimental observations, periodic phases are stable configurations at intermediate intensity of the acting field, while homogeneous phases are stable at higher strengths.
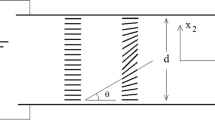
We present a fully nonlinear finite element approach able to describe homogeneous and periodic configurational phases in a cell of confined nematic liquid crystal with strong planar anchoring boundary conditions. Stationary configurations are obtained by setting to zero the first variation of the discretized total energy of the system. Unstable configurations are identified by evaluating the behavior of the solution under small numerical perturbations. Numerical calculations are able to describe the evolution of the configurational distortions as a function of the applied field and are able to capture the critical points between homogeneous and periodic phases. The proposed approach has been proved to be an excellent tool to predict the existence of unstable or metastable distortions, characterized by higher energy levels.
Similar content being viewed by others
References
Allender, D.W., Hornreich, R.M., Johnson, D.L.: Theory of the stripe phase in bend-Fréedericksz-geometry nematic films. Phys. Rev. Lett. 59, 2654–2657 (1987)
Amoddeo, A., Barbieri, R., Lombardo, G.: Electric field-induced fast nematic order dynamics liquid crystals. Liq. Cryst. 38(1), 93–103 (2011)
Barbero, G., Evangelista, L.R.: Ground states of nematic liquid crystals. Phys. Lett. A 356, 156–159 (2006)
Davis, T.A., Gartland, E.C. Jr.: Finite element analysis of the Landau–De Gennes minimization problem for liquid crystals. SIAM J. Numer. Anal. 35(1), 336–362 (1998)
de Gennes, P.G., Prost, J.: The Physics of Liquid Crystals. Clarendon Press, Oxford (1993)
Demmel, J.W., Eisenstat, S.C., Gilbert, J.R., Li, X.S., Liu, J.W.H.: A supernodal approach to sparse partial pivoting. SIAM J. Matrix Anal. Appl. 20(3), 720–755 (1999)
Deuling, H.: Deformation of nematic liquid crystals in an electric field. Mol. Cryst. Liq. Cryst. 19, 123–131 (1972)
Di Pasquale, F., Fernández, F.A., Day, S.E., Davies, J.B.: Two-dimensional finite-element modeling of nematic liquid crystal devices for optical communications and displays. IEEE J. Sel. Top. Quantum Electron. 2(1), 128–134 (1996)
Di Pasquale, F., Deng, H.F., Fernández, F.A., Day, S.E., Davies, J.B., Johnson, M.T., van der Put, A.A., van de Eerenbeemd, J.M.A., van Haaren, J.A.M.M., Chapman, J.A.: Theoretical and experimental study of nematic liquid crystal display cells using the in-plane-switching mode. IEEE Trans. Electron Devices 46(4), 661–668 (1999)
Ericksen, J.L.: Inequalities in liquid crystal theory. Phys. Fluids 9(18–19), 1205 (1966)
Fernández, F.A., Day, S.E., Trwoga, P., Deng, H.F., James, R.: Three-dimensional modelling of liquid crystal display cells using finite elements. Mol. Cryst. Liq. Cryst. 375, 291–299 (2002)
Frank, F.C.: On the theory of liquid crystals. Discuss. Trans. Faraday Soc. 25, 19–25 (1958)
Fréedericksz, V., Zolina, V.: Forces causing the orientation of an anisotropic liquid. Trans. Faraday Soc. 29, 919 (1933)
Gartland, E.C., Jr.: Structures and structural phase transitions in confined liquid crystal systems. Technical Report ICM-199511-03, Institute for Computational Mathematics, Department of Mathematics and Computer Science, Kent State University, pp. 1–17 (1995)
Golovaty, D., Gross, L.K., Hariharan, S.I., Gartland, E.C., Jr.: New ground state for the splay-Fréedericksz transition in a polymeric nematic liquid crystal. J. Math. Anal. Appl. 255, 391–403 (2001)
Gooden, C., Mahmood, R., Brisbin, D., Baldwin, A., Johnson, D.L., Neubert, M.E.: Simultaneous magnetic deformation and light-scattering study of bend and twist elastic constant divergence at the neumatic-smectic-A phase transition. Phys. Rev. Lett. 54, 1035–1038 (1985)
Gruler, H., Meier, G.: Electric field-induced deformations in oriented liquid crystals of the nematic. Mol. Cryst. Liq. Cryst. 16, 299 (1972)
James, R., Willman, E., Fernández, F.A., Day, S.E.: Finite element modeling of liquid crystal hydrodynamics with a variable degree of order. IEEE Trans. Electron Devices 53(7), 1575–1582 (2006)
Krzyzanski, D., Derfel, G.: Magnetic-field-induced periodic deformations in planar nematic layers. Phys. Rev. E 61(6), 6663–6668 (2000)
Lin, P., Liu, C.: Simulations of singularity dynamics in liquid crystal flows: a C0 finite element approach. J. Comput. Phys. 215, 348–362 (2006)
Lin, P., Liu, C., Zhang, H.: An energy law preserving C0 finite element scheme for simulating the kinematic effects in liquid crystal dynamics. J. Comput. Phys. 227, 1411–1427 (2008)
Lonberg, F., Meyer, R.B.: New ground state for the splay-Fréedericksz transition in a polymeric nematic liquid crystal. Phys. Rev. Lett. 55(7), 718–721 (1985)
Miraldi, E., Oldano, C., Strigazzi, A.: Periodic Fréedericksz transition for nematic-liquid-crystal cells with weak anchoring. Phys. Rev. A 34(5), 4348–4352 (1986)
Napoli, G.: Weak anchoring effects in elecrically driven Fréedericksz transitions. J. Phys. A, Math. Gen. 39, 11–31 (2006)
Rapini, A., Papoular, M.: Distortion d’une lamelle nématique sous champ magnétique. Conditions d’angrage aux paroix. J. Phys., Colloq. C4, 54 (1969)
Self, C.P., Please, R.H., Sluckin, T.J.: Deformation of nematic liquid crystals in an electric field. Eur. J. Appl. Math. 13, 1–23 (2002)
Srajer, G., Lonberg, F., Meyer, R.B.: Field-induced first-order phase transition and spinoidal point in nematic liquid crystals. Phys. Rev. Lett. 67(9), 1102–1105 (1991)
Virga, E.G.: Variational Theories for Liquid Crystals. Chapman & Hall, London (1994)
Zimmermann, W., Kramer, L.: Periodic splay-twist Fréedericksz transition in nematic liquid crystals. Phys. Rev. Lett. 56(24), 2655 (1986)
Author information
Authors and Affiliations
Corresponding author
Additional information
Communicated by P. Newton.
Rights and permissions
About this article
Cite this article
Pandolfi, A., Napoli, G. A Numerical Investigation on Configurational Distortions in Nematic Liquid Crystals. J Nonlinear Sci 21, 785–809 (2011). https://doi.org/10.1007/s00332-011-9100-7
Received:
Accepted:
Published:
Issue Date:
DOI: https://doi.org/10.1007/s00332-011-9100-7