Solitary Waves for Linearly Coupled Nonlinear Schrödinger Equations with Inhomogeneous Coefficients | Journal of Nonlinear Science Skip to main content
Springer Nature Link
Log in

Solitary Waves for Linearly Coupled Nonlinear Schrödinger Equations with Inhomogeneous Coefficients

  • Published:
Journal of Nonlinear Science Aims and scope Submit manuscript

Abstract

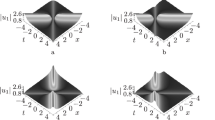
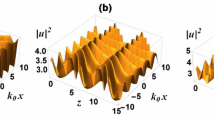
Motivated by the study of matter waves in Bose–Einstein condensates and coupled nonlinear optical systems, we study a system of two coupled nonlinear Schrödinger equations with inhomogeneous parameters, including a linear coupling. For that system, we prove the existence of two different kinds of homoclinic solutions to the origin describing solitary waves of physical relevance. We use a Krasnoselskii fixed point theorem together with a suitable compactness criterion.

This is a preview of subscription content, log in via an institution to check access.

Access this article

Log in via an institution

Subscribe and save

Springer+ Basic
¥17,985 /Month
  • Get 10 units per month
  • Download Article/Chapter or eBook
  • 1 Unit = 1 Article or 1 Chapter
  • Cancel anytime
Subscribe now

Buy Now

Price includes VAT (Japan)

Instant access to the full article PDF.

Institutional subscriptions

Similar content being viewed by others

References

  • Abdullaev, F.K., Garnier, J.: Propagation of matter-wave solitons in periodic and random nonlinear potentials. Phys. Rev. A 72, 061605 (2005)

    Article  MathSciNet  Google Scholar 

  • Ambrosetti, A., Colorado, E.: Bound and ground states of coupled nonlinear Schrödinger equations. C. R. Acad. Sci. Paris, Ser. I 342, 453–458 (2006)

    MATH  MathSciNet  Google Scholar 

  • Ambrosetti, A., Colorado, E.: Standing waves of some coupled Nonlinear Schrödinger equations. J. Lond. Math. Soc. 75, 67–82 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  • Ambrosetti, A., Colorado, E., Ruiz, D.: Multi-bump solitons to linearly coupled systems of nonlinear Schrödinger equation. Calc. Var. Partial Differ. Equ. 30, 85–112 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  • Ambrosetti, A., Cerami, G., Ruiz, D.: Solitons of linearly coupled systems of semilinear non-autonomous equation on ℝN. J. Funct. Anal. (2008). doi:10.1016/j.jfa.2007.11.013

    MathSciNet  Google Scholar 

  • Belmonte-Beitia, J., Pérez-García, V.M., Vekslerchik, V., Torres, P.J.: Lie symmetries and solitons in nonlinear systems with spatially inhomogeneous nonlinearities. Phys. Rev. Lett. 98, 064102 (2007)

    Article  Google Scholar 

  • Belmonte-Beitia, J., Pérez-García, V.M., Vekslerchik, V., Torres, P.J.: Lie symmetries, qualitative analysis and exact solutions of nonlinear Schrödinger equations with inhomogeneous nonlinearities. Discrete Contin. Dyn. Syst. B 9, 221 (2008)

    MATH  Google Scholar 

  • Brazhnyi, V.A., Konotop, V.V.: Stable and unstable vector dark solitons of coupled nonlinear Schrödinger equations: Application to two-component Bose–Einstein condensates. Phys. Rev. E 72, 026616 (2005)

    Article  MathSciNet  Google Scholar 

  • Brezzi, F., Markowich, P.A.: The three-dimensional Wigner–Poisson problem: existence, uniqueness and approximation. Math. Mod. Meth. Appl. Sci. 14, 35 (1991)

    Article  MATH  MathSciNet  Google Scholar 

  • Catani, J., De Sarlo, L., Barontini, G., Minardi, F., Inguscio, M.: Degenerate Bose–Bose mixture in a three-dimensional optical lattice. Phys. Rev. A 77, 011603 (2008)

    Article  Google Scholar 

  • Chu, J., O’Regan, D., Zhang, M.: Positive solutions and eigenvalue intervals for nonlinear systems. Proc. Indian Acad. Sci. Math. Sci. 117, 85–95 (2007)

    Article  MATH  MathSciNet  Google Scholar 

  • Dalfovo, F., Giorgini, S., Pitaevskii, L.P., Stringari, S.: Theory of Bose–Einstein condensation in trapped gases. Rev. Mod. Phys. 71, 463–512 (1999)

    Article  Google Scholar 

  • Davydov, A.S.: Solitons in Molecular Systems. Reidel, Dordrecht (1985)

    MATH  Google Scholar 

  • Deconinck, B., Kevrekidis, P.G., Nistazakis, H.E., Frantzeskakis, D.J.: Linearly coupled Bose–Einstein condensates: From Rabi oscillations and quasiperiodic solutions to oscillating domain walls and spiral waves. Phys. Rev. A 70, 063605 (2004)

    Article  Google Scholar 

  • Dodd, R.K., Eilbeck, J.C., Gibbon, J.D., Morris, H.C.: Solitons and Nonlinear Wave Equations. Academic Press, San Diego (1982)

    MATH  Google Scholar 

  • Dong, G., Hu, B.: Management of Bose–Einstein condensates by a spatially periodic modulation of the atomic s-wave scattering length. Phys. Rev. A. 75, 013625 (2007)

    Article  Google Scholar 

  • Erdős, L., Schlein, B., Yau, H.-T.: Derivation of the Gross–Pitaevskii equation for the dynamics of Bose–Einstein condensate. Preprint (2006). arXiv:math-ph/0606017

  • Erdős, L., Schlein, B., Yau, H.-T.: Derivation of the cubic non-linear Schrödinger equation from quantum dynamics of many-body systems. Invent. Math. 167, 515–614 (2007a)

    Article  MathSciNet  Google Scholar 

  • Erdős, L., Schlein, B., Yau, H.-T.: Rigorous derivation of the Gross–Pitaevskii equation. Phys. Rev. Lett. 98, 040404 (2007b)

    Article  Google Scholar 

  • Fedele, R., Miele, G., Palumbo, L., Vaccaro, V.G.: Thermal wave model for nonlinear longitudinal dynamics in particle accelerators. Phys. Lett. A 173, 407–413 (1993)

    Article  Google Scholar 

  • García-Ripoll, J.J., Cirac, J.I., Anglin, J., Pérez-García, V.M., Zoller, P.: Spin monopoles with Bose–Einstein condensates. Phys. Rev. A 61, 053609 (2000)

    Article  Google Scholar 

  • García-Ripoll, J.J., Pérez-García, V.M., Sols, F.: Split vortices in optically coupled Bose–Einstein condensates. Phys. Rev. A 66, 021602(R) (2002)

    Article  Google Scholar 

  • Garnier, J., Abdullaev, F.K.: Transmission of matter-wave solitons through nonlinear traps and barriers. Phys. Rev. A 74, 013604 (2006)

    Article  Google Scholar 

  • Granas, A., Dugundji, J.: Fixed Point Theory. Springer, Berlin (2003)

    MATH  Google Scholar 

  • Hasegawa, A.: Optical Solitons in Fibers. Springer, Berlin (1989)

    Google Scholar 

  • Jiang, D., Wei, J., Zhang, B.: Positive periodic solutions of functional differential equations and population models. Electr. J. Differ. Equ. 71, 1–13 (2002)

    MathSciNet  Google Scholar 

  • Kasamatsu, K., Tsubota, M.: Modulation instability and solitary-wave formation in two-component Bose–Einstein condensates. Phys. Rev. A 74, 013617 (2006)

    Article  Google Scholar 

  • Kivshar, Y., Agrawal, G.P.: Optical Solitons: From Fibers to Photonic Crystals. Academic Press, San Diego (2003)

    Google Scholar 

  • Krasnoselskii, M.A.: Positive Solutions of Operator Equations. Noordhoff, Groningen (1964)

    Google Scholar 

  • Li, H., Wang, D.N.: Control for dynamics of two coupled Bose–Einstein condensate solitons by potential deviation. Chaos Solitons Fractals 36, 1377–1384 (2008)

    Article  MATH  Google Scholar 

  • Lieb, E.H., Seiringer, R.: Proof of Bose–Einstein condensation for dilute trapped gases. Phys. Rev. Lett. 88, 170409 (2002)

    Article  Google Scholar 

  • Lieb, E.H., Seiringer, R., Yngvason, J.: Bosons in a trap: A rigorous derivation of the Gross–Pitaevskii energy functional. Phys. Rev. A 61, 043602 (2000)

    Article  Google Scholar 

  • López, J.L., Soler, J.: Asymptotic behaviour to the 3D Schrödinger/Hartree–Poisson and Wigner–Poisson systems. Math. Mod. Meth. Appl. Sci. 10, 923–943 (2000)

    MATH  Google Scholar 

  • Maddaloni, P., Modugno, M., Fort, C., Minardi, F., Inguscio, M.: Collective oscillations of two colliding Bose–Einstein condensates. Phys. Rev. Lett. 85, 2413 (2000)

    Article  Google Scholar 

  • Matthews, M.R., Anderson, B.P., Haljan, P.C., Hall, D.S., Wieman, C.E., Cornell, E.A.: Vortices in a Bose–Einstein condensate. Phys. Rev. Lett. 83, 2498–2501 (1999a)

    Article  Google Scholar 

  • Matthews, M.R., Anderson, B.P., Haljan, P.C., Hall, D.S., Holland, M.J., Williams, J.E., Wieman, C.E., Cornell, E.A.: Watching a superfluid untwist itself: Recurrence of Rabi oscillations in a Bose–Einstein condensate. Phys. Rev. Lett. 83, 3358 (1999b)

    Article  Google Scholar 

  • Merhasin, I.M., Malomed, B.A., Driben, R.: Transition to miscibility in a binary Bose–Einstein condensate induced by linear coupling. J. Phys. B: At. Mol. Opt. Phys. 38, 877–892 (2005)

    Article  Google Scholar 

  • Minardi, F., Fort, C., Maddaloni, P., Modugno, M., Inguscio, M.: Time-domain atom interferometry across the threshold for Bose–Einstein condensation. Phys. Rev. Lett. 87, 170401 (2001)

    Article  Google Scholar 

  • Modugno, G., Modugno, M., Riboli, F., Roati, G., Inguscio, M.: Two atomic species superfluid. Phys. Rev. Lett. 89, 190404 (2002)

    Article  Google Scholar 

  • Nakamura, K., Kohi, A., Yamasaki, H., Pérez-García, V.M., Konotop, V.V.: Levitation of spinor Bose–Einstein condensates: Macroscopic manifestation of the Franck–Condon effect. Europhys. Lett. 80, 50005 (2007)

    Article  Google Scholar 

  • Niarchou, P., Theocharis, G., Kevrekidis, P.G., Schmelcher, P., Frantzeskakis, D.J.: Soliton oscillations in collisionally inhomogeneous attractive Bose–Einstein condensates. Phys. Rev. A 76, 023615 (2007)

    Article  Google Scholar 

  • Pérez-García, V.M., Michinel, H., Herrero, H.: Bose–Einstein solitons in highly asymmetric traps. Phys. Rev. A 57, 3837 (1998)

    Article  Google Scholar 

  • Porter, M.A., Kevrekidis, P.G., Malomed, B.A., Frantzeskakis, D.J.: Modulated amplitude waves in collisionally inhomogenous Bose–Einstein condensates. Preprint. nlin.PS/0607009

  • Primatarowa, M.T., Stoychev, K.T., Kamburova, R.S.: Interactions of solitons with extended nonlinear defects. Phys. Rev. E 72, 036608 (2005)

    Article  Google Scholar 

  • Raghavan, S., Smerzi, A., Fantoni, S., Shenoy, S.R.: Coherent oscillations between two weakly coupled Bose–Einstein condensates: Josephson effects, π oscillations, and macroscopic quantum self-trapping. Phys. Rev. A 59, 620–633 (1999)

    Article  Google Scholar 

  • Rodas-Verde, M.I., Michinel, H., Pérez-García, V.M.: Controllable soliton emission from a Bose–Einstein condensate. Phys. Rev. Lett. 95(15), 153903 (2005)

    Article  Google Scholar 

  • Rosales, J.L., Sánchez-Gómez, J.L.: Nonlinear Schödinger equation coming from the action of the particles gravitational field on the quantum potential. Phys. Lett. A 66, 111–115 (1992)

    Article  Google Scholar 

  • Saito, H., Hulet, R.G., Ueda, M.: Stabilization of a Bose–Einstein droplet by hyperfine Rabi oscillations. Phys. Rev. A 76, 053619 (2007)

    Article  Google Scholar 

  • Sakaguchi, H., Malomed, B.: Two-dimensional solitons in the Gross–Pitaevskii equation with spatially modulated nonlinearity. Phys. Rev. E 73, 026601 (2006)

    Article  MathSciNet  Google Scholar 

  • Scott, A.: Nonlinear Science: Emergence and Dynamics of Coherent Structures. Oxford Appl. and Eng. Mathematics, vol. 1. Oxford University Press, Oxford (1999)

    MATH  Google Scholar 

  • Stuart, C.A.: Guidance properties of nonlinear planar waveguides. Arch. Ration. Mech. Anal. 125, 145–200 (1993)

    Article  MATH  MathSciNet  Google Scholar 

  • Sulem, C., Sulem, P.: The Nonlinear Schrödinger Equation: Self-focusing and Wave Collapse. Springer, Berlin (2000)

    Google Scholar 

  • Teocharis, G., Schmelcher, P., Kevrekidis, P.G., Frantzeskakis, D.J.: Matter-wave solitons of collisionally inhomogeneous condensates. Phys. Rev. A 72, 033614 (2005)

    Article  Google Scholar 

  • Theis, M., Thalhammer, G., Winkler, K., Hellwig, M., Ruff, G., Grimm, R., Hecker Denschlag, J.: Tuning the scattering length with an optically induced feshbach resonance. Phys. Rev. Lett. 93, 123001 (2004)

    Article  Google Scholar 

  • Torres, P.J.: Guided waves in a multi-layered optical structure. Nonlinearity 19, 2103–2113 (2006)

    Article  MATH  MathSciNet  Google Scholar 

  • Vázquez, L., Streit, L., Pérez-García, V.M. (Eds.): Nonlinear Klein–Gordon and Schrödinger systems: Theory and Applications. World Scientific, Singapore (1997)

    Google Scholar 

  • Vázquez-Carpentier, A., Michinel, H., Rodas-Verde, M.I., Pérez-García, V.M.: Analysis of an atom soliton laser based on the spatial control of the scattering length. Phys. Rev. A 74, 053610 (2006)

    Article  Google Scholar 

  • Williams, J., Walser, R., Cooper, J., Cornell, E.A., Holland, M.: Excitation of a dipole topological state in a strongly coupled two-component Bose–Einstein condensate. Phys. Rev. A 61, 033612 (2000)

    Article  Google Scholar 

  • Zafrany, A., Malomed, B.A., Merhasin, I.M.: Solitons in a linearly coupled system with separated dispersion and nonlinearity. Chaos 15, 037108 (2005)

    Article  MathSciNet  Google Scholar 

  • Zima, M.: On positive solutions of boundary value problems on the half-line. J. Math. Anal. Appl. 259, 127–136 (2001)

    Article  MATH  MathSciNet  Google Scholar 

Download references

Author information

Authors and Affiliations

  1. Departamento de Matemáticas, E.T.S. de Ingenieros Industriales and Instituto de Matemática Aplicada a la Ciencia y la Ingeniería (IMACI), Universidad de Castilla-La Mancha, 13071, Ciudad Real, Spain

    Juan Belmonte-Beitia & Víctor M. Pérez-García

  2. Departamento de Matemática Aplicada, Facultad de Ciencias, Universidad de Granada, Campus de Fuentenueva s/n, 18071, Granada, Spain

    Pedro J. Torres

Authors
  1. Juan Belmonte-Beitia
    View author publications

    You can also search for this author inPubMed Google Scholar

  2. Víctor M. Pérez-García
    View author publications

    You can also search for this author inPubMed Google Scholar

  3. Pedro J. Torres
    View author publications

    You can also search for this author inPubMed Google Scholar

Corresponding author

Correspondence to Juan Belmonte-Beitia.

Additional information

Communicated by B. Eckhardt.

Rights and permissions

Reprints and permissions

About this article

Cite this article

Belmonte-Beitia, J., Pérez-García, V.M. & Torres, P.J. Solitary Waves for Linearly Coupled Nonlinear Schrödinger Equations with Inhomogeneous Coefficients. J Nonlinear Sci 19, 437–451 (2009). https://doi.org/10.1007/s00332-008-9037-7

Download citation

  • Received:

  • Accepted:

  • Published:

  • Issue Date:

  • DOI: https://doi.org/10.1007/s00332-008-9037-7

Keywords

Mathematics Subject Classification (2000)